User talk:Wcherowi/Archive 3
| This is an archive of past discussions about User:Wcherowi. Do not edit the contents of this page. If you wish to start a new discussion or revive an old one, please do so on the current talk page. |
| Archive 1 | Archive 2 | Archive 3 | Archive 4 | Archive 5 | Archive 6 |
ArbCom Elections 2016: Voting now open!
Hello, Wcherowi. Voting in the 2016 Arbitration Committee elections is open from Monday, 00:00, 21 November through Sunday, 23:59, 4 December to all unblocked users who have registered an account before Wednesday, 00:00, 28 October 2016 and have made at least 150 mainspace edits before Sunday, 00:00, 1 November 2016.
The Arbitration Committee is the panel of editors responsible for conducting the Wikipedia arbitration process. It has the authority to impose binding solutions to disputes between editors, primarily for serious conduct disputes the community has been unable to resolve. This includes the authority to impose site bans, topic bans, editing restrictions, and other measures needed to maintain our editing environment. The arbitration policy describes the Committee's roles and responsibilities in greater detail.
If you wish to participate in the 2016 election, please review the candidates' statements and submit your choices on the voting page. MediaWiki message delivery (talk) 22:08, 21 November 2016 (UTC)
Please review that page and tell others (such as high school and college math students and faculty) about it.--2602:304:CDC1:90:64C5:4976:D62C:FF33 (talk) 10:32, 25 November 2016 (UTC)
About my edit that you reverted on Methods of computing square roots
About the proof for the algorithm, I didn't make the proof up; I received it from Adam E. Parker, who is Assistant Professor of Mathematics at the University of Wittenberg, through a third party. I'd upload the PDF with the proof, but I don't have a Wikipedia account, so I can't upload files, so I'm kind of stuck. I don't want this to turn into an edit war with reversions every week or so, but I feel that if Wikipedia requires someone to have an account in order to be able to get a mathematical statement with a mathematical proof from a reliable source that is in the publishing process to be "noteworthy and reliable enough" to be on one of Wikipedia's pages, it sort of defeats the whole point of even letting people who don't have Wikipedia accounts from editing Wikipedia at all because an account is required to get something that meets all of Wikipedia's criteria on its page. It's like saying, "Here, you may create whatever songs you want in the music room and write them on staff paper, but we'll erase all of it within a day." Is that really what Wikipedia stands for?2601:2C1:C301:6350:383D:4226:1A53:8B3B (talk) 02:19, 28 November 2016 (UTC)
- Two points. First of all, results put on Wikipedia should appear in reliable secondary sources so even a pdf of the proof provided by the author would not satisfy this requirement. We are not in the business of verifying the correctness nor determining the notability of results, the scholarly community does this and we merely report on what they say. The second issue of not having an account is a bit of a red herring. Anyone can create an account for themselves and in the rare event that this is not possible, one can ask someone to create an account for them. See WP:ACCOUNT. --Bill Cherowitzo (talk) 04:31, 28 November 2016 (UTC)
- Is there a list of contested math facts for scholarly review that I can put this on? If so, what's the link to it? 50.235.102.246 (talk) 14:59, 28 November 2016 (UTC)
- It doesn't quite work that way. First of all the result has to be published in a reliable journal (reliable in mathematics means peer reviewed). This is the mathematical community standard for judging correctness (not an infallible system, clearly errors get through, but major flaws are generally caught). Then, someone else needs to mention the result by using it or discussing it in their own work. This second step is what establishes notability. Wikipedia reports on what these secondary sources say. This process takes time, so new results are not generally found on Wikipedia. We are not a bulletin board and there are plenty of other venues available for "getting the word out" about some new results. --Bill Cherowitzo (talk) 18:31, 28 November 2016 (UTC)
Nontransitive dice
Hi, I would just like to let you know that following our dispute over grammar in the edit section, I have made a Request for Comment on the nontransitive dice talk page. Skewb? (talk) 11:12, 31 December 2016 (UTC)
Permutation Matrix
Hi Please write here a proof that shows that the order of composition is correct as stated in the wiki page. I tried writing such proofs and the order is simply wrong. — Preceding unsigned comment added by 79.178.242.43 (talk) 18:56, 16 January 2017 (UTC)
- Please read the page more carefully. Both orders are correct and are given on the page. The difference depends on whether the matrices act on column vectors on the right, or row vectors on the left. The two formulas correspond to these two choices. If you get them mixed up, you end up with nonsense. --Bill Cherowitzo (talk) 04:43, 17 January 2017 (UTC)
- Please provide a proof to : (Using the definition of the preceding section). Note that this isn't consistent with : since by composing twice you get the wrong composition: Please explain this and provide a proof.
- The problem is that your last step is incorrect, it should be,
- that is to say, first then . It is possible that you are switching from a right action to a left action when you write the composition of the permutations (that is the only way I can make sense out of what you wrote). I know that this can get very annoying. I learned my permutation actions one way, but I have often had to teach them using the other convention. This makes me keenly aware of the problems that arise when you subconsciously switch between the two.--Bill Cherowitzo (talk) 19:48, 17 January 2017 (UTC)
- But if we denote then we get How can you explain this?
- that is to say, first then . It is possible that you are switching from a right action to a left action when you write the composition of the permutations (that is the only way I can make sense out of what you wrote). I know that this can get very annoying. I learned my permutation actions one way, but I have often had to teach them using the other convention. This makes me keenly aware of the problems that arise when you subconsciously switch between the two.--Bill Cherowitzo (talk) 19:48, 17 January 2017 (UTC)
- You can ignore what I said before (and I probably got my lefts and rights mixed up anyway, I usually do). You are correct in that this property does not follow from the given definition in the article, and the reason is that the definition (actually, the statement that is supposed to be equivalent to it) is wrong! I will shortly fix the page, but let me expand on this here. Given a permutation and the standard basis (row) vectors , the permutation matrix associated to is (correctly given in the article)
- That is, the rows of the identity matrix are permuted according to . This means that in the column of the permutation matrix a 1 will appear in row (this is where the error is in the article - it gets the row and column mixed up). Now consider multiplying (on the right) by the standard basis (column) vector that I will still call . It is fairly easy to see that since you only need to know which row of the permutation matrix contains a 1 in the column. Composition of two permutation matrices on these basis vectors then follows immediately: . Since this is valid for all basis vectors, it holds for all vectors and we have I think that I will add an example to the page in order to get this clearer.--Bill Cherowitzo (talk) 04:25, 18 January 2017 (UTC)
- Shouldn't it be
- ? — Preceding unsigned comment added by 79.178.238.30 (talk) 05:11, 18 January 2017 (UTC)
- Yep, you are right. I've been checking various sources to see what is going on here, and the literature is in a mess. There are left - right, row - column ambiguities galore.
To get the formula to work (and I have found a source that has this) the permutation matrix needs to be defined by permuting the columns of the identity matrix, so we need to have with the being column vectors. Now the statement I made before, namely "in the column of the permutation matrix a 1 will appear in row " actually holds, and the rest of the argument follows. Your original concern was correct, with the definition given that formula doesn't work. I knew that the formula was correct, having seen it several times, but I didn't pay enough attention to the assumptions that needed to be made to get there. I am supposing that if permuting the rows of the identity is used to define a permutation matrix, then having the matrix act on the right of row vectors (I think that's correct terminology) should give the same composition formula ... but I need to go through the details before I'll commit to that statement. This will have to wait until tomorrow.--Bill Cherowitzo (talk) 07:46, 18 January 2017 (UTC)- Thanks for your patience. — Preceding unsigned comment added by 79.178.238.30 (talk) 22:22, 18 January 2017 (UTC)
- I must not have had enough sleep yesterday, I made so many mistakes in the above. The article is ok as it stands, except for the formula for composition. Here is the proof giving the right formula for this definition. With the being the standard row basis vectors, the permutation matrix corresponding to permutation is,
- This matrix can be obtained by permuting the COLUMNS of the identity matrix according to , i.e., column i is permuted to the position. Thus, in row i of the 1 appears in column . Alternatively said, the 1 in column k of occurs in row . Now consider the product with the standard basis column vector, . The result only has a 1 in the position corresponding to the row of that has a 1 in column k, that is, . From this it follows that for two permutation matrices we have
- Since this holds for all basis vectors, it is true for all column vectors and we have
- .
- Thanks for your patience. — Preceding unsigned comment added by 79.178.238.30 (talk) 22:22, 18 January 2017 (UTC)
- Yep, you are right. I've been checking various sources to see what is going on here, and the literature is in a mess. There are left - right, row - column ambiguities galore.
I'll fix this and also put in a numerical example tonight.--Bill Cherowitzo (talk) 22:58, 18 January 2017 (UTC)
Hello Wcherowi,
is "de:Mengendiagramm" or any other German interwikilink actually visible in your view of the page? It isn't in mine. Also the referenced Wikidata page cannot list it since it is already used to link to the more general page on Euler and Venn diagrams.
best regards, KaiKemmann (talk) 16:07, 30 January 2017 (UTC)
- Hi, the link, under Languages Deutsch, appears in the left-hand panel of the Euler diagram page. The placement may depend on the skin you are using; I'm using MonoBook. I did notice that there is no corresponding link on the Venn diagram page and this makes me think that there might be a problem involving some administrative decision about interlinks (there was a problem involving French interlinks not so long ago which seems to have a similar feel to it). If this is a problem with Wikidata, then I am out of my league ... the solution might be to add this link to the Venn diagram page. I hope this helps. --Bill Cherowitzo (talk) 17:10, 30 January 2017 (UTC)
- I just realized that I had reverted just this change ... my apologies, I'll undo that in a moment.--Bill Cherowitzo (talk) 17:34, 30 January 2017 (UTC)
- Thanks. The problem is in my view that Wikidata only allows for exactly one interwikilink per article and language. So when more articles on a certain subject matter exist in one language than in the other or the content is structured differently into articles in the various language wikis, this cannot be correctly mapped on Wikidata. best regards, KaiKemmann (talk) 14:48, 1 February 2017 (UTC)
- Yes, this is the same issue that I alluded to above, the French have one article while we have two: Fibonacci number and Fibonacci series, if I am not mistaken.--Bill Cherowitzo (talk) 19:59, 1 February 2017 (UTC)
Notation in the proof of bijectivity in Cantor's diagonal argument
You stated that indication of base is unnecessary, because the discourse has already established that we are in base 2. I think that's a fair point, but I respectfully disagree that the notation g(t)=0.t_{(2)} is superfluous. Because t is used as a sequence on the LHS (essentially an element of R^\infty), but as a radix notation quantity on the RHS (where it equals \sum_k a_k2^{-k}), it helps the reader to be reminded of that fact.
(It's really an egregious abuse of notation, but for the sake of simplicity, I support the current usage, provided that the base is explicitly indicated.)
Obviously, some people felt very strongly about how this section should be written, despite its numerous problems in English language and style, so I won't begrudge a few subscripts if you insist on removing them.
Cheers, -Jimmy Alsosaid1987 (talk) 06:57, 14 February 2017 (UTC)
- I really do understand what you are trying to do and I am not claiming that it is in any way improper, but I don't think that it is providing the clarity for the reader that you seem to think it does. Part of writing good mathematics is being able to anticipate the level of your audience; missing that mark by too much, in either direction, causes problems. Sometimes, for the sake of getting this right, I have to ask myself, "can I say in words what I would naturally want to include in notation?" and the answer to this question, when it arises here in Wikipedia, is almost invariably "yes, and I should do so." Note that I do not believe in dumbing down the discourse to reach my target audience, I'm just being careful about not packing too much into the symbolism that would otherwise be my natural way of communicating with mathematical professionals. --Bill Cherowitzo (talk) 17:20, 14 February 2017 (UTC)
Pascal's triangle
Hi, I wonder why you reversed the edits on Pascal's triangle? The country calls Iran and people are Iranian. I know before 1936 in western world used word Persia to reference the entire country, the countries authorities requested all foreign nations to call the country Iran and its residents Iranians (what they always used to call themselves). And this is officially accepted. So if you feel this is not convincing please let me know what is the rationales behind your reversion otherwise please undo your reversion.--F4fluids (talk) 19:33, 24 February 2017 (UTC)
- Editors do not have the flexibility to make the changes you wish to make since we are bound to use the terminology that is present in the sources that we use, whether or not this is currently politically correct. When alternatives exist, we are to use the most common expressions. The vast majority of English language sources use the terms Persia and Persian in this historical context and to change that would mean that we were not following the sources. If someday the majority of sources use the terms Iran and Iranian to refer to these historical places and people, then we would be obliged to follow that terminology, but this is not the case today.--Bill Cherowitzo (talk) 00:04, 25 February 2017 (UTC)
- Hi Bill. I still think this is wrong as the country and its residents wrongly used to be called Persia (which is a province even in current day Iran which is called Fars province) and Persians through a long history. And the two people who are referenced in the article are not from that province. But I understand the policy and if it doesn't give a way to correct this, then I won't protest against it. Thanks for response though.--F4fluids (talk) 06:53, 25 February 2017 (UTC)
Thanks.
Thanks for you edits at Determinant. Isambard Kingdom (talk) 17:43, 13 March 2017 (UTC)
- Actually, I was being lazy and your edit forced me to do the right thing. I had noticed that when you first reverted the IP's change that you hadn't gone back far enough. After thinking about it for a while I realized that the IP had gotten confused by the fact that there seemed to be too many indices, so I tried to fix that up in a rather minimalist way. However, you are right, both versions needed to be explicitly given to make this absolutely clear. So, in my book, I should be thanking you. --Bill Cherowitzo (talk) 20:50, 13 March 2017 (UTC)
Hyperbola
Hi Wcherowi,
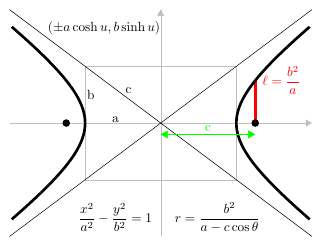
Anita5192 and I are seeing something different per the following exchange regarding the last bullet near the bottom of the article; can you understand what she just said to me in Imagine taking the triangle..., and flipping it over. ....? I'm an engineer so I must be missing something subtle.
Anita, Thanks for your hawk eyes; I am the 68.98.184.101 and wonder if the following needs fixing; "The distance from either focus to either asymptote is b, the semi-minor axis;..." should IMO per all previous discussion have 'focus' replaced by 'vertex' at minimum, and might be clearer by saying "The distance from either vertex to either of its asymptotes is b,...."? Jedwin 68.98.184.101 (talk) 22:23, 12 March 2017 (UTC) I just changed one line to read correctly, "The distance b (not shown) is the length of the perpendicular segment from either focus to the asymptotes." It is unfortunate that the diagram does not show b or θ. The line which reads, "The distance from either focus to either asymptote is b . . ." is already correct.—Anita5192 (talk) 23:34, 12 March 2017 (UTC) Anita, A*A + B*B == C*C cannot exist if the focus is used because it is not a right triangle; and also, C is already the distance from the center to the focus that is actually A*(E-1) beyond A. — Preceding unsigned comment added by 68.98.184.101 (talk) 01:23, 14 March 2017 (UTC) b is the distance from a focus to an asymptote, i.e., the perpendicular distance, hence the triangle is a right triangle. Imagine taking the triangle with base from C to the vertex and a right angle at the vertex, and flipping it over. If you do the math, you will see that it is correct.—Anita5192 (talk) 01:41, 14 March 2017 (UTC)
THANKS, JEDWIN at 68.98.184.101 68.98.184.101 (talk) 02:19, 14 March 2017 (UTC)
Hi Jedwin, I'll do my best. Anita5192 is correct about the edit, but her last bit of explanation to you was missing a vital piece of information. Here is the diagram that I believe you are thinking of:
Unfortunately, this isn't labelled so the explanation will be a bit long winded. The line segment from a vertex, drawn perpendicular to the major axis and ending at an asymptote has length b. I think that this is what you are thinking of when you want to replace focus with vertex. However, b is not the distance from the vertex to the asymptote because that distance is measured along the line drawn from the vertex that is perpendicular to the asymptote. If that distance was also b you would be in the awkward position of having a right triangle whose hypotenuse was the same length as one of its legs. (If you don't see it, label a vertex V, the end of a vertical line through V on the asymptote Q and the foot of the perpendicular dropped from V to the asymptote P. VPQ is the right triangle with right angle at P. VQ is the hypotenuse with length b and VP is the leg with length b.) Now, when you drop a perpendicular from a focus (say F) to an asymptote, its length will also be b. To see this, consider the right triangle VQO, where O is the origin (I think Anita labelled this point C), the right angle here is at V. Note that Q is at distance c from O (see diagram). Rotate the asymptote that Q is on so that it lies on the major axis and Q coincides with F. Now flip the right triangle VQO over its hypotenuse (OF) and V turns into the foot of the perpendicular drawn from F to the asymptote (in its original position). Since the rotation and flip are rigid motions, the length of that segment is still b. I hope this helps.
- It's not my first way of thinking about these things, but perhaps this would be clearer if I did it with coordinates. Let V(a,0) be the vertex, F(c,0) a focus. The line from F that is perpendicular to the asymptote with equation y = b/ax has equation y = −a/bx + ac/b. So, the point of intersection P has coordinates (a2/c, ab/c). Now the distance from P to F can be calculated to be b and the distance from P to O is calculated to be a (with heavy use of the relation a2 + b2 = c2.) --Bill Cherowitzo (talk) 16:32, 14 March 2017 (UTC)
Editor of the Week

|
Editor of the Week | |
| Your ongoing efforts to improve the encyclopedia have not gone unnoticed: You have been selected as Editor of the Week for your work on math articles. Thank you for the great contributions! (courtesy of the Wikipedia Editor Retention Project) |
User:Buster7 submitted the following nomination for Editor of the Week:
- Editor Wcherowi is a Mathematician. One of the difficulties in writing Math articles is providing hard-to-grasp information with clarity. As he says, "Writing good mathematics is being able to anticipate the level of your audience; missing that mark by too much, in either direction, causes problems". Having a concern for the wide ranging capacity of our reader is vital to the health of WikiPedia. Wcherowi has been most active since 2014, and spends 85% of his edits to writing and improving math article such as Non-Euclidean geometry, Projective Geometry, Conic section, Oval (projective plane), History of Mathematics, and many, many more. He further states:"I do not believe in dumbing down the discourse to reach my target audience, I'm just being careful about not packing too much into the symbolism that would otherwise be my natural way of communicating with mathematical professionals".
You can copy the following text to your user page to display a user box proclaiming your selection as Editor of the Week:
{{Wikipedia:WikiProject Editor Retention/Editor of the Week/Recipient user box}}
Thanks again for your efforts! Lepricavark (talk) 19:18, 18 March 2017 (UTC)
- Congratulations, Wcherowi! Thank you so much for your contributions!
 Mz7 (talk) 17:41, 19 March 2017 (UTC)
Mz7 (talk) 17:41, 19 March 2017 (UTC)
- WOW, I did not see this one coming. I am honored and deeply touched and want to thank everyone involved (especially the nominator and seconder, naturally
 ). I can think of maybe a dozen active math editors who are probably more worthy of this than I am–but heh, I'm going to enjoy it while I can. Just one comment for the Editor Retention WikiProject, I've been a very active editor for a couple of years now, so how come I've never heard about this Editor of the Week program? Perhaps it is because I don't stick my nose outside of the math pages very much, but then again there are probably many worthy editors who specialize in this way ... just saying. Thanks again. --Bill Cherowitzo (talk) 19:31, 20 March 2017 (UTC)
). I can think of maybe a dozen active math editors who are probably more worthy of this than I am–but heh, I'm going to enjoy it while I can. Just one comment for the Editor Retention WikiProject, I've been a very active editor for a couple of years now, so how come I've never heard about this Editor of the Week program? Perhaps it is because I don't stick my nose outside of the math pages very much, but then again there are probably many worthy editors who specialize in this way ... just saying. Thanks again. --Bill Cherowitzo (talk) 19:31, 20 March 2017 (UTC)
- WOW, I did not see this one coming. I am honored and deeply touched and want to thank everyone involved (especially the nominator and seconder, naturally
Poset
Hi Wcherowi, could you explain this reversion? https://en.wikipedia.org/w/index.php?title=Partially_ordered_set&oldid=prev&diff=771120063
What are you saying is not consistent with general terminology? The word "precedes"? That word is used in the same sense in the lede, which is why I chose it. I think it's more likely to cause confusion to say "a is related to b" to mean "a ≤ b", since in ordinary English, "is related to" is symmetric. To the non-mathematician, I think "a is related to b" immediately implies that b is also related to a. CodeTalker (talk) 18:17, 19 March 2017 (UTC)
- When discussing properties of general binary relations the term "is related to" is the most common locution and a reader who linked to Binary relation in search of some more background material would be facing such terminology. "Precedes" is the term typically used for the usual order relation of the reals (or other number systems) and since partial orders are a generalization of that relation it is sometimes okay to extend that usage to more general partial orders. However, in the context of the other common partial orders, subset inclusion and integer divisibility, this language sounds very artificial and does not promote understanding. You have a point, although I don't completely agree, that "is related to" has a symmetric connotation in English, but then again many mathematical terms have exactly that problem–carrying additional connotations in the embedding language–would you be wanting to avoid all of them as well? I don't think that the way to deal with this problem is to avoid it but rather to use the opportunity to clarify the differences between technical and non-technical usages of words.--Bill Cherowitzo (talk) 21:27, 19 March 2017 (UTC)
- Do you also object to the word "precedes" in the lede? It seems to me that the same problem exists there, since the lede is talking about general partial orders, not just numeric ordering. Why is it ok there?
- I agree that technical terms in math and science sometimes have specialized meanings that differ from their common usage, and it's not possible to avoid them all. However, it's hard for me to believe that there isn't a better term than "is related to" that we could use, that is mathematically acceptable but also not so starkly symmetric in everyday use. I took a quick look through my copy of Kaplansky's Set Theory and Metric Spaces where partial ordering is discussed, and it seems he avoids the whole problem by using the symbol ≤ rather than any English word. It seems to me that the point of using English sentences in addition to the symbolic statements in the Formal definition section of our article is to give some help to readers who aren't completely comfortable understanding the statements when written purely symbolically. Such readers are exactly the ones who are likely to be confused by the technical use of "is related to" in a non-symmetric sense. It seems a little odd to add an English restatement of a symbolic statement, but phrase it in a way that is potentially confusing to readers who don't understand the symbolic version. At the very least, I think we should add a sentence at the top of that section explaining that "related" is used in a specialized sense in this section, although I still think that finding a different word would be a better solution. CodeTalker (talk) 23:56, 20 March 2017 (UTC)
- I don't actually object to using "precedes" in the lead, but only because it is the lead and I consider it a bit of creative sloppiness. It gives the right impression for perhaps the wrong reasons. I don't think that this was done intentionally, whoever wrote it probably just uses "precedes" in all instances of the partial order relation (order-theorists do this because they are looking at the subject as a whole and not examining individual examples). However, in the formal definition, the properties being discussed are properties of general relations so using "precedes" there is putting the cart before the horse ... you don't yet have a partial order when these are being discussed. The only way to get around the math/English problem is to go completely symbolic (a la Russell and Whitehead) but this is useless if your intent is to convey meaning and not just information. The terminology is standard and attempting to change it (even for good reasons) would be considered WP:OR because it wouldn't be used in reliable sources. I grabbed a handful of math texts from my bookshelf that include something about order relations and they uniformly use the terminology: if (a,b) is in relation R then we say that "a is related to b" or sometimes "a is R-related to b". So I think that finding a different word just won't work, but I'm okay with adding something about the usage of the word "related" in this context.--Bill Cherowitzo (talk) 03:52, 21 March 2017 (UTC)
- Ok, can you see what you think of this version in my sandbox: [1] I've added a statement about "related to", and also reworded the description of antisymmetry, which I think is a little clearer. CodeTalker (talk) 00:07, 23 March 2017 (UTC)
- Looks fine to me. Go ahead and make the changes. --Bill Cherowitzo (talk) 04:31, 23 March 2017 (UTC)
Youtube
Hi, someone placed a link to a Youtube video within the article Rytz's construction. Is that OK ? Or should it be in a section weblinks ? I am not quite familiar with the WIKI-rules. Could You have a look ? Thanks !--Ag2gaeh (talk) 05:29, 18 April 2017 (UTC)
- Ok. I'll take a look at it. --Bill Cherowitzo (talk) 16:58, 18 April 2017 (UTC)
- English Wikipedia guidelines are that such links belong in an external link section. However, links in that section are subject to greater scrutiny due to several possible abuses that can occur. In particular, links to YouTube by new editors (which is the case here) are automatically removed by a bot. To over-ride the bot some justification is usually needed. Although I did not see any obvious fault with the video, I could not determine who created it and thereby establish its reliability. Without some assurance of reliability, I did not think that I would over-ride the bot, so I just removed the link from the article. If you think the video really belongs in the article, either of us can re-add it to the external links section and the bot will not remove it.--Bill Cherowitzo (talk) 17:56, 18 April 2017 (UTC)
- Thank You ! The creator of the link had first inserted a link to another video, which was rather confusing. So I removed it. The new one is correct. I added a section "External links" and inserted the link there. Hope, its OK. Thanks !--Ag2gaeh (talk) 20:38, 18 April 2017 (UTC)
Even though we disagree, I do thank you for seeing my edit as good faith.LakeKayak (talk) 15:14, 23 April 2017 (UTC)
- You are welcome. I don't always mark edits as being of good faith, so when I do, I mean it. I was getting ready to do some clean-up on your edit and it struck me that even though it would be a shorter proof it was just a manipulation of trig identities and didn't add much in an encyclopedic sense. The original proof, a bit more complicated, had the advantage of referring back to the triangle, and so, may have provided a bit more insight into the identity. This was a fairly close call on my part and I don't want to belittle your effort. --Bill Cherowitzo (talk) 16:34, 23 April 2017 (UTC)
Thank You
I thank You very much for Your patience and so many improvements of my contributions ! --Ag2gaeh (talk) 07:03, 22 April 2017 (UTC)
- You are welcome, but thanks aren't necessary. I think you make valuable contributions and I am only too glad to help. I do hope that you forgive me for introducing an error every once in a while–that's just me thinking too much about the English and not paying enough attention to the mathematics.--Bill Cherowitzo (talk) 17:01, 22 April 2017 (UTC)
- Sorry, but our effort had been perhaps in vain. See the discussion at Talk:Axonometry. The German article on Axonometry has the most daily clicks (60/d) within the descriptive geometry articles. By the way SharkD has drawn the discussion on my "poor" English to Wikipedia:Village pump (miscellaneous). I think I take a break. Bye !--Ag2gaeh (talk) 16:10, 24 April 2017 (UTC)
- Sorry, but our effort had been perhaps in vain. See the discussion at Talk:Axonometry. The German article on Axonometry has the most daily clicks (60/d) within the descriptive geometry articles. By the way SharkD has drawn the discussion on my "poor" English to Wikipedia:Village pump (miscellaneous). I think I take a break. Bye !--Ag2gaeh (talk) 16:10, 24 April 2017 (UTC)
- Taking a break may be the wisest course of action at this point. I had thought that SharkD's position had been softening of late and I am more concerned with John Blackbourne's comments, as he may have a point about the Axonometry article. There are differences between what is acceptable in the German versus English versions of Wikipedia and this article might fall into that gap...I need to think about this. SharkD is, at the moment, defending the article, so we should wait to see how this turns out. --Bill Cherowitzo (talk) 17:10, 24 April 2017 (UTC)
Line Segment and Euclidean distance
Hi, I was informed you reverted my edit on Line Segment. https://en.wikipedia.org/w/index.php?title=Line_segment&oldid=prev&diff=777931485 I probably did not get my message across, which might of ended up in the Talk page, but I was trying to be constructive. The problem is, kids at school get set problems dealing with the size of segments, circumferences and areas, but nowhere in this article does it hint to how a segment is measured, or why a segment should be linked to a distance. I have tried to make amends by editing the paragraph in Euclidean distance. Any revision would be welcome. Apparently it is considered too elementary to mention, or too circular to define, but I do feel there is a gap in the current set of articles. https://en.wikipedia.org/w/index.php?title=Euclidean_distance&action=history Ziounclesi (talk) 13:22, 1 May 2017 (UTC)
- My revert was based on my perception that the statement was too vague to be called a property. I was hoping that a more detailed attempt would be forthcoming. I do however see your point, there is a gap that needs to be addressed. I took the liberty of expanding your edit to Euclidean distance and moving the paragraphs around since this needed to be stated first. If you like what I have done we can think about how the statement in line segment should be phrased. --Bill Cherowitzo (talk) 17:51, 1 May 2017 (UTC)
- Thanks for your further revision, and for taking time to understand my viewpoint. In my haste I forgot the direction, thanks again for fixing that. Getting back to line segment, in the Proofs paragraph, it quotes the Segment addition postulate and jumps into the concept of distance, which, in my ignorance of higher mathematics, does not seem to be mentioned in the Properties paragraph. Now, please allow me a wee provocation: length is the only feature a segment has http://www.dummies.com/education/math/geometry/how-to-measure-line-segments/ :-) Now, WP is not for dummies, but many elemetary geometry problems for juniors revolve completely around the relative size of segments and angles. How to explain or fix this omission? "Historically, the basic property of a segment is its length. In Euclidean geometry, two dimensional shapes are built using segments, and defined by the number of segments, relative size and joining angle. Theorems and proofs describe the relationships between shapes, segments and angles. The idea of segment is intimately tied to a metric. This viewpoint was overturned at the beginning of the 20th century, by the rise of mathematical logic, that aimed to unbundle numbers, theorems and proofs from any physical representation, like size and distance." Ziounclesi (talk) 19:34, 3 May 2017 (UTC)
ANI notice
![]() There is currently a discussion at Wikipedia:Administrators' noticeboard/Incidents regarding an issue with which you may have been involved. Sławomir Biały (talk) 11:59, 6 May 2017 (UTC)
There is currently a discussion at Wikipedia:Administrators' noticeboard/Incidents regarding an issue with which you may have been involved. Sławomir Biały (talk) 11:59, 6 May 2017 (UTC)
on Prototypehumanoid's edits
I put notes on NeilN's and Meters' talk pages to inform them about the recent Calculus edits, since both already warned him. Regards, Purgy (talk) 08:06, 27 May 2017 (UTC)
Graph Theory
Hi, I've added links to open source, academic (all MIT or BSD licensed) software stacks that help one to simulate graph theory in practice. Current article is all talk, no action. They are removed with the reason "link farm". I'm confused. Github, the platform that hosts these software free and show no ads, is no more for-profit than Wikipedia. Please advise. — Preceding unsigned comment added by Esokullu (talk • contribs) 20:39, 31 May 2017 (UTC)
- It is the practice here (as opposed to other places on the web) to put new remarks at the bottom of a talk page, so I have taken the liberty of moving yours here. As to your issue–there is nothing wrong with the links that you have tried to add to this Wikipedia page. The reason that they have been removed (twice now) has to do with the nature of Wikipedia. There are several things that Wikipedia is not (see Wikipedia:Not) and one of these is that WP is not a collection of links (a linkfarm) to other sites on the web. When links are made, they have to provide information about the subject of the article that is not adequately presented in the article. This is an encyclopedia, not a nexus for activities surrounding an article's topic. There are plenty of places on the web that one can go to for such activities, but to try to maintain some semblance of integrity and purpose, WP is not one of them. This is a shared belief in the Wikipedia community and many editors will remove such links when they see them. --Bill Cherowitzo (talk) 21:31, 31 May 2017 (UTC)
Wrote a response to your recent undo of my changes to "vacuous truth" article. I think your undo was a bad decision.
I added a section to the vacuous truth article's talk page (link), entitled "Article quality has become poor. Has inconsistencies and also needs explanation of why vacuous truth exists.".
It seems to me that if this is how the editing culture is on Wikipedia then I should probably spend my time elsewhere, somewhere with a more reasonable culture. My time is valuable, and I shouldn't have to argue over even the most basic and obviously useful changes.
--MagneticInk (talk) 17:24, 5 June 2017 (UTC)
Thanks!
Dear Wcherowi
I learned several materials related and know follow things.
1 Avoid linking to Arxiv. That's not allowed by them.
2 There is risk about conflict of interest with journal to which my paper will submit.
3 Wikipedia articles must not contain original research. I post it on talk page and want to ask other people to give me their opinions about it then I can improve my work. It’s difficult for me to discuss my work with people. I’m an engineer and don’t work in a university. However contents in my post are enough simple to be understand. I want to know if my statement is clear enough and how difficult for it to be accepted by people. I participated ICM 2010 in Hederabad. Woodschain175 (talk) 10:11, 26 June 2017 (UTC)
Orthogonal lines
Hi ! I wrote a new version of orthogonal trajectory. An essential statement there is: two lines intersect perpendicular, if . My question: Where (in en.wp) is this simple statement hidden ?. I would like to insert a link to this statement.--Ag2gaeh (talk) 11:55, 24 July 2017 (UTC)
- I found it at Perpendicular#Graph of functions, but this is well hidden for such a basic result.--Bill Cherowitzo (talk) 15:49, 24 July 2017 (UTC)
- Thank You ! --Ag2gaeh (talk) 20:37, 24 July 2017 (UTC)
Axioms
No indeed axioms are not proven(notice causality direction). Rather it is "a thing is a proof(proven), and THEN" that is an axiom, so yes: proof --> axiom; and: no(i.e. false) axiom--->proof. Yes, yes. Perhaps you may see this then make that change yourself. Sinsearach (talk) 00:40, 25 July 2017 (UTC)
- I am sorry, but your statement above makes no sense in English–which I am assuming is not your native language. You may try to express yourself more naturally in your own language and then get it translated. --Bill Cherowitzo (talk) 02:06, 25 July 2017 (UTC)
- oh wow am I _RED_ I... I just dont know, I see that I was tired and rushed but this is very much my fist language. Wow.... Wowowowowowow. Just wow. Lets try again. An axiom is not proven, it *CAN* be something that *HAS* been proven. I.e. proof comes first, then a given thing can be an axiom, not the other way around. Sinsearach (talk) 11:29, 25 July 2017 (UTC)
- Alright, that is now clear (sorry about my false assumption), however it is not correct as stated. In order to make this a true statement you would need to be talking about a subject that has more than one set of defining axioms, say some theory X is given by axiom system A and also by a different axiom system B. It is possible that an axiom in system A can be proved from the axioms of system B, and so, would be a theorem of X and vice versa, however, within a fixed axiom system an axiom is a statement which is and can not be proved from the other axioms. What may be confusing you is the regrettable tendency of some modern textbook authors of calling any result that they do not wish to prove at a particular time in the narrative an axiom. This is particularly true in modern geometry texts, where early results are hard to establish, so they are brushed over by being called axioms and are then proved later when more tools are available. In a properly developed axiom system, each axiom is shown to be independent of the other axioms (meaning it can not be proved from them).--Bill Cherowitzo (talk) 21:32, 25 July 2017 (UTC)
basic proportionality theorem
Concerning the revert [2], I agree with the revert because the information made little at that location. However the IP was not completely wrong either the "basic proportionality theorem" is another name for the intercept theorem, which is also called Thales' theorem, but the article already links to that with a had note anyhow. It seems "basic proportionality theorem" is particularly common with Indian authors and (English) math education in India (judging by Google). --Kmhkmh (talk) 19:21, 13 August 2017 (UTC)
- Mea culpa. I must plead American myopticism (wd?). While I do know that you use "intercept theorem" as the name of
this resultThales theorem, it is rare to find a name attached to the right angle result in American English elementary geometry texts, and when one does appear "Thales theorem" seems to dominate.I think that this might reflect the fact that these other names do not provide the visual sound bite of right angles in circles. Granted, Thales theorem doesn't either, but it is at least neutral in this regard.When I see the term "Thales theorem", I do not associate it with the intercept theorem, so I wasn't thinking about alternative names. Thanks for pointing out my blindfolds; I'll try to be more careful next time. --Bill Cherowitzo (talk) 19:51, 13 August 2017 (UTC)
ANI
![]() There is currently a discussion at Wikipedia:Administrators' noticeboard/Incidents regarding an issue with which you may have been involved. Scr★pIronIV 16:29, 25 August 2017 (UTC)
There is currently a discussion at Wikipedia:Administrators' noticeboard/Incidents regarding an issue with which you may have been involved. Scr★pIronIV 16:29, 25 August 2017 (UTC)
Infinity
Dear Sir or Madam,
Please inform me why did you revert my edits on Wikipedia page titled Infinity.
Regards, Wilkn. — Preceding unsigned comment added by 2601:C0:C401:190:0:0:0:2 (talk) 13:02, 23 August 2017 (UTC)
- We typically place new comments at the end of a talk page (unlike other places on the web), so I have taken the liberty of moving yours to the end.
- As to why I reverted your addition to the infinity article, you should know that this had nothing to do with the content that you added, I am taking no position on that. Statements and claims of the type that you wanted to add have to be supported by citations to Wikipedia:Reliable secondary sources. This is not an option, it is a fundamental cornerstone of WP. Editors do not add their own opinions and beliefs to the encyclopedia, rather, they report what is found in the reliable literature. At the top of the editing page there is a disclaimer that says that any unsupported additions may be removed at any time. If I hadn't done this, some other editor would have. What you need to do is to find some reliable sources that support your claims in order to add them back with references. Note that "reliable" here means that the credibility of the source will be judged by various editors, so some effort is needed to make sure that the sources are good ones. --Bill Cherowitzo (talk) 17:26, 23 August 2017 (UTC)
Dear Bill:
Thank you so much for your detailed explanation. Coming specifically to my edit, I did give citations from Wikipedia page itself. Please let me know what/where else would you (or any other editor) need so that the useful information may be added to the immense repertoire of Wikipedia knowledge. I am pasting my edit below for ease of reference.
The concept of infinity first originated in the Indian civilization as one of the mantras of Brihadaranyaka Upanishad and Ishavasya Upanishad popularly known as Shanti Mantra, around 700 BCE.
ॐ पूर्णमदः पूर्णमिदम् पूर्णात् पूर्णमुदच्यते | पूर्णस्य पूर्णमादाय पूर्णमेवावशिष्यते ||
which means. [If] That is complete (infinite), this is complete (infinite), from completeness comes completeness (infinite = infinite) Complete (infinite) minus complete (infinite), infinite remains. (infinite - infinite = infinite)
My apologies for not adding my comment at the bottom. As you may have guessed, I was not aware of the norm.
Regards, Wilkn — Preceding unsigned comment added by 2601:C0:C401:190:0:0:0:2 (talk) 23:49, 23 August 2017 (UTC)
- Apologies are not necessary, this happens frequently. While the links you have included are good (they permit readers to get more information on a topic) they can not be used as sources to verify your statements (Wikipedia is not considered a reliable source for Wikipedia!). You have provided a translation and an interpretation of this mantra. In my (limited) understanding of these matters, authorities often disagree on either or both of these for very old texts. For the purposes of Wikipedia, you must supply citations to whichever authority provided the translation/interpretation of the mantra, and where this was published (so that readers can verify for themselves that this correct).--Bill Cherowitzo (talk) 00:16, 24 August 2017 (UTC)
- Thanks Bill!
I guess, possibly because you belong to western civilization, you are considering Sanskrit more esoteric than it really is. Like we are conversing in English, similarly, we converse in Sanskrit as well. Sanskrit has roots and words originate from roots. What you are asking of me is equivalent to asking me to provide citation of each and every word that is every written in Wikipedia. If you look at complex analysis and non-standard analysis in the same article, there are no citations for even statements there. In the case in question, I am providing citation of where the mantra occurs and in which text.
Lets take the example of the fist word - poorna-adah. Poorna means complete - adah means that. The second word: Poorna-idah, again poorna means complete and idah means this. I am totally at loss as to why would you want me to provide citations for word meanings. Could you please clarify? At worst, I think you can just add "citations needed" to my post?
Regards, Wilkn. — Preceding unsigned comment added by Wilkn (talk • contribs) 04:36, 24 August 2017 (UTC)
- Languages, like English or Sanskrit, change over time and the longer the time interval the greater the change. Meaning of words are not static, they have morphed into what we use today and it would be a mistake to assume that what they meant when they were written is how we interpret them today. This is why we need scholars of ancient texts who study how words have changed and how they haven't to provide appropriate interpretations. And it is not just the words; the context in which things were written has much to do with the meaning. Things are even more complicated when a translation from another language is involved. Translation is not an exact science, as many computer specialists who have attempted to quantify it have discovered. I was recently involved in trying to get a translation of a single sentence written in French by Pierre de Fermat around 1465 CE (less than 600 years old). Several French translators came up with their renditions, and they did not agree with each other. There was a subtle choice of words that Fermat used that changed the meaning of the printed words and it was only after a scholar who was familiar with Fermat's letters weighed in did we get what I think is the correct translation. Now the mantra you've quoted may not be as complicated as this situation, but the age of the mantra surely indicates that some authority needs to be quoted as saying that "this is what the mantra is in fact saying". Also, the claim that this is the first occurrence of the concept of infinity in Indian culture would need to be verified by citing some authority on ancient Sanskrit writing who would be in a position to know this.--Bill Cherowitzo (talk) 21:03, 24 August 2017 (UTC)
I am respectably disregarding the part before "Now the mantra you've quoted...," because as you rightly stated it is not relevant. It is a very simple Sanskrit sholoka, meaning of which is universally accepted. Similar to English language we are conversing in.
As per the Wiki philosophy of finding a solution to move forward, I have following proposals as citations:
0. https://en.wikipedia.org/wiki/The_Principal_Upanishads (This is a book written by Second President of India) — Preceding unsigned comment added by Wilkn (talk • contribs) 00:54, 25 August 2017 (UTC) 1. http://www.hinduwebsite.com/sacredscripts/hinduism/parama/isa.asp 2. http://siddharthssinha.blogspot.com/2013/02/isha-upanishad-yajur-veda-part-of-four.html 3. http://aumamen.com/mantra/om-purnamadah-purnamidam-shanti-mantra 4. http://pushpagowda.blogspot.com/2016/01/meaning-of-shanthi-mantra-poornamadah.html 5. http://www.eaglespace.com/spirit/poornamadah.php 6. https://www.quora.com/Is-the-meaning-of-the-Sanskrit-shloka-Om-Purnamadah-Purnamidam-very-similar-to-the-continuum-theory 7. We leave it as is and have "citations needed"
What do you think?
Regards, Wilkn (talk) 01:00, 25 August 2017 (UTC) — Preceding unsigned comment added by Wilkn (talk • contribs) 00:50, 25 August 2017 (UTC)
- Have you looked at Wikipedia:Reliable secondary sources? None of these citations qualifies under those criteria. Moreover, what they do say just underscores the points that I have been trying to make. Look at reference 6 more closely ... he talks about the vagarities involved with translation of ancient Sanskrit text. Many of the translations you cited do not use the word "infinite" at all and the picture I get is that the mantra has different meanings for different people. It is not clear to me why one interpretation should be considered more correct than any of the others.--Bill Cherowitzo (talk) 04:40, 25 August 2017 (UTC)
Mr. Cherowitzo, I am charging you with bias. The very first source is a book, which is considered a reliable source according to your own citation. Further, I am citing exactly from the original source, which again is the most authentic form of the citations as per your citation. You are not ready to accept that as well. Third, all the citations that I provided do indeed have the word infinite, and you wrote that it is not the case. I think, and I say that based on proofs, you are not able to digest that the concept of infinity originated in India way before than other civilizations, therefore, you are are finding lame excuses to justify your personal bias. Respectfully, I seek a neutral adjudicator. (vagarities - did you mean vagaries?)
Regards, Wilkn (talk) 05:26, 25 August 2017 (UTC)
- You may charge me with whatever you like, but the only one demonstrating any bias here is you. I have not argued for or against the inclusion of this statement, I have only taken the position that if it is to be included it needs to have good references. The book you mentioned could be such a reference (you would need a page number and perhaps a quote) but you only gave me a wiki page about the book which as I have said before can not be used as a reference (nor does the page mention anything in support of your claim). Your reference 3 does not use the word "infinite". Reference 2 argues that the word "infinite" is too confining and should not be used. This reference also has a disclaimer that says that exact translation of the mantra is not possible. The blogs in your list are not considered reliable sources. You have put blinders on and you are not listening to what I am saying or what your references are saying ... this is true bias. You have asked me why I reverted and I have gone to great lengths to respond to this request. Until you come up with a reliable source, there is nothing more that I can say.--Bill Cherowitzo (talk) 17:25, 25 August 2017 (UTC)
How can you cite, the same sources for your argument, if those sources do not qualify as proper sources? Such behavior, in my opinion, is bias.Wilkn (talk) 15:43, 29 August 2017 (UTC)
- You seem to be under the impression that I am arguing with you ... I am not. I have not been trying to make any points concerning the statement and have only been talking about the need for good reliable sources in this matter. Wikipedia has standards with regards to what can be considered as a reliable source. Blogs, personal webpages, websites without specific authors, etc. are expressions of personal opinions and do not meet this standard. That does not mean that what is contained in those sources is necessarily false, just that one can not use them as a basis for inclusion in WP. I do not have any independent sources for this material, so I looked at the ones you provided. It was clear to me that they did not support your point of view, and several were saying the same thing that I was saying (and you chose to disregard) about interpretations. Since you provided these sources I assumed that you would believe what was contained in them, so I pointed that out, and I have no reason not to believe in their content. Your concept of bias is rather interesting as well. It appears to me that you think that anyone who you perceive as not agreeing with you is biased.--Bill Cherowitzo (talk) 16:39, 29 August 2017 (UTC)
Edit waring
Hi, I have edited some articles on Wikipedia about Algebraic geometry and Sharaf al-Din al-Tusi and you reverted my work without providing any explanations but your own opinion, so i'm here to know why. My sources where University St Andrews and Roshdi Rashed. You said that the great mathematician and historian "Roshdi Rashed´s bias is this matter is well known", this looks like a personal opinion. If i'm wrong, could you please provide a reliable source supporting your claim ? if not, please avoid reverting orher contributors edits without providing a legit reason.
- On Wikipedia talk pages new comments are added to the end, so I have moved yours to the appropriate position. Also, we sign our messages (using 4 tildes as requested at the top of the edit page).
- I would suggest that you take a look at WP:BRD as this is the way we conduct ourselves. Your edit was Bold, I Reverted and the next step is to Discuss the difference on the talk page and arrive at a consensus. Instead you simply replaced your original edit. This is the definition of edit warring that you, and not I, have engaged in.--Bill Cherowitzo (talk) 16:54, 3 September 2017 (UTC)
I had a look at WP:BRD as you wanted, so can we now discuss about the real problem which is the removal of sourced informations ? I see nothing in your message or on Wikipedia which supports you doing so. And as you're giving me lessons about how Wikipedia works, i would like to inform you that removing of sourced materials is forbidden on Wikipedia. I can see on your page that you are a mathematician, and i inform you that so i am (i studied PhD in the field of stochastical calculus at the University Pierre et Marie Curie in Paris and i expect a real answer from you as you're speaking to someone who knows the field of mathematics...). I'm open to discussion and waiting for your explanation about your statement on Roshdi Rashed who is a prominent scholar about this topic (just have a look at his Wikipedia page...). By the way, as i don't have a Wikipedia account, i can't sign my comments, i'm sorry for that.
- Sorry, I was called away from my computer before I could finish responding to you. This discussion belongs on a talk page of one of the affected articles, but I'll give you the brief version here. Although I generally respect the work done at the St. Andrews site, there are some places in which they are weak, in particular, the essays that can be found at the site and some of the pages linked to those essays do not have the same integrity as other work that is found there. Various Wikipedia editors have commented on this over the years and I am in agreement with them. As to the "great mathematician and historian" (now that sounds like a personal opinion to me!), his wiki page contains a number of unsubstantiated claims and I see no evidence of any secondary sources backing them up. When other math historians have examined his work they have uncovered clear examples of bias. For example, look at J.L. Berggren's review of Rashed's Encyclopedia of the History of Arabic Science in the Journal of the American Oriental Society, vol. 120, #2, 2000 (pp. 282-283). There are several places in Wikipedia articles where Rashed's over-exuberance for all things Arab have been called into question. I am not in a position to evaluate his work, but I've seen enough commentary to make me skeptical and desire to see more than just his opinion on something. Part of the job of an editor is to evaluate how reliable a source is and when this is questioned, several editors need to weigh in and form a consensus. (Typing in 4 tildes will produce your IP number if you are not registered ... and that will permit me to know if I am talking to the same person or not) --Bill Cherowitzo (talk) 20:26, 3 September 2017 (UTC)
I understand your point of view but all i have seen about Rashed is elogious, the guy works for CNRS in France and owns a medal from that prestigious intitution, so, when i say he is a great mathematician and historian, this is not my personal opinion (as i don't know him) but only the reflect of what i have red about him... As long as Rashed is believed to be a legit source and cited in various articles (on Wikipedia and elsewhere), you should not revert edits which cite him as source (and in our case, Sharaf al-Din al -Tusi is not Arab but Persian) claiming that he is not legit. Just have a look at Wikipedia's articles about medieval mathematicians and you will find innumerable articles citing Rashed as a legit source. Berggren mat have a different point of view, but who can say if he is right or if Rashed is ? All we can say then is that sources are not unanimous, do you agree ? Thanks for the time you spent about this topic. (I don't know what you mean by "tiping in 4 tildes", until now, when i used the talk page on Wikipedia, an automata called "sinebot" signed my messages...)
Gaussian Correlation Inequality
Yeah, so, my edit was completely true. I get that u deleted it without actually checking with Mr. Royen, bc I am still basically an unknown at this point, but this was one of those circumstances where the mistake was on yalls' end. However, no hard feelings on my end; my first edit was far too specific, was long-winded, and did read too much like a story. So, all is forgiven. Have a great, productive day.
Sincerely, Joshua H. Lempert Jlempert87 (talk) 11:35, 25 September 2017 (UTC)
- Hi Joshua. First person accounts just don't wash on Wikipedia; truth or falsity is not even an issue. If you want your side of the story to be told it would have to be published somewhere before it can actually be considered. --Bill Cherowitzo (talk) 15:49, 25 September 2017 (UTC)
Ok, awesome. That's what I needed to know. Thank you. I'm gonna swing for the Mensa Bulletin, but we'll see where it ends up being published. Thank you for ur time and info. Hopefully, it won't be too long before I can get my name back up there. Many thanks, and have a productive day! Jlempert87 (talk) 20:09, 25 September 2017 (UTC)
Sharaf al-din al-Tusi
Hi, my edit on the Sharaf al-Din al-Tusi's page is supported by the following sources:
University of St Andrews, Oxford islamic studies and Science and Religion Around the World (Oxford University Press). You said my edit is weakly sourced, can you explain me why ? Farawahar (talk) 16:46, 5 October 2017 (UTC)
- Sure. Good sources are secondary sources which use and reference the primary sources that they use to draw conclusions. All three of these are tertiary sources and the second two appear to be inspired by the St. Andrews site, having no references and not going beyond what is said at the St. Andrews site. The St. Andrews site at least references Rashed as the source of this interpretation and the wording that you replaced was a more faithful rendering of Rashed's actual statements, with direct reference to his work. So, you have replaced a good source (which personally I don't happen to agree with, but my opinions on the matter are not germane) with three poorer sources. --Bill Cherowitzo (talk) 17:40, 5 October 2017 (UTC)
Sorry, i think you're wrong. The source cited in your version is Rashed's book (edited in 1994) and cited by St Andrews. So thiis source is also in my version but if it pleases you, i will remove St Andrews and replace it by Rashed, they roughly say the same thing. Even if you want to remove the second source (which, according to you, is Rashed again...), the third one (Oxford islamic studies) is a reliable secondary source. So my version is richer when it comes to sources and more complete, because your version makes people think that only Rashed is thinking that Tusi introduced algebraic geometry, and this is blatantly wrong. Farawahar (talk) 21:28, 5 October 2017 (UTC)
- First of all, this is not my version as I did not write it, but I do support it. What evidence do you have that the Oxford Islamic Studies reference is a secondary source? There was no evidence of that from the link that you provided, and if you have additional information about this source you would need to make that available.--Bill Cherowitzo (talk) 05:03, 6 October 2017 (UTC)
Not to sound like a broken record... If i understand well, according to you, Rashed and Oxford islamic studies are not reliables, what proof do you have for that ? If it's the best you can do, then i'll revert your edit again because as you can see below, Oxford islamic studies IS a reliable source for this topic :
http://www.oxfordislamicstudies.com/Public/about.html
"This authoritative, dynamic resource brings together the best current scholarship in the field for students, scholars, government officials, community groups, and librarians to foster a more accurate and informed understanding of the Islamic world. Oxford Islamic Studies Online features reference content and commentary by renowned scholars in areas such as global Islamic history, concepts, people, practices, politics, and culture, and is regularly updated as new content is commissioned and approved under the guidance of the Editor in Chief, John L. Esposito."
Farawahar (talk) 08:09, 6 October 2017 (UTC)
- Please read my comments more carefully. The version I would like to see is directly sourced to Rashed, so how can you claim that I have indicated that he is not a reliable source? This link to the Oxford Islamic Studies On-line is a much better link to that source and it shows, beyond a doubt, that this is not a secondary source, at least for this material. The biography listing clearly indicates the sources of its material and all of the sources given are encyclopedias of one type or another. Encyclopedias are tertiary sources themselves, so this source can be no better than a tertiary source. Also, you need to register at the website to obtain the information on the site, and this is problematic for a Wikipedia source. Your quote from the site is all very good, but realize that it is a piece of self-promotion and not a third party evaluation of the site.--Bill Cherowitzo (talk) 19:02, 6 October 2017 (UTC)
Algebra
I think this is not a reliable source-https://www.algebra.com/algebra/about/history/ .Better source template ( [better source needed] ) should be added after Reference number 13 of the article ( Algebra ) F0r★binIV 19:47, 21 October 2017 (UTC)
- I agree. This is just a Wikipedia mirror site, of which there are many on the web, and so, totally useless as a source. The statement that this is supposed to support will probably have to be changed as well. Being at a "higher level" is not something that a reliable source is likely to say. I think that the intent might have been to say that they developed the subject more deeply (more broadly?) than their Egyptian and Babylonian roots. The exact phrasing will depend on the source(s) that we can find. They shouldn't be too difficult to locate, as the statement is not particularly controversial.--Bill Cherowitzo (talk) 22:54, 21 October 2017 (UTC)