User:Tomruen/Uniform honeycombs

This is a list of uniform tessellations in dimensions 2-8, constructed by vertex figures that are uniform polytopes with circumradii equal to 1.0.

Lattice refers to the vertex arrangement of a uniform tessellation/honeycomb. There are also dual lattices, which place vertices at the tessellation facet centers.

The number of vertices in the vertex figure is equal to the kissing number of the tessellation.

Circumradius 1 vertex figures

BSA	Dim	Uniform polytope (vertex figure)	Vertices	Fam	Uniform tessellation	Tessellation, packing and vertex figure	Dual tessellation and facet
	2D	t0,1{3} expanded 2-simplex (hexagon)	6	${\tilde {A}}_{2}$ honeycomb A2 lattice
	2D	{6} hexagon	6	H~2			{3,6} H2 lattice

co	3D	t0,2{3,3} expanded 3-simplex (cuboctahedron)	12	${\tilde {A}}_{3}$	${\tilde {A}}_{3}$ honeycomb A3 lattice
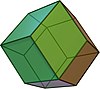
co	3D	t1{3,4} rectified 3-orthoplex (cuboctahedron)	12	${\tilde {B}}_{3}$	h{4,3,4} D3 lattice

spid	4D	t0,3{3,3,3} expanded 4-simplex	20	${\tilde {A}}_{4}$	${\tilde {A}}_{4}$ honeycomb A4 lattice
tes	4D	(4-4 duoprism (4-cube)	16	C~4	t2{4,3,3,4}
tes	4D	{}x{4,3} cube prism (4-cube)	16	F~4	t1{3,3,4,3}
ico	4D	t1{3,3,4} rectified 4-orthoplex	24	${\tilde {D}}_{4}$	h{4,3,3,4} D4 lattice		File:24-cell.svg
ico	4D	{3,4,3} (24-cell)	24	F~4	{3,3,4,3} F4 lattice		File:24-cell.svg

scad	5D	t0,4{3,3,3,3} expanded 5-simplex	30	${\tilde {A}}_{5}$	${\tilde {A}}_{5}$ honeycomb A5 lattice
squoct	5D	{4}x{3,4} duoprism	24	C~5	t2{4,3,3,3,4}
rat	5D	t1{3,3,3,4} rectified 5-orthoplex	40	${\tilde {D}}_{5}$	h{4,3,3,3,4} D5 lattice

stef	6D	t0,5{3,3,3,3,3} expanded 6-simplex	42	${\tilde {A}}_{6}$	${\tilde {A}}_{6}$ honeycomb A6 lattice
squahex	6D	{4}x{3,3,4} duoprism	32	${\tilde {C}}_{6}$	t2{4,3,3,3,3,4}
octdip	6D	{3,4}x{3,4} duoprism	36	${\tilde {C}}_{6}$	t3{4,3,3,3,3,4}
rag	6D	t1{3,3,3,3,4} rectified 6-orthoplex	60	${\tilde {D}}_{6}$	h{4,3,3,3,3,4} D6 lattice
trittip	6D	{3}x{3}x{3} triaprism	27	${\tilde {E}}_{6}$	t2(2_22) honeycomb
nodeip	6D	{}xt2{3,3,3,3} birectified 5-simplex prism	40	${\tilde {E}}_{6}$	t1(2_22) honeycomb
mo	6D	1_22	72	${\tilde {E}}_{6}$	Gosset 2_22 honeycomb E6 lattice

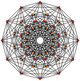
	7D	t0,6{3,3,3,3,3,3} expanded 7-simplex	56	${\tilde {A}}_{7}$	${\tilde {A}}_{7}$ honeycomb A7 lattice
	7D	{4}x{3,3,3,4} duoprism	40	${\tilde {C}}_{7}$	t2{4,3,3,3,3,3,4}
	7D	{3,4}x{3,3,4} duoprism	48	${\tilde {C}}_{7}$	t3{4,3,3,3,3,3,4}
rez	7D	t1{3,3,3,3,3,4} rectified 7-orthoplex	84	${\tilde {D}}_{7}$	h{4,3,3,3,3,3,4} D7 lattice
he	7D	0_33 trirectified 7-simplex	70	${\tilde {E}}_{7}$	Gosset 1_33 honeycomb	File:7-simplex t3.svg.png
	7D	{}x(1_31)		${\tilde {E}}_{7}$	t1(3₃₁) honeycomb
	7D	{3}x(0_31)		${\tilde {E}}_{7}$	t2(3₃₁) honeycomb
	7D	{3,3}x{3}x{}		${\tilde {E}}_{7}$	t3(3₃₁) honeycomb
laq	7D	2_31	126	${\tilde {E}}_{7}$	Gosset 3_31 honeycomb E7 lattice

	8D	t0,7{3,3,3,3,3,3,3} expanded 8-simplex	72	${\tilde {A}}_{8}$	${\tilde {A}}_{8}$ honeycomb A8 lattice
	8D	{4}x{3,3,3,3,4} duoprism	48	${\tilde {C}}_{7}$	t2{4,3,3,3,3,3,3,4}
	8D	{3,4}x{3,3,3,4} duoprism	60	${\tilde {C}}_{7}$	t3{4,3,3,3,3,3,3,4}
	8D	{3,3,4}x{3,3,4} duoprism	64	${\tilde {C}}_{7}$	t4{4,3,3,3,3,3,3,4}
rek	8D	t1{3,3,3,3,3,3,4} rectified 8-orthoplex	112	${\tilde {D}}_{8}$	h{4,3,3,3,3,3,3,4} D8 lattice
rene	8D	0_52 birectified 8-simplex	84	${\tilde {E}}_{8}$	Gosset 1_52 honeycomb
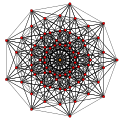
roc prism	8D	{} x 0_51	56	${\tilde {E}}_{8}$	t1(2₅₁) honeycomb
hocto	8D	1_51 8-demicube	128	${\tilde {E}}_{8}$	Gosset 2_51 honeycomb
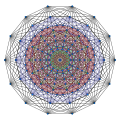
	8D	{} x (3_21)	112	${\tilde {E}}_{8}$	t1(5₂₁) honeycomb
	8D	{3} x (2_21)	81	${\tilde {E}}_{8}$	t2(5₂₁) honeycomb
	8D	{3,3} x (1_21)	48	${\tilde {E}}_{8}$	t3(5₂₁) honeycomb
	8D	{3,3,3} x (0_21)	50	${\tilde {E}}_{8}$	t4(5₂₁) honeycomb
	8D	{3,3,3,3} x {3} x {}	30	${\tilde {E}}_{8}$	t5(5₂₁) honeycomb
fy	8D	4_21 polytope	240	${\tilde {E}}_{8}$	Gosset 5_21 honeycomb E8 lattice

Families =

Infinite Coxeter groups

Families of convex uniform tessellations are defined by Coxeter groups.

n	${\tilde {A}}_{2}+$ eα_n	${\tilde {B}}_{3}+$ Alternated n-cubic^[1] hδ_n	${\tilde {C}}_{2}+$ n-cubic^[2] δ_n	${\tilde {D}}_{4}+$ Quartered n-cubic^[3] qδ_n	${\tilde {E}}_{6}$ - ${\tilde {E}}_{9}$	${\tilde {F}}_{4}$	${\tilde {G}}_{2}$ Hexagonal	${\tilde {I}}_{1}$ Aperigonal
1								{∞}
2	{6,3}		{4,4}				{6,3}
3	q{4,3,4}	h{4,3,4}	{4,3,4}
4	{3^[5]}	{4,3²,4}	{4,3²,4}	q{4,3²,4}		{3,4,3,3}
5	{3^[6]}	h{4,3³,4}	{4,3³,4}	q{4,3³,4}
6	{3^[7]}	h{4,3⁴,4}	{4,3⁴,4}	q{4,3⁴,4}	{3^2,2,2} E6 lattice
7	{3^[8]}	h{4,3⁵,4}	{4,3⁵,4}	q{4,3⁵,4}	{3^3,3,1} {3^1,3,3} E7 lattice
8	{3^[9]}	h{4,3⁶,4}	{4,3⁶,4}	q{4,3⁶,4}	{3^5,2,1} E8 lattice {3^2,5,1} {3^1,5,2}
9	{3^[10]}	h{4,3⁷,4}	{4,3⁷,4}	q{4,3⁷,4}	{3^6,2,1} E9 lattice {3^2,6,1} {3^1,6,2}
10	...	...	...	...

Notes

^ Coxeter, The beauty of Geometry, Wythoff's construction of uniform polytopes, Page 47, hδ_n
^ Coxeter, The beauty of Geometry, Wythoff's construction of uniform polytopes, Page 47, δ_n
^ Coxeter, The beauty of Geometry, Wythoff's construction of uniform polytopes, Page 47, qδ_n

http://books.google.com/books?id=fUm5Mwfx8rAC&printsec=frontcover&dq=Coxeter&hl=en&ei=0jjOS-yZOpKuNvi79BU&sa=X&oi=book_result&ct=result&resnum=4&ved=0CDsQ6AEwAw#v=onepage&q=lattice&f=false

[1] Coxeter, The beauty of Geometry, Wythoff's construction of uniform polytopes, Page 47, hδ_n

[2] Coxeter, The beauty of Geometry, Wythoff's construction of uniform polytopes, Page 47, δ_n

[3] Coxeter, The beauty of Geometry, Wythoff's construction of uniform polytopes, Page 47, qδ_n

[1]

[2]

[3]