Talk:Harmonic series (music)
| This is the talk page for discussing improvements to the Harmonic series (music) article. This is not a forum for general discussion of the article's subject. |
Article policies
|
| Find sources: Google (books · news · scholar · free images · WP refs) · FENS · JSTOR · TWL |
| This It is of interest to the following WikiProjects: | ||||||||||||||
‹See TfM›
| ||||||||||||||
Consonance & dissonance
[edit]Rather than saying
"the difference between consecutive harmonics is constant. But because our ears respond to sound logarithmically...,we perceive higher harmonics as 'closer together' than lower ones"....
would it not be more accurate to say that the distance between consecutive harmonics, as measured in semitones, decreases, but because of our ears respond to sound logarithmically, the frequency difference between consecutive harmonics is constant?
Kj4321 (talk) 17:19, 9 December 2008 (UTC)kj4321
- Here's another try: "The difference in number of cycles per second between the frequencies of consecutive members of any harmonic series remains constant, but because the human ear and brain compare frequencies logarithmically (by division rather than by subtraction), higher harmonics sound closer together intervalically." -- Another Stickler (talk) 19:04, 9 December 2008 (UTC)
- Thanks for the response. Considering first the sound source, before it reaches the listener, each standing wave in a vibrating object with harmonic overtones is larger than the succeeding standing wave (1/2, 1/3, 1/4, 1/5...). And this also conforms with tonal practice, with intervals of the harm series decreasing in size. So is it just the distance between them which is constant? Couldn't this 'constant frequency between harmonic numbers' be an "imprint" of the logarithmic human hearing system? Kj4321 (talk) 03:02, 10 December 2008 (UTC)kj4321
- I don't know. -- Another Stickler (talk) 05:33, 10 December 2008 (UTC)
Not sure that assertion concerning combination tones contradicts overtone series as source of consonance and dissonance.
The discussion designed to rebut the concept of natural harmonic series as source of consonance and dissonance does not argue well. The writer is merely demonstrating that these sine wave frequencies are members of a natural harmonic series, but not the holders of the fundamental frequency. All lowest common denominator calculations are merely indicating the harmonic series to which both frequencies belong. The higher the partial numbers of the two frequencies, the more dissonant those frequencies will be perceived. This article needs cleanup. Davidbrucesmith 07:36, 21 February 2007 (UTC)
Barbershop
[edit]On the use of the term "overtone", this page says: "Barbershop uses overtone colloquially in reference to the psychoacoustic phenomenon of close harmony." But the Barbershop music page and other info I've seen emphasis the use of Just intonation and the resulting resonant "ringing" sound. Unless I'm mistaken (quite possible), I don't think "overtone" just means "close harmony" in barbershop.. ? Pfly 07:47, 9 March 2007 (UTC)
- Concurred. There's no reason wide harmony can't ring too. I changed "close harmony" to "just intonation harmony". -- Another Stickler (talk) 22:19, 9 December 2008 (UTC)
- If memory serves, bluegrass singers call this phenomenon "the bird," referring to a mixing tone heard "above" the actual notes being sung. Can't recall where I read that, but will keep looking. __Just plain Bill (talk) 19:40, 10 December 2008 (UTC)
Overtones are always harmonic up to the 7th degree (the sixth overtone)
[edit]This article is incorrect in:
Terminology...
[edit]| discussion obviated by fix |
|---|
| The following discussion has been closed. Please do not modify it. |
|
Likewise, many musicians use the term overtones as a synonym for harmonics, though not all overtones are necessarily harmonic, some are inharmonic or non-harmonic.
That is, an overtone may be any frequency that sounds along with the fundamental tone, regardless of its relationship to the fundamental frequency. The sound of a cymbal or tam-tam includes overtones that are not harmonics; that's why the gong's sound doesn't seem to have a very definite pitch compared to the same fundamental note played on a piano.
I reworked the terminology section, so I'm folding this part of the discussion. -- Another Stickler (talk) 06:01, 10 December 2008 (UTC) |
Harmonic serie on the moodswinger...
[edit]From what I have experienced all overtones up to the 7th grade are clearly harmonic to the fundamental. If you go behind the 7th overtone, the string doesn't clearly resonate anymore, with the exception of the prime positions (8/1, 9/1, ->16/1). So the main dotted harmonic serie mentioned at moodswinger is the perfect consonant serie related to the fundamental tone. The 2nd smaller line serie (8/1 -> 16/1) mentioned at the instrument are less consonant, because the string only clearly resonates at the prime overtone. (14/1 is clear, 14/3 or 14/5 for example cannot be heard clearly). 8/3 and 8/5 are maybe also consonant, but that's shaky. I'm still considering to put those tones in the scales, because they are important tones for the minor scale. They are the Plutos of the consonant harmonic serie.YuriLandman 07:58, 18 August 2007 (UTC)
Nomenclature...
[edit]I'd like to point out two things: I think everything will be much clearer if the fundamental is numbered as O, the first harmonic as 1, so that the following order ensues: octaves 1,2,4,8,16 etc fifths 3,6,12,24 thirds 5,10,20 sevenths 7,14,28 etc. This order seems logical and certainly practical. Above is mentioned "the 7th degree or sixth overtone which is utterly confusing.
Secondly, nr 11 on the staff notation is given as a g-flat which is wrong as it is a raised f. Normally, special signs such as half flats and sharps come in handy here.Martinuddin (talk) 11:30, 20 July 2008 (UTC)
- Please count again. In the current table Harmonic series (music)#Harmonics and tuning you can see that the order you claim is achieved by starting from the fundamental as 1. −Woodstone (talk) 15:05, 20 July 2008 (UTC)
- Wow. I never noticed that pattern of multiples in the numbers. SharkD (talk) 19:18, 12 August 2008 (UTC)
Table
[edit]A while back the table of harmonic series was changed to a new format. I think the old version was easier for novices to understand. The current table has a two dimensional array in place of a table column, which could perplex readers. SharkD (talk) 19:14, 12 August 2008 (UTC)
- I'm confused by this statement. A few lines above you expressed surprise that the table brings out the pattern so beautifully. In a single column table it is not visible that easily. −Woodstone (talk) 19:23, 12 August 2008 (UTC)
- It's still harder to grasp. It was necessary for User:Martinuddin to point the pattern out to me. I did not notice it on my own, so I don't think it is much more visible. How about adding the current table minus the "Variance" column as a second table? Assuming it is noteworthy, it could be used for the purposes of highlighting this pattern. SharkD (talk) 19:53, 12 August 2008 (UTC)
Disputed
[edit]I see there's already a section above on some disputed stuff in this article, but those aren't what I consider to be the problems. Since it's all unsourced made-up stuff, of course it's largely free to say whatever came to someone's mind, like the nutty idea that an instrument is in some sense like a harmonic oscillator, or that a harmonic oscillator would have harmonics in its oscillation. Nonsense, all; hence, the disputed tag. With a merge, maybe it could be cleaned up, if there's anything here worth merging. Dicklyon (talk) 04:12, 5 September 2008 (UTC)
- Please remember to make some effort at civility.
- I agree that the factual accuracy needs to be reviewed and in several places edited a good bit. But any suggestion that the content of the Article is wholly or even largely contrived is quite wrong. If the implication of the posting of fictional content is meant as an exaggerated criticism of its lack of citation then I do acknowledge that point. But the notion that the physics concepts discussed in the Article were fabricated from the imagination of the author does not ring at all true to me. Personally, I have read a great deal about harmonics as they relate to music but my physics expertise is limited to a handful of honors Physics Dept. classes that I took while in college, largely because I liked the professor.
- Given my relatively novice status I cannot defend or dispute many of the specific details. But I can offer reliable evidence that musical instruments are indeed significantly akin to harmonic oscillators and that the mathematics that describe them are in fact quite useful an Ideal model for the oscillation produced in making music.
- To try to be a bit more succinct I am just going to provide the relevant links for citation and an explanation if necessary.
- http://ccrma.stanford.edu/~jos/SimpleStrings/ a goldmine of information about modeling oscillating strings on musical instruments--discusses that "quai-harmonic" model in relation to the ideal oscillation model
- http://ccrma.stanford.edu/~jos/mus423h/Analysis_Resynthesis_Quasi_Harmonic_Sounds.html This specific page discusses the phrase "quasi-harmonic" to explain the jargon.
- http://books.google.com/books?id=rVq8YiInxz0C&pg=PA106&lpg=PA106&dq=Harmonic+Oscillator+piano+string+dampened&source=web&ots=qojiULCXcx&sig=RL1DEK1ss_CBivSwBGzfm8wkG9I&hl=en&sa=X&oi=book_result&resnum=2&ct=result discusses how dampened harmonic oscillation may be effectively analyzed as ideal harmonic oscillation
- http://hyperphysics.phy-astr.gsu.edu/hbase/waves/string.html#c1 discusses the harmonic nature of an oscillating stretched guitar string
- http://ccrma.stanford.edu/~jos/SimpleStrings/ a goldmine of information about modeling oscillating strings on musical instruments--discusses that "quai-harmonic" model in relation to the ideal oscillation model
- There's obviously plenty of more sources out there. The main point that I mean to demonstrate in this is that, however much cleanup the Article may need, the content is relevant and there are no lack of sources for an ambitious editor. For these reasons I oppose the proposed merge with Harmonic. Korbnep (talk) 06:19, 13 September 2008 (UTC)
- Well and good, but the sources about strings don't mention harmonic oscillators, and the one about harmonic oscillators doesn't mention harmonics. The confusion of these things should be removed. Dicklyon (talk) 22:29, 9 December 2008 (UTC)
Harmonic numbering
[edit]Dicklyon, I undid your replacement of the Harmonic numbering paragraph with something true, because 1) the something true is true but redundant with the paragraphs above it, and 2) the point of the original paragraph is to expose two harmonic numbering systems (not to be confused with overtone numbering or partial numbering). I put the [citation needed] tag on it yesterday, hoping someone might defend it. We don't know for sure that it's not true, just that it's un-cited, and one of the wikipedia policies is to not destroy information, so let's leave it tagged for a while and see if someone champions it. If no one does, then eventually the Harmonic numbering subsection should be removed in whole. There's no need to replace it with anything. I personally suspect that the practice of numbering harmonics by excluding the fundamental is a terminological mistake by people who confused overtones with harmonics, and not worthy of encyclopedic note, but let's wait and see. -- Another Stickler (talk) 04:19, 10 December 2008 (UTC)
- OK, we can wait on that. Dicklyon (talk) 18:49, 10 December 2008 (UTC)
On the sine waves
[edit]Stickler, I don't like your statement about sine waves. The claim "Any complex tone or timbre is a sum of sine waves of different frequencies, amplitude envelopes, and phases" has two problems: first, the phrase "or timbre" is not needed, and is not using the word timbre in the way it's defined in its article; second the statement is defensible only for periodic tones. The way I had it was better: "A tonally complex waveform, such as a musical tone, can be approximated as a sum of sine waves of different frequencies, amplitudes, and phases," since any such decomposition of real sounds is necessarily approximate. Dicklyon (talk) 18:49, 10 December 2008 (UTC)
- I just put the approximation back. Does the article need to expand on the fact that any musically interesting sound varies with time, and so is not strictly periodic, except when observed in a time window on the order of tens or dozens of milliseconds? I find that a lot of people automatically assume a steady mix of partials when talking about tone or timbre, when in fact the dynamic response of the instrument(s) to human input is what brings music to life, and allows it to be shaped by the player(s). __Just plain Bill (talk) 19:00, 10 December 2008 (UTC)
- Probably this is not the article where we need to go into that issue, but we certainly don't want to state things that depend on the illusion that tones are periodic or even stationary. The "approximation" concept can cover a lot of sins. Dicklyon (talk) 22:30, 10 December 2008 (UTC)
- The section is for defining terminology. The first term defined is partial, which I have defined as "any of the composite sine waves in a complex tone," and no one disagreed. Are either of you now saying there's something else beside sines in a complex tone? -- Another Stickler (talk) 03:01, 11 December 2008 (UTC)
- Yes, of course. The breakdown of things into sines is almost arbitrary, and many ohter decompositions are known and used. However, sines are the things to which the concept of "frequency" applies, and so they are the things that harmonics, partials, overtones, etc. refer to. If you'd like to see a bit of what's wrong with thinking of our ears as Fourier analyzers (that is, of trying to break everything into sines), see my talk about modeling how hearing works, in which I make Ohm and Helmholtz and Fourier the "bad guys": http://www.archive.org/details/Helen_Wills_2007_10_01_Richard_Lyon Dicklyon (talk) 03:31, 11 December 2008 (UTC)
- Unless the signal exactly repeats itself endlessly, it cannot be said to be a sum of sines. Better to say it may be approximated by a sum of sines. Real signals' harmonic composition varies with time, introducing complications. Fourier transforms of a time-limited signal segment have their own issues to do with the time window being sampled; shaping the edges of that window may be done various ways to serve various analytic needs. I am only vaguely familiar with that much of the iceberg's tip, and I agree with Dick (who knows a whole lot more about it) that the "approximation" concept covers as much as we need to go into in this article. __Just plain Bill (talk) 03:41, 11 December 2008 (UTC)
- There's so much to respond to, this may be long. Sorry. My version of the old paragraph wasn't describing Fourier. The Fourier series is only a harmonic series, plus an out-of-phase clone of that series, plus amplitude information for both. I changed the original editor's "amplitudes" to "amplitude envelopes" to make it describe all tones including non-repeating tones. I don't mind dropping "or timbre" since it means tone color, which is implicit in tone, which is inherited by complex tone. Wave-form, sound, signal, and tone are equivalent, but we shouldn't complicate the article with needless synonyms. I don't like the addition of "such as a musical tone" because it opens the can of worms of judging what is musical vs. what is not, and it allows the reader to misinterpret that non-musical tones might not be included in the description. My last question above (is there anything else but sines) was rhetorical, but I guess it didn't make my point. The point is that the mainstream model for the composition of sound, modulation of the frequency spectrum, is sufficient for all sounds. Each point in the spectrum is a frequency, which is a sine. Any tone is therefore composed of sines just as the number line is composed of points. No mention needs to be made that there are an indefinite number of points. That's the model we can assume here. If there are alternative models, I'm not aware of them, but we can create new articles for them. "Unless the signal exactly repeats itself endlessly, it cannot be said to be a sum of sines." J.p.B., I have two answers to that. First, the simplest example that proves that your rule is not true is the non-repeating signal that is the sum of two sines at an irrational frequency relationship. Second, any signal, repeating or non-repeating, is the sum of sines if you include the whole spectrum, if you allow that they don't all have to be in phase, and allow for amplitude changes over time. D.l., I can't get your video links to work on my computer/browser combination. It looks interesting, but it's about models for hearing, which is outside the scope of this article. I would really like to learn more about it, but not here. Here's a reworked paragraph I would like to put back in: "Any tone is a sum of sine waves of different frequencies and phases, changing in volume over time." That describes the mainstream model in a simple sentence, it accounts for all sounds, repeating and non-repeating, musical and non-musical, and sets the foundation for the following paragraphs which define terms starting with partial. -- Another Stickler (talk) 05:18, 12 December 2008 (UTC)
- Oops, I meant this one: "Any complex tone is composed of sine waves of different frequencies and phases, changing in volume over time." —Preceding unsigned comment added by Another Stickler (talk • contribs) 05:24, 12 December 2008 (UTC)
- "Changing in volume over time" implies other spectral components (representable as phased sines again, but still a needless complication for this article.) I'd prefer to see "Any complex tone may be approximated as a sum of sine waves of different frequencies and phases." and leave it at that.
- Oops, I meant this one: "Any complex tone is composed of sine waves of different frequencies and phases, changing in volume over time." —Preceding unsigned comment added by Another Stickler (talk • contribs) 05:24, 12 December 2008 (UTC)
- I suppose inharmonic partials do deserve passing mention, but the context of the article is, simply put, the harmonic series. __Just plain Bill (talk) 06:04, 12 December 2008 (UTC)
- Originally Stickler wrote it with justification via the Fourier theorem, which only applies to periodic functions, not real sounds. Obviously by allowing the amplitude coefficients to vary with time, any signal can be written as a sum of sinusoids, for example trivially as f(t)*cos(0*t). Getting into what it means to have slowly varying amplitudes is too complicated for here, and too ambiguous. Saying "Any tone is a sum of sine waves of different frequencies and phases, changing in volume over time" is a bit misleading in saying "is". It would be more OK as "can be decomposed into" or "can be described as" or "can be approximated as". Dicklyon (talk) 08:44, 12 December 2008 (UTC)
- I didn't write the Fourier paragraph. I just moved it to the top, which probably caused some confusion. D.L. was right to replace it with something less restrictive. Let's super refine it. I'm assuming we can ignore phase as harmlessly as we can ignore amplitude and time, because each can be brought in as needed in a more detailed description beyond this article without making this statement false. In other words, a sine, shifted in phase, and changing in amplitude over time, is still a sine. How about this: "A complex tone is composed of sine waves of different frequencies." -- Another Stickler (talk) 21:13, 12 December 2008 (UTC)
- I object to "is composed of", which suggests something much too strong and real when it's in fact not true; I would be OK with "can be approximated as" or "can be analyzed as" or "can be modeled as" or "is conceptualized as" or something like that. Dicklyon (talk) 22:44, 12 December 2008 (UTC)
- I see now that the Fourier thing came in in 2006 with this edit. Sorry for the confusion, and thanks for helping. Dicklyon (talk) 22:49, 12 December 2008 (UTC)
- Thank all of us. I wonder if readers appreciate how much work can go into building one correct sentence. I based "is composed of" on your "can be decomposed into", reversed into the non-passive voice. It also works with the next sentence that uses the adjective "composite". I think "is composed of" is at least as "true" as any other geometric or mathematical statement describing the situation can be, as well as being mainstream, and therefore defensible for the article text. Why do you say it's "in fact not true"? -- Another Stickler (talk) 01:12, 13 December 2008 (UTC)
Not sure what "mainstream" has to do with it... Those sinusoid decomposition products are useful for some analytical purposes, but it's just as valid to call them "artifacts of one of many possible ways to describe the signal." They aren't inherent in the signal, but appear when someone goes looking for them with certain tools.
The active voice is a fine thing to use in descriptive writing, but I'm not sure that means we need to get rid of the passive voice altogether. Here especially, I believe the specialized use of "decompose" in an analytic context is not reciprocal with "compose" as it is commonly used.
Of the choices offered a few paragraphs earlier, I favor "can be modeled as" over the others. I hope most readers will understand that the modeling may more or less accurate, but still understand that "the map is not the territory." __Just plain Bill (talk) 04:32, 13 December 2008 (UTC)
- The excellent new book Music, Thought, and Feeling: The Psychology of Music, by William Forde Thompson, uses "can be described as", that is, "Any naturally occurring sound, ..., can be described as a combination of many simple periodic waves (i.e., sine waves) or partials, each with its own frequency of vibration, amplitude, and phase." That's a "mainstream" view, and is not really true, but it's a language that I wouldn't object much to. Of course, "naturally occurring" is irrelevant, and doesn't make it more true or less true. Dicklyon (talk) 16:21, 13 December 2008 (UTC)
- I think both of you are still stuck on approximation. An approximation is not true in that it leaves parts out. When you accept the whole continuous unbroken frequency spectrum, and not a subset like a Fourier series, it is a complete and sufficient description to say every signal is composed of sines; it doesn't leave any parts out, it's not an approximation, and therefore as "really true" as any statement can be. J.p.B., I said composed not compose, as a whole is composed of parts. The map is not the territory, but all perception and all language is mapping, including science, music theory, and wikipedia articles, so that is not a reason not to rely on our best maps and even call them "true". As far as mainstream, that's a combination of the wiki-tenets of neutrality, verifiability, and no-original-research-ity. Thinking of a wave as a spectrum of frequencies is the first tool of analysis. Graphs of time vs frequency are common in the literature. The model is definitely mainstream. I know no other model. Do you? D.L., thanks for the research; the Thompson sentence exactly parallels the sentence in question, and it's verifiable. I've put it in. Please check that I did the citation right. It kind of screws up the flow a little, in that it defines partial too early and as a secondary subject in the sentence, it says partials in plural, and italicizes it, so it makes the next sentence look redundant, but the next sentence should not be removed because it's important to define "partial" in it's own sentence. I'm satisfied. Are we all? -- Another Stickler (talk) 18:30, 13 December 2008 (UTC)
- Darn it. I just realized the Thompson quote uses "amplitude" instead of "amplitudes", which makes it not apply to some evolving complex tones. -- Another Stickler (talk) 19:11, 13 December 2008 (UTC)
- The notion of partials is inherently discrete, and the conditions needed to make Fourier analysis work exactly leave out all sounds that have a discrete spectrum, so it's hard to see what kind of Fourier theorem you believe in to justify your outlook. Of course, as I said already, if you let amplitudes change in time it's trivial, but no longer are the partials sinusoids. You can't have it both ways, mathematically, so it's best to just stick to admitting an approximation, or a "description". The previous statement relying on the Fourier theorem was at least true for periodic sounds, but making something periodic out of a bit of music is itself a way to approximate that piece of music, no? The "mainstream" view in the not-quite-technical music literature is actually just wrong; even Thompson is wrong in places. I'm OK leaving it not mathematically rigorous, but we ought to not pretend that it rigorous, either. I toned down a few things that I felt were stated too strongly, including the bit about "harmonics" being "technically incorrect" when the partials are not perfect. That would need a source. And I took out timbre since it hadn't been explained how it stood for a sound and I don't like the implicit assumption that it corresponds to spectrum; looks like I still have some work to do on that... Dicklyon (talk) 00:31, 14 December 2008 (UTC)
- OK, I did some more work, broke up the long lead into what looked like intended paragraphs (had single returns in places instead of double), and then broke off a long example section and put it after the terminology, and some more edits. I apologize that I did more at once than you can easily follow by looking at the diff, but I hope you will all find it OK, or work to improve it further. There is still a lot of redundancy, like about the musical intervals at the end of the new section and the start of the next section. Dicklyon (talk) 01:01, 14 December 2008 (UTC)
- I also took out the bit about our ears responding logarithmically, since I'm an ear guy and it's not really true, and has little to do with why musical intervals are consonant or dissonant or sound alike when transposed. Dicklyon (talk) 01:06, 14 December 2008 (UTC)
- I'll be letting this particular point sit for a while, and taking some time to look over the whole article's big picture. I don't see the original issue of this section as too badly broken at the moment, so no rush to fix it. Stickler, I understand how you meant composed (of parts) but I still don't think of that and decomposed (into analytical elements) as exactly reciprocal cognitive items. __Just plain Bill (talk) 06:39, 14 December 2008 (UTC)
- D.L., if you think I'm proposing Fourier it in any way, let alone defending it, you haven't understood what I've written. I'll say it once again: I'm talking about the whole, complete, unbroken, continuous, frequency spectrum. Here's an analogy: if the frequency spectrum is like the positive domain of the real number line, then a Fourier series is like a series of equidistant points on that line. The problem with Fourier is the gaps between its points. It's an incomplete model. -- Another Stickler (talk) 17:19, 14 December 2008 (UTC)
- But the whole complete spectrum is also not a useful concept. I presume you mean the Fourier transform, or maybe the power spectral density. But neither of these is applicable to all sounds (Fourier transform applies to finite-energy sounds, but not sounds that last forever, such as periodic ones; power spectral density applies only to stationary processes), and neither leads to a decomposition that can be called partials, so it's really unclear what you have in mind in terms of connecting spectrum to partials. Dicklyon (talk) 06:11, 15 December 2008 (UTC)
- D.L., you said "if you let amplitudes change in time it's trivial, but no longer are the partials sinusoids." I don't see a mathematical problem with calling them sines with changing amplitude. That explains that the shape will change slightly as the amplitude changes. It shows that two signals are present, the sine and the amplitude/time curve. It doesn't confuse things in any way because the sine is still present in the mix. -- Another Stickler (talk) 17:29, 14 December 2008 (UTC)
- The trivial example Dick gave was a time-varying amplitude function applied to a sinusoid of frequency zero, or cos(0), which amounts to DC. Then the frequency components of the modulating signal become an issue... __Just plain Bill (talk) 19:42, 14 December 2008 (UTC)
- D.L., you said "if you let amplitudes change in time it's trivial, but no longer are the partials sinusoids." I don't see a mathematical problem with calling them sines with changing amplitude. That explains that the shape will change slightly as the amplitude changes. It shows that two signals are present, the sine and the amplitude/time curve. It doesn't confuse things in any way because the sine is still present in the mix. -- Another Stickler (talk) 17:29, 14 December 2008 (UTC)
- It actually does makes some sense to think of sounds as being decomposed into modulated sinusoids, but you also need frequency modulation to get a sensible decomposition, for cases such as vibrato. It's considerably more complicated though to put constraints on the modulating functions, since there are trivial solutions and an infinity of alternative decompositions. So as a way to define partials it's not altogether helpful. Dicklyon (talk) 06:03, 15 December 2008 (UTC)
- Do the frequency relationships between fundamental and partials change during vibrato? I suppose they might, depending on the instrument. Of course, in the case that they don't, there's no need to redefine the partials, as they track the fundamental. In the case that they do, then there are two ways to think of it, either as the partials retaining their identity while being modified, or as different partials. I think the latter is consistent with the definitions we already have, where each partial's identity is precisely its relation to the fundamental, but I don't remember this question even coming up, let alone being written about to cite. -- Another Stickler (talk) 07:49, 15 December 2008 (UTC)
- The whole complete frequency spectrum is not only useful, it's essential in that it holds all possible partials. No Fourier series can do that. -- Another Stickler (talk) 07:59, 15 December 2008 (UTC)
Strict inference and sourcing
[edit]I'm a stickler myself on statements like "although the partials are not harmonics by the strict meaning of the word if they have any inharmonicity at all." This is an inference from a strict interpretation, and unless it is sourced it is either an editor's opinion or original research. I don't see how it adds anything to the statement before, which is that partials are commonly called harmonics even there's a bit of inharmonicity. The reader can be left to deal with the implied bending of the definitions, or to do further research on how strictly the terms are used in various communities; if we want to tell him, we better find out, verifiably, before doing so. Dicklyon (talk) 01:18, 14 December 2008 (UTC)
- It's the "Terminology" section. That's why it's important to make extra sure the meanings are not accidentally blurred. I'll tone it down, but the point still must be made, harmonics are a special case of partials. -- Another Stickler (talk) 01:50, 14 December 2008 (UTC)
- But you linked a dictionary that says in music a harmonic is any overtone. We need better sources if we want to make stricter statements. Dicklyon (talk) 02:19, 14 December 2008 (UTC)
harmonic
[edit]D.L., why did you remove the harmonic citation [1]? The majority of dictionaries listed there have at least one sense of the word that exactly corresponds to the sense in the sentence I placed the citation after, the sense that harmonics are integer multiples of the fundamental, which is why we need to be sure to distinguish from partials, of which they are a subset. -- Another Stickler (talk) 02:08, 14 December 2008 (UTC)
- I removed as I said in the edit summary because the definition that it list in the music field does not agree with what is stated in the article. Also, it was not a reference, but an in-line external link. If you want to put it back as a citation, it would be good to quote both the physics and music definitions and maybe modify the text to suit. Even better, seek a music book to cite, since dictionaries are actually pretty poor sources. GBS finds no sources that mention the notion of an "ideal partial", so that should probably be reworded. Dicklyon (talk) 02:15, 14 December 2008 (UTC)
- Here's a book in which harmonics follow from the approximation of a sound as "essentially periodic". This seems appropriate, and is quite distinct from what you seem to have in mind, which is the notion that partials can be found rather exactly, and then compared with an ideal to see if they are harmonics. There's no theory I know of by which the latter makes much sense, though one can estimate inharmonicity of a partial in some cases. Dicklyon (talk) 02:27, 14 December 2008 (UTC)
- You didn't read all the dictionaries did you? You stopped at Random House Unabridged Dictionary that says in music harmonics are a synonym with overtones, which is clearly wrong. You have to read all of them, then you'll see that the majority do define it as we understand it, as an integer multiple of the fundamental. That's not saying actual instruments are capable of producing exact integer multiples. Partials can be harmonic or inharmonic. There's nothing wrong with an in-line link. If you want to improve it into a citation, do so, but don't remove the inline link until you do. Don't destroy information. -- Another Stickler (talk) 16:42, 14 December 2008 (UTC)
- The only dictionary entry I read was the one you cited. Dicklyon (talk) 06:07, 15 December 2008 (UTC)
- I thought so. Wait for it to load and use the scroll bar. There are six dictionaries if I remember right. No wonder you thought it was a bad citation. Try again, then put the citation back in. -- Another Stickler (talk) 07:08, 15 December 2008 (UTC)
Inharmonicity
[edit]Looking for a source, I found this one that makes it clear that inharmonicity is a property of an individual partial, not of a tone. There may be other definitions, so need to look more before changing this in the article. Dicklyon (talk) 02:13, 14 December 2008 (UTC)
- That's my understanding too. Inharmonicity refers to individual partials, not tones. -- Another Stickler (talk) 16:56, 14 December 2008 (UTC)
Definite pitch and other changes
[edit]Bill, it seems counterproductive to link pitch to definite pitch instead of to pitch (music), since the former is an unsourced stub. I'll work on merging it into the latter, and I'll put the link back as it was.
Other changes of yours and stuff nearby that I might modify (I'm writing all this offline on a plane while looking at your diff I saved, hence the future tense for edits):
- "without apparent waves traveling along it" is not as good as "the wave ... oscillates to and fro but does not appear to travel along it." That latter is more descriptive, since in fact there are waves moving in both directions, and that makes it appear to not travel. It still has apparent waves, so "without apparent waves traveling" sounds misleading. Can you think of a better way to put it? I'll probably just put the old phrasing back.
- the "spacing of resonances" is a concept we might want to modify or clarify, since it is easy to get confused here between the string resonances and the body resonances (formants) if one gets that in mind. I'll think about how to improve it.
- "The musical pitch of a note is usually perceived as the lowest partial present, which may be the one created by vibration over the full length of the string or air column, or a higher harmonic chosen by the player" makes me question whether "usually" is what we want here. It's probably true, but also tends to point away from the possibility of a missing fundamental, which may not be usual at the instrument, but would be usual if listing over a telephone, for example. Maybe "often" would leave it more open. Also, in brasses and woodwinds, it would seem important to note that the excitation rate from the lips or the reed is what directly determines the pitch; it does certainly tend to sync up pretty nearly with one of the harmonic resonances, as "chosen by the player". What might we say here that would make this stuff more clear? Or is it good enough? Consult some sources?
- Inharmonicity; most books I've looked at in GBS talk about it per partial, but at least one talked about inharmonicity of a complex tone; I guess we better mention both. Thompson, which I have with me and was quoting in the middle of the jet-lagged night, doesn't have "inharmonicity" in the index, but has "inharmonic", and says "naturally produced sounds typically include inharmonic components" (p.47); I'm not sure what he has in mind there, in the middle of his discussion of periodic tones. And (p.59), section on Timbre, "In naturally occurring sounds, inharmonic partials (i.e., partials with frequency equal to a non-integer multiple of the fundamental) also affect timbre. When inharmonic partial are removed from a piano note, for example, the note sounds artificial and unfamiliar." So I guess a piano is "natural".
- The idea that what's important about a harmonic series is that all the frequencies are integer multiples of a fundamental is hard to connect with hearing, especially with the notion that frequencies are measured on a log scale, which would make the integer relationship completely non-obvious. It would be more sensible, but pretty much mathematically equivalent, to say that a harmonic series is a set of frequencies that share a common period (that is, all partials being periodic at the period corresponding to the pitch). Thinking about it this way helps to clear up a lot of mysteries about consonance, harmonic intervals, etc. The repetition interval is something that's easy to extract and represent in neural circuits, as J. C. R. Licklider showed in 1951, and these representations explain essentially all pitch phenomena, consonance and dissonance, harmony, etc. It takes the musical harmonic series out of the realm of numerology and puts it into the realm of neuroscience. Why don't we mention something about that? Probably because musicians don't. Malcolm Slaney and I wrote a chapter back in '93, "On the Importance of Time – A Temporal Representation of Sound," which covered some of this stuff, like illustrating the pitch of the strike note of an inharmonic chime. Others, like Roy D. Patterson, and Alain de Cheveigne, and John R. Pierce, and Egbert de Boer, have written about aspects of this approach. But these works have mostly not been music focused, and have not focused on the "harmonic series", so may be hard to pull relevant nuggets from for this article. It strikes me in reading Thompson that several of things he sees as quite mysterious are so easy to understand in this temporal domain. When I get back to my office, I'll have to check Perry Cook's 2001 book Music, Cognition, and Computerized Sound: An Introduction to Psychoacoustics, which has relevant chapters by John Pierce and Max Mathews, and see if it has some nuggets we can use.
Anyway, I'm back now but pooped. Maybe more later. Dicklyon (talk) 06:06, 15 December 2008 (UTC)
- Be cautious about putting in original research. The article isn't about hearing or neuroscience. I'm sure there are perfectly good places to put that in other articles. Let's stick to the harmonic definition as integer multiples of the fundamental, as in my citation you deleted without reading. I don't see anything wrong with adding that they share a period. It is equivalent and may give some readers a quicker grasp. -- Another Stickler (talk) 07:25, 15 December 2008 (UTC)
- I've adjusted the language about standing waves; the previous "to and fro" was not clear to me whether it meant longitudinal travel or transverse motion (in the case of a string.) For now, I'd like to leave the links to Definite pitch since they are more relevant to the context where they stand. The first sentence of the second lead paragraph has a link to Pitch (music) where it applies, so that info is only a click away for the reader.
- Not sure yet how to word the stuff around missing fundamental etc. I have read that low notes on a violin do indeed have an attenuated fundamental at the instrument... I was more concerned with the "lowest possible" seeming to exclude the possibility of overblown notes, or string flageolets, or higher-frequency excitation of brasses. __Just plain Bill (talk) 15:27, 15 December 2008 (UTC)
Table's fouth inaccurate
[edit]Excuse me if I'm not filling this form with correct standards, but I'm new to this. My understanding of the harmonic series is that the pattern 1/2, 1/3, and 1/4 makes a statement regarding fourths and fifths relationship with octaves. That being said I didn't understand how a fifth that was only 2 cents off could translate into a fourth that was 29 cents off. So I did the math and personally found the equal temperament fourth to in fact be 2 cents sharp. Am I simply misunderstanding the table? 24.21.9.176 (talk) 22:03, 23 May 2009 (UTC)
According to the table, the 21st harmonic is a fourth (in some octave). Within the same octave the ratio comes to 21/16. In equal temperament 12*log(21/16)/log(2)= 4.71 or 29 cents flat of 5 semitones. The table seems correct. −Woodstone (talk) 22:57, 23 May 2009 (UTC)
- fast response, I'll remember to check back more consistently in the future! I've always understood the fourth and fifth as summing to an octave. I've read in numerous places that the ratio 3:2 is equivalent to the perfect fifth. Since the 1/3 that makes the 3:2 splits an octave, I was explained that you could conclude that 4:3 was a fourth since it and a perfect fifth make the octave. If you plug that into the equation you showed you get 4.98, or 2 cents flat of 5 ET semitones. The chart doesn't list that as one of the ratios however...I guess I'll reread the article, but in case you answer swiftly again where's the source for those specific intervals?24.21.9.176 (talk) 21:40, 30 May 2009 (UTC)
Your reasoning is correct, but this article does not talk about compounding intervals. It just shows the interval between a tone and its multiples (reduced to within an octave). Since you see in the table that the fifth is 2 cents sharp, you could have concluded immediately that the fourth that completes the octave must be 2 cents flat. For more on compound intervals you might look at just intonation. −Woodstone (talk) 22:52, 30 May 2009 (UTC)
- I think this points at a general problem with this section. It seems to imply that, for example, the occurrence of the fourth in Western music has something to do with its relationship to the 21st harmonic, and that it is only the use of equal temperament that makes this inexact (The Western chromatic scale has been modified into twelve equal semitones, which is slightly out of tune with many of the harmonics). In fact the fourth corresponds to 4:3 in just intonation, and has nothing to do with the 21st harmonic. The same is true of most of the other intervals. 68.239.116.212 (talk) 02:27, 1 December 2009 (UTC)
"Combination tones"
[edit]For example, a perfect fifth, say 200 and 300 Hz (cycles per second), produces a combination tone of 100 Hz (the difference between 300 Hz and 200 Hz); that is, an octave below the lower (actual sounding) note. This 100 Hz first order combination tone then interacts with both notes of the interval to produce second order combination tones of 200 (300-100) and 100 (200-100) Hz and, of course, all further nth order combination tones are all the same, being formed from various subtraction of 100, 200, and 300.
I'm not sure what this is intended to mean. Is it supposed to describe some psychoacoustic effect? Certainly no such tones are actually produced (unless the sound is passed through some sort of nonlinearity). 68.239.116.212 (talk) 02:33, 1 December 2009 (UTC)
- I have no idea what that paragraph is trying to say, either. But there really are physical combination tones produced by nonlinearities in the normally functioning cochlea, as well as psychophysical effects even with binaural presentation of two tones. See combination tone. Tartini noticed that you could use these to tune a fifth to an appropriate temperament, since you can hear beats between the f2-f1 and the 2f1-f2 combination tones. For example, with 200 Hz and 301 Hz, the f2-f1 is 101 Hz and the 2f1-f2 is 99 Hz, so you hear a 2 Hz beat. The beat is slow enough that you can rely on it to get a particular tuning of the fifthen when tuning a piano or organ (see this page for example. Dicklyon (talk) 07:10, 1 December 2009 (UTC)
Additional citations/ref improve
[edit]Why, what, where, and how does this article need additional citations for verification? Hyacinth (talk) 03:29, 18 October 2010 (UTC)
- Tag removed. Hyacinth (talk) 17:17, 15 July 2011 (UTC)
Timbre of musical instruments
[edit]Why and where does this section need additional citations for verification? What references does it need and how should they be added? Hyacinth (talk) 00:22, 30 January 2012 (UTC)
Table again
[edit]the A-natural above C is a Major Sixth not a Minor Sixth. I don't know how to edit this table but it seems inaccurate. — Preceding unsigned comment added by Shastymax (talk • contribs) 02:30, 23 July 2016 (UTC)
- The 13th harmonic is close to a neutral sixth, half way between minor and major sixths, but closer to minor (41 cents off). Is the note name wrong in the table? No, it says A flat. Dicklyon (talk) 02:49, 23 July 2016 (UTC)
- Yes, in the table is a wrong note name. The mathematically reliable name must be A-natural with a flattering by tridecimal comma. Shastymax is right, because the talking is about the tridecimal version of Major Sixth and the table requires correction. --93.76.26.31 (talk) 19:26, 3 August 2016 (UTC)
"Musical notation" section
[edit]The text in question
|
|---|
|
Musical notation There are many systems of musical notation in the world today and throughout history. Our system of treble and bass clef notation, as well as actual pitch information is the result of much compromise and approximation. Our common musical notation and tunings do not match up exactly with the natural harmonic series. No musical notation systems ever have. The following section tries to explain differentiation between common "Western" pitch systems and the more natural, ancient Greek Pythagorean system using symbolic graphic symbols. This is a lot of work to go through for just this one musical notation style, but somebody must have thought it was worth it. (The following is poorly translated from Ukrainian. Don't worry that even the first sentence makes no sense, there's plenty more to come. The math relationships in sound and acoustics are not nearly as complicated and dense as this article seems to project. It's not you, it's the translation.) In the first approximation (without enharmonic, i.e. microtonal, amendments) may be considered as most faithful, for example, a musical notation by Kathleen Schlesinger:[1] Harmonic Series in C Essence is that evaluation of any musical scale, and especially natural, is best to do through comparison with Pythagorean pitches.[2] Such comparison reveals in the set of first 16 overtones a fragment of Pythagorean chainlet with 2 perfect fifths,[3] formed by three overtones with numbers 4, 6, 9; and a subset of the overtones of Pythagorean pitches that contains not only listed overtones, but also those from octave chainlets where these listed are found. This fact evidently demonstrates the relation on the set of matrix structure that reflects the pitches of musical example involving literal notation of Helmholtz where by annexes (from Greek: Πυθαγόρας) are marked Pythagorean notes and by arrows ― chainlet of perfect fifths::
A subset of non-Pythagorean overtones remains after removing Pythagorean subset from the set of all overtones of series:
If non-Pythagorean pitches are compared with appropriate Pythagorean, the first are distinguished from second on small intervals, called commas, among diversity of which is one of the very famous ― syntonic comma by Didymus [4] (further designation ― from Greek: Δίδυμος ― for sharping and inverted ― ― for flattering):
Because the non-Pythagorean pitches may be obtained from the Pythagorean through flattering of latest on , they need be noted as . Really:
For the just notation of pitches is needed another comma, known as septimal comma by Archytas [5] (further designation ― from Greek: Αρχύτας ― for sharping and inverted ― ― for flattering):
By using the prefixes of flattering on of Pythagorean pitches notation for just intonation gets the form , the truthfulness of which is easy to check:
The pitch require undecimal comma by al-Farabi [6] (позначення ― from Greek: αλ-Φαράμπι ― for sharping and inverted ― ― for flattering):
Prefix of sharping leads Pythagorean notation to form , which corresponds the just intonation :
One else pitch is required tridecimal comma [7] (designation ― from Greek: δεκατρία ― for sharping and inverted ― ― for flattering):
Sharping on gives , meaning just intonation :
Must be accounted that the duality of existence multiplicity harmonious [8] and subharmonious,[9] as well as intervals and tones duality,[10][11] was reflected in the dual numbering of pitches (via a slanted, rarer simple, fractional line) of just intonation system. Before (over) the line are written pitch numbers in an overtone series, and after (under) line ― undertone number from which this series was built.[12] Overtones of musical example by Kathleen Schlesinger are natural numbered, but harmonic series is subsystem of just intonation. Identical renumbering in a dual manner, with the 1 after line clearly express that the whole series was built from the 1st undertone (coinciding with the 1st overtone) and each number before the line indicates its membership namely in overtone series from common fundamental, i.e. from the 1st undertone in a series those from this common fundamental. Thus if for full definiteness add else the designation of absence of any comma (from Greek: χωρίς), the set of first 16 overtones has the form:
Combining with musical example shows that literal names are fully responsible to it. Therefore, the notation by Kathleen Schlesinger stands out from other known as the most faithful for use to it enharmonic fictas (in this example they are above notes) that prescribe all necessary microtonal pitch bends to reach just intonation. Harmonic Series in C
Paying attention to the fact of presence in each enharmonic ficta the symbol indicating Pythagorean level of pitch, should be remembered that a bend to the pitch of just intonation a standard tempered pitch, for example, is necessary at first to perform to Pythagorean level, and then whether leave so, if no comma-prefix or is without-comma prefix, either perform from Pythagorean level else bend, by comma-prefix specified. Character above of pitch bind its Pythagorean level with standard tuning frequency,[13] what expresses equality:
References
|
The above section has been added, reverted, and re-added several times. (See WP:BRD.) If anyone wants to copyedit it into something that belongs in the main space of this article, be my guest. Just plain Bill (talk) 22:59, 16 August 2016 (UTC)
- No guests and hosts in the article. There is equality for all. The section has been removed contrary to the mandatory rule for all WP: NOTCENSORED and therefore unreservedly restored. Now is possible to discuss its shortcomings and make improvements. --93.76.26.88 (talk) 16:18, 19 August 2016 (UTC)
- "Be my guest" is an English idiom for "feel free" [to fix the text in question, in this case.] Removing this kind of content is not censorship, but is normal editing. This text needs some serious sandbox work before it will be suitable for inclusion in the article. Just plain Bill (talk) 00:12, 20 August 2016 (UTC)
- Discussion of potentially objectionable content should usually focus not on its potential offensiveness but on whether it is an appropriate image, text, or link. Beyond that, "being objectionable" is generally not sufficient grounds for the removal. Unreservedly restored. Which namely image, text, or link is not appropriate and why? --93.76.28.131 (talk) 20:47, 21 August 2016 (UTC)
- "WP is not censored" is irrelevant here. I don't see anybody saying there are offensive images or text. Have a look at WP:NOTTEXTBOOK instead.
- Discussion of potentially objectionable content should usually focus not on its potential offensiveness but on whether it is an appropriate image, text, or link. Beyond that, "being objectionable" is generally not sufficient grounds for the removal. Unreservedly restored. Which namely image, text, or link is not appropriate and why? --93.76.28.131 (talk) 20:47, 21 August 2016 (UTC)
- "Be my guest" is an English idiom for "feel free" [to fix the text in question, in this case.] Removing this kind of content is not censorship, but is normal editing. This text needs some serious sandbox work before it will be suitable for inclusion in the article. Just plain Bill (talk) 00:12, 20 August 2016 (UTC)
- Most importantly, this article is not the place to explain at great length, with illustrations in set notation, how Pythagorean tuning fits (or does not fit) with scales written in Western notation. The section on "Harmonics and tuning" already explains the relation between the harmonic series and equal-tempered notes.
- The issues of iffy translation, use of unencyclopedic first- and second-person language, and clumsy formatting (e.g. the mix of unreadably small superscripts and overly large low-resolution images) are all secondary to the question of whether this material belongs in an article on the harmonic series in music, or whether it is excessive detail explaining a tangential topic. Just plain Bill (talk) 13:32, 22 August 2016 (UTC)
- The section "Harmonics and tuning" is written without the support of a sufficient number of different sources, what led it to the spread of goofy table with a lot of errors even in the names of basic intervals. Therefore, the section giving a rigorous and highly visual (for familiar with arithmetic and pre-algebra schoolchildren) evidence of the necessity to rely on primary Schlesinger notation for error-free preparation so simple tables, is restored. If you and Woodstone do not like it, then vs. those whom it is not offended is only 0.(571,428)% of you together with him. So stop your censor habits with demands to beg your permission for the restoration of a good cognitive material. --93.76.28.238 (talk) 23:53, 7 September 2016 (UTC)
- The issues of iffy translation, use of unencyclopedic first- and second-person language, and clumsy formatting (e.g. the mix of unreadably small superscripts and overly large low-resolution images) are all secondary to the question of whether this material belongs in an article on the harmonic series in music, or whether it is excessive detail explaining a tangential topic. Just plain Bill (talk) 13:32, 22 August 2016 (UTC)
It has never been clarified what the relevance of the section "Musical notation" is for the subject of this article. The section talks mostly about sequences of pythgorean fifths, not about harmonics. That alone—aside from doubts about quality of writing and sourcing—should be enough grounds to eliminate it from the article. It does not make sense to start making small adjustments. The whole is irrelevant. −Woodstone (talk) 15:18, 25 August 2016 (UTC)
- I agree that attempts to polish this section are pointless, since its relevance to the article's subject is scant to nil. I've removed it again. Just plain Bill (talk) 20:32, 30 August 2016 (UTC)
This is not really getting anywhere. The section is clearly unreadable at this point, and likely not relevant anyway, yet what seems to be the same person on 93.76.0.0/20 keeps restoring it without any explanation of its relevance, without sandboxed revisions, and in violation of WP:3RR. If they think the content of the section is important, I suggest to them that they edit it significantly in their sandbox before putting it in the article. In its current state, there is simply no way that it will be helpful to anyone reading the article. It should also be considered, if the section would be more suitable for the page on pythagorean tuning. Regardless of the quality and relevance of the content, large parts of it is original research, and should not be included. For now I will let the section be (since 93.76.0.0/20 keeps restoring it anyway), but I have tagged it as off-topic and original research. If these issues are not resolved soon-ish, it should be removed. SBareSSomErMig (talk) 12:58, 14 September 2016 (UTC)
- You did not say in addition that different IPs is always one person, the verifiable information and for you hardly understandable characters like are original researches. Labels {off topic} and {original research} are removed. --93.76.18.18 (talk) 08:09, 15 September 2016 (UTC)
- Please stop removing these templates. Clearly both the relevancy and the unoriginality of the sections contents is disputed; just take a look at this talk page. You saying that these issues do not exist does not make it so. In stead, the section should be edited as to resolve the issues, or it should be removed altogether. SBareSSomErMig (talk) 21:02, 15 September 2016 (UTC)
RfC Harmonic series (music)
[edit]The following discussion is closed. Please do not modify it. Subsequent comments should be made on the appropriate discussion page. No further edits should be made to this discussion.
Does the current section Musical notation have sufficient relevance and quality to be kept? 10:02, 9 September 2016 (UTC)
- In my opinion, it is blindingly obvious that the section should be removed. I cannot really tell if it is relevant, because I cannot comprehend the text. This is not is for lack of my own ability - the section is simply poorly written. It uses highly specialized notation without explaining its meaning, and the few sentences of discussion between the formulas are grammatically dubious, and either nonsensical or vacuous. It also seems like it is original research if not straight up crackpottery. SBareSSomErMig (talk) 16:34, 11 September 2016 (UTC)
- One can not say that the whole article is written not poorly. But as time goes on, the article are reading, and those who know the subject better than you improve it without removing. Therefore, the section restored, at least in order to make clear why tables with erroneous content should not be left without edits. --93.76.28.126 (talk) 09:22, 13 September 2016 (UTC)
- I am confident that I know both mathematics and music well enough to see that it is complete gibberish. If you want to improve the section "as time goes on", you should do such in a sandbox, not on the actual article. At this point it is simply not suitable. The table you refer to compares to notes in Equal Temperament, and is thus not erroneous. SBareSSomErMig (talk) 10:38, 13 September 2016 (UTC)
- Show rule, which says that the Equal Temperament is authoritative source. Discussion of potentially objectionable content should usually focus not on its potential offensiveness but on whether it is an appropriate image, text, or link. Restored. It was not specified which namely image, text, or link is not appropriate and why? Why, for example is not appropriate Schlesinger notation? --93.76.13.171 (talk) 19:37, 13 September 2016 (UTC)
- You keep linking to "Wikipedia is not censored" as if it were relevant. It is not relevant.
- Crying "censorship!", accusing other editors of not being smart enough to understand, and now wikilawyering with "show rule" is not going to convince other editors that this dense, opaque section has a place in the article. A simple explanation of "Schlesinger notation" might be a step in the right direction. Even better, show reliable secondary sourcing that shows how it is relevant to the subject of this article. Just plain Bill (talk) 21:22, 13 September 2016 (UTC)
- In the section that you censor out again, there are sources more than in any other of them in the article. That is why it has a direct relation to the article and restored. --93.76.28.9 (talk) 02:45, 14 September 2016 (UTC)
- Show rule, which says that the Equal Temperament is authoritative source. Discussion of potentially objectionable content should usually focus not on its potential offensiveness but on whether it is an appropriate image, text, or link. Restored. It was not specified which namely image, text, or link is not appropriate and why? Why, for example is not appropriate Schlesinger notation? --93.76.13.171 (talk) 19:37, 13 September 2016 (UTC)
- I am confident that I know both mathematics and music well enough to see that it is complete gibberish. If you want to improve the section "as time goes on", you should do such in a sandbox, not on the actual article. At this point it is simply not suitable. The table you refer to compares to notes in Equal Temperament, and is thus not erroneous. SBareSSomErMig (talk) 10:38, 13 September 2016 (UTC)
- One can not say that the whole article is written not poorly. But as time goes on, the article are reading, and those who know the subject better than you improve it without removing. Therefore, the section restored, at least in order to make clear why tables with erroneous content should not be left without edits. --93.76.28.126 (talk) 09:22, 13 September 2016 (UTC)
- You calling it "censorship" does not make it so.
- None of your sources offer substantial support:
- One is a 1911 Britannica attribution for a PD image, staff notation of a "harmonic series in C" (which does not address the mismatch between harmonics and the pitches as notated. E.g. the seventh harmonic is notoriously flat with respect to a diatonic scale such as the modern mixolydian, be it justly tuned or equally tempered.)
- Волконский 1998 is a "sky is blue" definition of the "Pythagorean spiral" for which a wikilink would suffice.
- Five references to Coul's list of intervals: more "sky is blue" dictionary definitions, where wikilinks to perfect fifth, syntonic comma, septimal comma, undecimal comma, and tridecimal comma would suffice.
- Some "sky is blue" dictionary definitions from IEC "Electropedia" including "standard tuning frequency" at A440. Really? Again, a wikilink would suffice for that.
- Three references to a work by the estimable Harry Partch: uncontroversial statements about ratios and tones. The last one is typographic trivia about the use of a slash versus a horizontal line when notating numerical ratios.
- In summary, none of those references is essential to support the text you added. They may be numerous, but they are not needed. Among them, if I am not mistaken, there is no reliable source for "Schlesinger notation."
- The section in question looks like unencyclopedic original research, and does not belong here. It is not relevant to the subject of this article. Just plain Bill (talk) 15:53, 14 September 2016 (UTC)
- Section is written in accordance with the for all mandatory rule: In Wikipedia, verifiability means that anyone using the encyclopedia can check that the information comes from a reliable source. Wikipedia does not publish original research. Its content is determined by previously published information rather than the beliefs or experiences of its editors. Even if you're sure something is true, it must be verifiable before you can add it. You should also show the rule, which says: wikilink would suffice and verifiable information is an original research and must be ruthlessly removed. Labels {off topic} and {original research} are removed. --93.76.19.12 (talk) 05:34, 15 September 2016 (UTC)
- The section in question looks like unencyclopedic original research, and does not belong here. It is not relevant to the subject of this article. Just plain Bill (talk) 15:53, 14 September 2016 (UTC)
Providing external links to various comma intervals is not a substantial reference. For example, a hyperlink to the existing Wikipedia article on such a comma serves as an inline explanatory note. See the links in the third bullet item above. Adding such trivial notes as external links does nothing to verify the notability or applicability of the abstruse notation you insist on adding, in opposition to three other editors. I am beginning to think that you do not understand what original research means in a Wikipedia context.
- It will be more good for the common deal, when you'll begin to think how to depict for WP the Schlesinger notes for harmonic series with extension to the 32-th overtone and enharmonic fictas at the top of notes, as sketched in the discussed section. The circle of fifths, even drawed beautifully, remains the rough and unworthy of our time fake of eternal ground of musical spikelets — Pythagorean spiral. Direct your skills to the constructive channel, instead of barratry, non-productive for further collaboration. --93.76.24.139 (talk) 00:32, 16 September 2016 (UTC) --93.76.24.41 (talk) 01:06, 16 September 2016 (UTC)
I am looking around for the appropriate administrators' notice board, in the hope of putting an end to this tendentious episode. I would welcome input from other editors who may be watching this page. Just plain Bill (talk) 20:30, 15 September 2016 (UTC)
- I agree that this needs to stop. The section should obviously not be included in its current form, but at this point its no use trying to remove it, since the same person keeps restoring it from different ips citing "censorship". It would indeed appear that admin intervention is necessary. I also believe that the table in the "Harmonics and tuning" section should be restored to its original form (that is, from before it was changed by the same user). "Augmented fifth" and especially "augmented prime" are needlessly confusing to the reader. We might as well use the simpler "minor sixth" and "minor second", since the table compares harmonics to 12TET, where these intervals are exactly equivalent. SBareSSomErMig (talk) 20:50, 15 September 2016 (UTC)
- Some of the readers wrote earlier for Woodstone: The big mistake is to be sure that our readers are not smarter than you. You have not read? --93.76.18.208 (talk) 00:56, 16 September 2016 (UTC)
- Thanks. I have added this page to the requests for page protection. Just plain Bill (talk) 21:23, 15 September 2016 (UTC)
- I am restoring the tags with a warning that removal by the IP will mean a block. To take away that temptation, I am semi-protecting the article for four days. --NeilN talk to me 22:09, 15 September 2016 (UTC)
- Agree with all tags here, this section is impenetrable, reads like a WP:OR essay ("This fact evidently demonstrates ...") and should not be taking up a full third of the article. It might work if rewritten in plain English and sourced, it could even merit a full, separate page, but as it stands it's not improving the article. --McGeddon (talk) 09:15, 16 September 2016 (UTC)
- Are you also shocked (like SBareSSomErMig) by fact of existence augmented prime between the 16th and 17th overtones? --93.76.24.190 (talk) 09:37, 16 September 2016 (UTC)
- It is a mistake to think the article without discussed section will be better. There is a lot that must be edited in the article and be added to it, taking into account that here somehow believe that wikilink would suffice. Therefore, the whole article should be decorated with tags which you approved. When the article will reach the right not to have the such jewelry, the discussed section in the amount of current state will be lost in a mass of other necessary information. --93.76.24.73 (talk) 05:07, 17 September 2016 (UTC) --93.76.18.95 (talk) 05:55, 17 September 2016 (UTC)
- This section, motivated with a substantial source or two, and with the language adjusted to be accessible to an interested lay reader, might find a suitable place at Pythagorean tuning. There, it might find editors willing to hammer it into readable shape. Just plain Bill (talk) 12:20, 16 September 2016 (UTC)
- It seems you don't know: all tunings, including the Pythagorean arose in connection with the unavoidable existence of the harmonic series. Therefore, must be exactly here located the section with information that to avoid wrong five-line notation of the harmonic series is only possible without forgetting that the notes on the lines in fact Pythagorean. Have you not know that the five-line system originated to be best system for writing exactly the Pythagorean notes? --93.76.24.190 (talk) 17:22, 16 September 2016 (UTC)
- Remove. That section is a travesty of incomprehensible English and original research. As well, the wiki should not take a teaching tone, which appears to be the chosen style. Binksternet (talk) 06:24, 17 September 2016 (UTC)
- Remove. What follows is somewhat detailed to help the (apparently new to Wikipedia) author of the section to understand the relevant Wikipedia guidelines. Sorry, but This section is nearly incomprehensible and not ready for inclusion in Wikipedia. In addition, as covered in detail by Just plain Bill above, none of the cited sources support the notation in question, and are mostly of the WP:SKYISBLUE type; until multiple WP:reliable sources can be found that use this notation, it is WP:original research. If this is the section author's own idea, and has not gained notability as described in WP:Notability guidelines, it does not belong in Wikipedia per Wikipedia:What_Wikipedia_is_not#Wikipedia_is_not_a_publisher_of_original_thought.
- The notation appears to be used to describe musical tuning, rather than the harmonic series itself. If reliable sources can be found that use this notation, a line or two describing the notation could be added to a tuning article, with the appropriate citations, or might even warrant a stand-alone article. If this is the case, I would strongly recommend that the section's author first post a draft to the appropriate article's talk page for some polishing (there are usually lots of editors around to help, even though it might take a while to get a response). Or for a stand-alone article, become a registered user and create a WP:Sandbox draft for feedback (see also Help:My sandbox), and then when a few editors think it is ready, submit it at WP:Articles for creation.--Wikimedes (talk) 04:14, 23 September 2016 (UTC)
When therefore he was condemned by 281 votes more than those given for acquittal <...> and not long afterwards the Athenians felt such remorse that they shut up the training grounds and gymnasia. They banished the other accusers but put Meletus to death therefore he was condemned <...> and not long afterwards <...> They banished the other accusers --93.76.19.184 (talk) 09:31, 23 September 2016 (UTC)
At it again
[edit]"Psychophysical properties" section
| ||
|---|---|---|
 Simultaneous sounding of all overtones of harmonic series forms the so-called klang [1] [2] usually audible as one pitch[3] at the level of fundamental ie the lowest, first partial.[4] The timbre of klang depends on the loudness distribution on the set of all partials and can move sensation of klang pitch on the level of second or third or some other partial in selected cases of close to zero loudness of some partial subsets. Vertical harmonic series or klang as a kind of complex sound,[5] makes active some group of nerve fibers separated by groups of inactive fibres and the active fibers correspond to partials. The properties of of nerve fibers to conduct separately an appropriate for each partial sensation develop, probably before the birth of organism under the influence of tireless sound of mother's heart. The interaction of nerve fibers in the perception partials of several complex sounds have a significant impact on the overall assessment of pleasantness/unpleasantness of the received sensations. The interaction of nerve fibers in the perception partials of several complex sounds have a significant impact on the overall assessment of pleasantness / unpleasantness of the received sensations. In case perception of several klangs no doubt a dependence of feeling the consonance / dissonance from the expressed by rational numbers frequency relations of fundamentals.[6] Important role in perception of klang playing ability of its partials collectively reproduce the sensation of pitch of missing fundamental, or some other partial from such most notable, ie low frequency partial in a given klang.[7] Therefore, the sensation of klang pitch in general is a sensation of exact unison between pitch of taken separately fundamental and collectively expressed pitch of its residue, as the set of all partials of given klang without the fundamental, ie the lowest from partials.
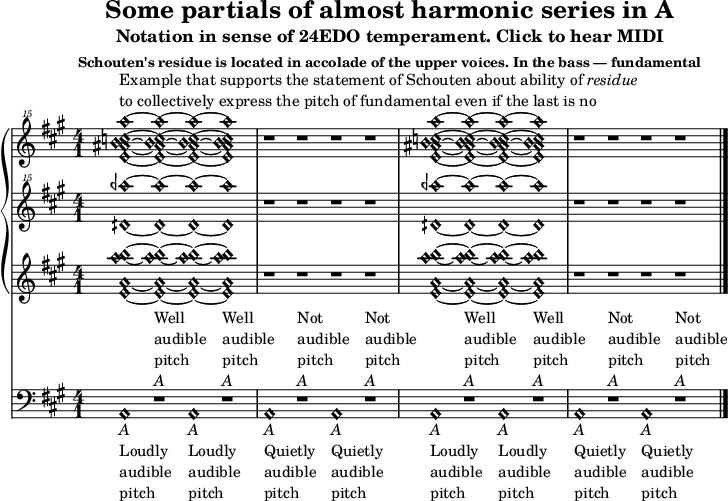

|
The same ip range as before has now created this piece of gibberish. Although it is marginally better sourced than the previous "Musical Notation" section, it is still of very poor quality. The English is near-incomprehensible and the "mathematical" notation is highly non-standard. I have thus taken the liberty of removing it. SBareSSomErMig (talk) 11:48, 12 October 2016 (UTC)
- Elementary operations of set theory can not be "mathematical" notation is highly non-standard. This is a weak excuse to remove the entire section. Another excuse (The English is near-incomprehensible) is also far-fetched. In the section there is a tag for the language experts calling to take part in improving the text. The section has a right to exist in the article and thus was restored. --46.201.181.89 (talk) 12:58, 12 October 2016 (UTC)
- Qouting from the section: "The score clearly show an essence that with involvement of alphabetic notation of Helmholtz looks more clear perhaps as math expression". I don't know what that is supposed to mean, and I don't think a "language expert" would either. The first, and maybe even the second paragraph might be relevant to the article after some some considerable sandbox work (at present it is barely readable and reads like a textbook). The rest of the section is plain nonsense and looks like the same original research that we previously reached consensus for removing. The set expression is a very poor communication of the idea that it tries to convey (indeed, as written, it is completely vacuous). SBareSSomErMig (talk) 13:20, 12 October 2016 (UTC)
- Leave alone the material, for you personally (well and else for such within half percent out of daily readers) not understandable, as it turns out. The harmonic series is a natural system, and the systems are described by language of set theory, which has long and successfully implemented in general educational process. If it frightens you personally, it does not mean that out of 400 readers per day it frightens everyone. Frightening only you signs are recovered with small addition. Let at least 40 different readers will explain here why these inscriptions are so offensive that must be removed. May be then will happens consensus... --93.76.25.155 (talk) 23:36, 12 October 2016 (UTC)
- Personally attacking another editor is not a persuasive tactic. Linking to a google search result is not convincing either. Without a link to a reliable source using this infinitely extended union of sets in an acoustical or music-theoretical context, that section is original research, which does not belong on Wikipedia. Just plain Bill (talk) 00:00, 13 October 2016 (UTC)
- Leave alone the material, for you personally (well and else for such within half percent out of daily readers) not understandable, as it turns out. The harmonic series is a natural system, and the systems are described by language of set theory, which has long and successfully implemented in general educational process. If it frightens you personally, it does not mean that out of 400 readers per day it frightens everyone. Frightening only you signs are recovered with small addition. Let at least 40 different readers will explain here why these inscriptions are so offensive that must be removed. May be then will happens consensus... --93.76.25.155 (talk) 23:36, 12 October 2016 (UTC)
- Qouting from the section: "The score clearly show an essence that with involvement of alphabetic notation of Helmholtz looks more clear perhaps as math expression". I don't know what that is supposed to mean, and I don't think a "language expert" would either. The first, and maybe even the second paragraph might be relevant to the article after some some considerable sandbox work (at present it is barely readable and reads like a textbook). The rest of the section is plain nonsense and looks like the same original research that we previously reached consensus for removing. The set expression is a very poor communication of the idea that it tries to convey (indeed, as written, it is completely vacuous). SBareSSomErMig (talk) 13:20, 12 October 2016 (UTC)
I have removed the section as uninterpretable. Someone who understands the intent can perhaps work with us here to recreate something better. Dicklyon (talk) 02:39, 13 October 2016 (UTC)
Johnston's notation
[edit]
Several of these harmonics do not match their counterparts an octave lower. How can this be correct? For the octaves to match each other:
Burninthruthesky (talk) 09:34, 21 March 2017 (UTC)
External links modified
[edit]Hello fellow Wikipedians,
I have just modified 2 external links on Harmonic series (music). Please take a moment to review my edit. If you have any questions, or need the bot to ignore the links, or the page altogether, please visit this simple FaQ for additional information. I made the following changes:
- Corrected formatting/usage for http://www.itcsra.org/sra_story/sra_story_research/sra_story_resrch_links/sra_story_resrch_pubs/hindustani_music.html
- Added archive https://web.archive.org/web/20050528090732/http://id.mind.net/~zona/mstm/physics/waves/interference/waveInterference2/WaveInterference2.html to http://id.mind.net/~zona/mstm/physics/waves/interference/waveInterference2/WaveInterference2.html
When you have finished reviewing my changes, you may follow the instructions on the template below to fix any issues with the URLs.
This message was posted before February 2018. After February 2018, "External links modified" talk page sections are no longer generated or monitored by InternetArchiveBot. No special action is required regarding these talk page notices, other than regular verification using the archive tool instructions below. Editors have permission to delete these "External links modified" talk page sections if they want to de-clutter talk pages, but see the RfC before doing mass systematic removals. This message is updated dynamically through the template {{source check}} (last update: 5 June 2024).
- If you have discovered URLs which were erroneously considered dead by the bot, you can report them with this tool.
- If you found an error with any archives or the URLs themselves, you can fix them with this tool.
Cheers.—InternetArchiveBot (Report bug) 08:54, 30 October 2017 (UTC)
Definition of "overtone" inaccuracy
[edit]The article's "Terminology" section states that "An overtone is any partial above the lowest partial" This is technically incorrect -- it implies that the lowest overtone is the fundamental, which is untrue. According to this definition, ANY partial (other than the lowest one) is an overtone. I believe it should read, "An overtone is any partial above the FUNDAMENTAL." — Preceding unsigned comment added by 98.109.98.74 (talk) 03:27, 5 July 2018 (UTC)
harmonic singing (music)
[edit]what is harmonic singing 102.89.3.217 (talk) 18:20, 18 January 2022 (UTC)
Speculation about origins of consonance not supported by research
[edit]The section "Harmonics and tuning" currently says, "...small whole-numbered ratios are likely the basis of the consonance of musical intervals." The closest thing it has to a supporting citation is a reference to Hindemith's The Craft of Musical Composition, Book 1, pg. 15. I have that book on hand and I can't find a statement quite like that; the closest he comes that I've found is his statement that "[the ear] hears simple ratios as beautiful and correct sounds" in Ch. 2, Sec. 4, "The Triad." That's not nearly as strong a statement as "basis of consonance," and considering that he basis the whole discssion in that section first and foremost on the actual overtone series I don't think he means to imply that either.
Even if he had said that, though, it would just be his opinion (he doesn't offer any further evidence even for "simple ratios" being "beautiful" except to suggest that the reader go listen for themselves). Research on human subjects has been done which shows that the perception of dissonance between two pure tones is typically greatest when their interval is 25% of the critical band in that area of the spectrum, and the perception of consonance is greatest when their interval is 100% of the critical band. Perceptions of dissonance and consonance between complex pitched sounds is the result of the ratios and loudnesses of their partials on that basis. (See Plomp and Levelt's Tonal Consonance and Critical Bandwidth.) Models for predicting and quantifying the consonance of a set of notes together have been developed from this that work quite well; William Sethares provides such a model (with source code for a computer program) based on Plomp and Levelt in Tuning, Timbre, Spectrum, Scale.
If this kind of material was accounted for in that section it would change the presentation considerably. It's worth remembering that some quite-dissonant intervals correspond to small whole-number ratios (7/4, 9/8) and some intervals that are widely considered more consonant correspond to ratios with larger whole numbers (19/16, 21/16, 25/16, 27/16). However, evaluating their consonance based on critical bands and acoustic beating as described above accounts for this. It's true that many consononant intervals do correspond to small whole-number ratios, and it's not a coincidence, but it doesn't tell the whole story just to say that.
Of course, an in-depth exploration of this topic probably belongs better in Consonance and dissonance, so to some extent there's not much reason to go into it very far here. Given all this, I sort of think that if we're going to talk about tuning in this article, it might make more sense to discuss things like spectralism than just comparing the "harmonic intervals" to 12-TET and briefly mentioning just intonation. Most popular forms of just intonation (e.g. 5-limit) aren't perfectly in-tune with the harmonic series any more than 12-TET is, but there are composers out there who write music explicitly based on the harmonic series, or on one instrument's overtones or the like. I don't think it would hurt to mention the bugle on that basis, speaking of, and it would probably also make sense to take about things like choirs and string ensembles instinctively tuning their chords harmonically. I do find the chart there really useful, but not so much the parts relating to 12-TET; I like the column giving the best-fit diatonic interval, because it helps you get an idea of the sound if you have that background, but those interval names are not specific to 12-TET (they're just diatonic). Mesocarp (talk) 07:34, 29 November 2022 (UTC)




![{\displaystyle \left\{{\begin{matrix}\vdots \\\theta c^{3}[16]&&\vdots \\\theta c^{2}[8]&&\theta g^{2}[12]&&\vdots \\\theta c^{1}[4]&\leftrightarrows &\theta g^{1}[6]&\leftrightarrows &\theta d^{2}[9]\\\theta c[2]&&\theta g[3]\\\theta C[1]\end{matrix}}\right\}\subset \left\{{\begin{matrix}\vdots &&\vdots &&\vdots \\\theta c^{3}[16]\\b^{2}[15]&&b^{2}[15]\\bes^{2}[14]\\a^{2}[13]\\\theta g^{2}[12]&&\theta g^{2}[12]\\f^{2}[11]\\e^{2}[10]\\\theta d^{2}[9]&&\theta d^{2}[9]&&\theta d^{2}[9]\\\theta c^{2}[8]\\bes^{1}[7]&&&\swarrow \nearrow \\\theta g^{1}[6]&&\theta g^{1}[6]\\e^{1}[5]&\swarrow \nearrow \\\theta c^{1}[4]\\\theta g[3]&&\theta g[3]\\\theta c[2]\\\theta C[1]\end{matrix}}\right\}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f124163eeac03e3a07a2dd0a4c26e9fd5d20f12b)
![{\displaystyle \left\{{\begin{matrix}\vdots &\vdots &\vdots \\b^{2}[15]&b^{2}[15]\\bes^{2}[14]&&bes^{2}[14]\\a^{2}[13]\\f^{2}[11]\\e^{2}[10]&e^{2}[10]\\bes^{1}[7]&&bes^{1}[7]\\e^{1}[5]&e^{1}[5]\\\end{matrix}}\right\}=\left\{{\begin{matrix}\vdots &\vdots &\vdots \\\theta c^{3}[16]\\b^{2}[15]&b^{2}[15]\\bes^{2}[14]\\a^{2}[13]\\\theta g^{2}[12]&\theta g^{2}[12]\\f^{2}[11]\\e^{2}[10]\\\theta d^{2}[9]&\theta d^{2}[9]&\theta d^{2}[9]\\\theta c^{2}[8]\\bes^{1}[7]\\\theta g^{1}[6]&\theta g^{1}[6]\\e^{1}[5]\\\theta c^{1}[4]\\\theta g[3]&\theta g[3]\\\theta c[2]\\\theta C[1]\end{matrix}}\right\}\setminus \left\{{\begin{matrix}\vdots \\\theta c^{3}[16]&\vdots \\\theta c^{2}[8]&\theta g^{2}[12]&\vdots \\\theta c^{1}[4]&\theta g^{1}[6]&\theta d^{2}[9]\\\theta c[2]&\theta g[3]\\\theta C[1]\end{matrix}}\right\}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/afba60a3bd9e015a2cc22f4365d5fa5f0302acdd)


![{\displaystyle {\begin{array}{lclcl}\Delta \iota {,}&=&1200\cdot \log _{2}(81/80)&\approx &+21{,}51{\cancel {\mbox{C}}}[{\mbox{Cent}}];\\\iota \Delta {,}&=&1200\cdot \log _{2}(80/81)&\approx &-21{,}51{\cancel {\mbox{C}}}.\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/367c9103a469b8228006c29ee0d59bbbe5393fb4)
![{\displaystyle e^{1}[5],e^{2}[10],b^{2}[15]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/11e175fec4c16b3e6c572b7bc8b6ea1529fe838b)
![{\displaystyle \theta e^{1}[81/16],\theta e^{2}[81/8],\theta b^{2}[243/16]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/735943e65d26572f3f4b9afd5b2d76dd21d2bd6f)
![{\displaystyle \iota \Delta {,}[80/81]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/17251816c3e9a273c8685ac63132ec249c27731e)
![{\displaystyle \iota \Delta {,}\theta e^{1}[5];\iota \Delta {,}\theta e^{2}[10];\iota \Delta {,}\theta b^{2}[15]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/706d1f43767588ff75e7287c871d867d10f89211)
![{\displaystyle {\begin{array}{rcccl}\iota \Delta {,}\theta b^{2}[15]&=&\iota \Delta {,}\theta b^{2}[(80/81)\cdot (243/16)\equiv (80/16)\cdot (243/81)]&=&b^{2}[5\cdot 3\equiv 15];\\\iota \Delta {,}\theta e^{2}[10]&=&\iota \Delta {,}\theta e^{2}[(80/81)\cdot (81/8)\equiv (80/8)\cdot (81/81)]&=&e^{2}[10\cdot 1\equiv 10];\\\iota \Delta {,}\theta e^{1}[5]&=&\iota \Delta {,}\theta e^{1}[(80/81)\cdot (81/16)\equiv (80/16)\cdot (81/81)]&=&e^{1}[5\cdot 1\equiv 5].\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a16d3130a38acb8b5ef3bf4bd5a4337146fbd4dd)
![{\displaystyle bes^{1}[7],bes^{2}[14]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/78600b61d9e5e8714cc0471d7de30740095192f0)



![{\displaystyle \rho {\mbox{A}}{,}[63/64]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0fa6d306505edac42c8e568f0d22bb178a17d1b5)
![{\displaystyle \theta bes^{1}[64/9],\theta bes^{2}[128/9]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/814fd2e52927476198978aa360bf5a5ffcce4949)
![{\displaystyle \rho {\mbox{A}}{,}\theta bes^{1}[7],\rho {\mbox{A}}{,}\theta bes^{2}[14]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/08e4b506713e1aa0de5e14dbc390781efd9209eb)
![{\displaystyle {\begin{array}{rcccl}\rho {\mbox{A}}{,}\theta bes^{2}[14]&=&\rho {\mbox{A}}{,}\theta bes^{2}[(63/64)\cdot (128/9)\equiv (63/9)\cdot (128/64)]&=&bes^{2}[7\cdot 2\equiv 14];\\\rho {\mbox{A}}{,}\theta bes^{1}[7]&=&\rho {\mbox{A}}{,}\theta bes^{1}[(63/64)\cdot (64/9)\equiv (63/9)\cdot (64/64)]&=&bes^{1}[7\cdot 1\equiv 7].\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/75d71cc6ea70e8f1bf0649be0bce49b4ca6601e0)
![{\displaystyle f^{2}[11]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/54e9f7f83191a837cc45193f3f1e7e7587e6c562)



![{\displaystyle \Phi \alpha {,}[33/32]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/14787eddee09c8b240c2dab2245a37b2e96d7f7c)
![{\displaystyle \theta f^{2}[32/3]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b79750560bdc3f8719a8db138354781d19e30a2e)
![{\displaystyle \Phi \alpha {,}\theta f^{2}[11]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7abd2b41c0e2b9f2ca3a7e0b1e349b762949facb)
![{\displaystyle {\begin{array}{rcccl}\Phi \alpha {,}\theta f^{2}[11]&=&\Phi \alpha {,}\theta f^{2}[(33/32)\cdot (32/3)\equiv (33/3)\cdot (32/32)]&=&f^{2}[11\cdot 1\equiv 11].\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9dc7c293c287782f8dcf73ecaccbfab06cc71452)
![{\displaystyle a^{2}[13]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1c8ddbfbabc2c15a521ea6e44dd2dfb90bc71a5e)



![{\displaystyle \theta a^{2}[27/2]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6cd0fea6d36b153159beca12884e4f3fe7704f11)
![{\displaystyle \iota \rho {,}\theta a^{2}[13]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/04f5b8b90048c5cda6028f89d6ecdbb5054aa413)
![{\displaystyle {\begin{array}{rcccl}\iota \rho {,}\theta a^{2}[13]&=&\iota \rho {,}\theta a^{2}[(26/27)\cdot (27/2)\equiv (26/2)\cdot (27/27)]&=&a^{2}[13\cdot 1\equiv 13].\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/73c04801f5a1c4bc2bc4a3da590941f4813ffca6)

![{\displaystyle \left\{{\begin{array}{|c|c|c|c|c|c|c|c|c|c|c|c|c|c|c|}\hline _{\chi {,}\theta }&_{\chi {,}\theta }&_{\chi {,}\theta }&_{\chi {,}\theta }&_{\iota \Delta {,}\theta }&_{\chi {,}\theta }&_{\rho \mathrm {A} {,}\theta }&_{\chi {,}\theta }&_{\chi {,}\theta }&_{\iota \Delta {,}\theta }&_{\Phi \alpha {,}\theta }&_{\chi {,}\theta }&_{\iota \rho {,}\theta }&_{\rho \mathrm {A} {,}\theta }&_{\iota \Delta {,}\theta }&_{\chi {,}\theta }\\^{~~C}_{[1/1]}&^{~~c}_{[2/1]}&^{~~g}_{[3/1]}&^{~~c^{1}}_{[4/1]}&^{~~e^{1}}_{[5/1]}&^{~~g^{1}}_{[6/1]}&^{~bes^{1}}_{[7/1]}&^{~~c^{2}}_{[8/1]}&^{~~d^{2}}_{[9/1]}&^{~~e^{2}}_{[10/1]}&^{~~f^{2}}_{[11/1]}&^{~~g^{2}}_{[12/1]}&^{~~a^{2}}_{[13/1]}&^{~bes^{2}}_{[14/1]}&^{~~b^{2}}_{[15/1]}&^{~~c^{3}}_{[16/1]}&^{\cdots }\\\hline \end{array}}\right\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/85f1f7f0c918ecbb045deddc1013192df95a0af5)


![{\displaystyle {\begin{array}{|c|c|c|}\hline ^{~~C}_{\left[{\frac {1}{1}}\right]}&^{~~c}_{\left[{\frac {2}{1}}\right]}&^{~~g}_{\left[{\frac {3}{1}}\right]}\\\hline \end{array}}~~~~~~~~~{\begin{array}{|c|c|c|c|c|c|c|c|c|c|c|c|}\hline ^{~~c^{1}}_{\left[{\frac {4}{1}}\right]}&^{~~e^{1}}_{\left[{\frac {5}{1}}\right]}&^{~~g^{1}}_{\left[{\frac {6}{1}}\right]}&^{bes^{1}}_{\left[{\frac {7}{1}}\right]}&^{~~c^{2}}_{\left[{\frac {8}{1}}\right]}&^{~~d^{2}}_{\left[{\frac {9}{1}}\right]}&^{~~e^{2}}_{\left[{\frac {10}{1}}\right]}&^{~~f^{2}}_{\left[{\frac {11}{1}}\right]}&^{~~g^{2}}_{\left[{\frac {12}{1}}\right]}&^{~~a^{2}}_{\left[{\frac {13}{1}}\right]}&^{~bes^{2}}_{\left[{\frac {14}{1}}\right]}&^{~~b^{2}}_{\left[{\frac {15}{1}}\right]}&^{~~c^{3}}_{\left[{\frac {16}{1}}\right]}\\\hline \end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/db31f994d1b284539b23d3a67a69141690c565fb)


![{\displaystyle {\begin{array}{rcccl}_{\theta }^{\pitchfork }a^{2}[27/2]-(\theta P8[2/1])&=&_{\theta }^{\pitchfork }a^{(2-1\equiv 1)}[(27/2)\cdot (1/2)\equiv (27/4)]&=&{\pitchfork }a^{1}[27/4][440{\mbox{Hz}}].\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5941dff9fe22773607d51e20fccb5fa0ff08c8ef)

![{\displaystyle \left\{{\begin{matrix}:\\~~~c^{3}{:}[16]\\~~~b^{2}{:}[15]\\bes^{2}{:}[14]\\~~~a^{2}{:}[13]\\~~~g^{2}{:}[12]\\~~~f^{2}{:}[11]\\~~~e^{2}{:}[10]\\~~~d^{2}{:}[9]\\~~~c^{2}{:}[8]\\bes^{1}{:}[7]\\~~~g^{1}{:}[6]\\~~~e^{1}{:}[5]\\~~~c^{1}{:}[4]\\~~~~g{:}[3]\\~~~~c{:}[2]\\~~~~C{:}[1]\\\end{matrix}}\right\}\supseteq \left\{{\begin{matrix}:\\~~~c^{3}{:}[16]\\~~~b^{2}{:}[15]\\bes^{2}{:}[14]\\~~~a^{2}{:}[13]\\~~~g^{2}{:}[12]\\~~~f^{2}{:}[11]\\~~~e^{2}{:}[10]\\~~~d^{2}{:}[9]\\~~~c^{2}{:}[8]\\bes^{1}{:}[7]\\~~~g^{1}{:}[6]\\~~~e^{1}{:}[5]\\~~~c^{1}{:}[4]\\~~~~g{:}[3]\\~~~~c{:}[2]\\~~~~C{:}[1]\\\end{matrix}}\right\}\cup \left\{{\begin{matrix}:\\~~~e^{3}{:}[20]\\~~~d^{3}{:}[18]\\~~~c^{3}{:}[16]\\bes^{2}{:}[14]\\~~~g^{2}{:}[12]\\~~~e^{2}{:}[10]\\~~~c^{2}{:}[8]\\~~~g^{1}{:}[6]\\~~~c^{1}{:}[4]\\~~~~c{:}[2]\\\end{matrix}}\right\}\cup \left\{{\begin{matrix}:\\~~~b^{3}{:}[30]\\~~~a^{3}{:}[27]\\~~~g^{3}{:}[24]\\~~~f^{3}{:}[21]\\~~~d^{3}{:}[18]\\~~~b^{2}{:}[15]\\~~~g^{2}{:}[12]\\~~~d^{2}{:}[9]\\~~~g^{1}{:}[6]\\~~~~g{:}[3]\\\end{matrix}}\right\}\cup \left\{{\begin{matrix}:\\~~~c^{4}{:}[32]\\bes^{3}{:}[28]\\~~~g^{3}{:}[24]\\~~~e^{3}{:}[20]\\~~~c^{3}{:}[16]\\~~~g^{2}{:}[12]\\~~~c^{2}{:}[8]\\~~~c^{1}{:}[4]\\\end{matrix}}\right\}\cup \left\{{\begin{matrix}:\\~~~e^{4}{:}[40]\\~~~d^{4}{:}[35]\\~~~b^{3}{:}[30]\\gis^{3}{:}[25]\\~~~e^{3}{:}[20]\\~~~b^{2}{:}[15]\\~~~e^{2}{:}[10]\\~~~e^{1}{:}[5]\\\end{matrix}}\right\}\cup \left\{{\begin{matrix}:\\~~~f^{4}{:}[42]\\~~~d^{4}{:}[36]\\~~~b^{3}{:}[30]\\~~~g^{3}{:}[24]\\~~~d^{3}{:}[18]\\~~~g^{2}{:}[12]\\~~~g^{1}{:}[6]\\\end{matrix}}\right\}\cup }](https://wikimedia.org/api/rest_v1/media/math/render/svg/747af7a02789a0a496b46cde948ffcafec891683)
![{\displaystyle \cup \left\{{\begin{matrix}:\\~~~f^{4}{:}[42]\\~~~d^{4}{:}[35]\\bes^{3}{:}[28]\\~~~f^{3}{:}[21]\\bes^{2}{:}[14]\\bes^{1}{:}[7]\\\end{matrix}}\right\}\cup \left\{{\begin{matrix}:\\~~~g^{4}{:}[48]\\~~~e^{4}{:}[40]\\~~~c^{4}{:}[32]\\~~~g^{3}{:}[24]\\~~~c^{3}{:}[16]\\~~~c^{2}{:}[8]\\\end{matrix}}\right\}\cup \left\{{\begin{matrix}:\\~~~a^{4}{:}[54]\\fis^{4}{:}[45]\\~~~d^{4}{:}[36]\\~~~a^{3}{:}[27]\\~~~d^{3}{:}[18]\\~~~d^{2}{:}[9]\\\end{matrix}}\right\}\cup \left\{{\begin{matrix}:\\~~~b^{4}{:}[60]\\gis^{4}{:}[50]\\~~~e^{4}{:}[40]\\~~~b^{3}{:}[30]\\~~~e^{3}{:}[20]\\~~~e^{2}{:}[10]\\\end{matrix}}\right\}\cup \cdots }](https://wikimedia.org/api/rest_v1/media/math/render/svg/23920fdff3269a8dd9baf961eaabd88a98c45e98)


