Mathematics desk
< February 21	<< Jan \| February \| Mar >>	February 23 >

Welcome to the Wikipedia Mathematics Reference Desk Archives
The page you are currently viewing is an archive page. While you can leave answers for any questions shown below, please ask new questions on one of the current reference desk pages.

February 22

Different answer to an integral than that given by Mathematica

Ok, so I have this Calculus II homework problem. $\int {\cot ^{2}(2\theta )}d\theta$ . When I solved it it gives me $-{\frac {1}{2}}\left[\cot(2\theta )-2\theta \right]+C$ , but Mathematica's Integrator gives me $-\theta -{\frac {1}{2}}\cot(2\theta )+C$ . Is my solution wrong? If not, How are these two responses equal? I guess I should sign eh?MentalPower 06:34, 22 February 2007 (UTC)[reply]

Clearly they differ, but only by one sign; check your work. I'm amazed that you could do the integral (almost) and not the comparison. --KSmrq^T 10:11, 22 February 2007 (UTC)[reply]

Yes, your answer is wrong. I assume you used the trig identity I'm thinking of, so let's start there.

\int \cot ^{2}(2\theta )\ d\theta =\int \csc ^{2}(2\theta )-1\ d\theta =\int \csc ^{2}(2\theta )\ d\theta -\int 1\ d\theta =-{\frac {1}{2}}\cot(2\theta )-\theta +C.

I hope that's helpful. –King Bee ^{(T • C)} 14:29, 22 February 2007 (UTC)[reply]

I doubt this is the right place to ask the question, but

Has anyone ever considered having laymen's articles on complicated subjects, especially in Math and Computers? Even the fairly noncomplicated things can be quite obtuse. I was just looking at the article on Slope Fields, a pretty straightforward Calculus concept (not that I understand it), and the wiki page didn't really clarify things at all. It just had a lot of very technical writing, and a lot of symbols. I do realize that you're all mathmaticians, and this is how such things are most directly expressed, but most of the people who look at these articles are coming at them with limited info, and they cant understand the complicated and technical jargon on most of the page. This pertains pretty much exclusively to computer and math articles with are really complicated. Science, pop culture, history, literature etc articles seem pretty straightforward, though perhaps that's due to what I know more about. At any rate, the idea would be that you could go to a page like Slope Field and instead of being expected to understand this:

"Given a system of differential equations,

${\frac {du}{dt}}=f(t,u,...y,z)$

$\cdots$

${\frac {dy}{dt}}=j(t,u,...y,z)$

${\frac {dz}{dt}}=k(t,u,...y,z)$

the slope field is an array of slope marks in the phase space (the preceding equations imply seven dimensions, but can be any number depending on the number of relevant variables; for example, two in the case of a first-order linear ODE, as seen to the right). Each slope mark is centered at a point (t,u,...y,z) and is parallel to the vector

${\begin{pmatrix}1\\f(t,u,...y,z)\\\cdots \\j(t,u,...y,z)\\k(t,u,...y,z)\end{pmatrix}}$ ."

you could click on a link to a simplified version that would just explain it in English so you'd have something more tangible to work with before you jumped straight into the math. Just a thought. Didn't know where else it would go.

Clear writing is always helpful, and it doesn't need to be a simplified version of the article for clear writing to be important. Probably the article you describe could use some work. However unlike some other subjects not all topics in mathematics can be explained easily in words of one syllable: "there is no royal road". —David Eppstein 07:15, 22 February 2007 (UTC)[reply]

Wikipedia is an encyclopedia, not an instruction manual. —Preceding unsigned comment added by 203.49.242.20 (talk • contribs) 07:28, 2007 February 22

The plots in question are of little use in seven dimensions, and the idea is much more accessible than the article. Meanwhile, try following the references at the end. Note that we use these to get an intuition about instances of a rather complicated beast called a differential equation. Unless you understand that context, the tool will make little sense.

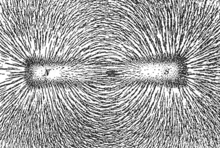
Here's a physical analog. Take a bar magnet, a strip of magnetized iron with a North magnetic pole at one end and a South at the other. Physics claims the magnet creates a magnetic field, the physical embodiment of a differential equation. At every place in that field we can point in one direction as feeling North and the opposite direction as feeling South. If we take a piece of (unmagnetized) iron and shave off a pile of small iron filings, we can demonstrate. Place a very thin sheet of glass or plastic over the bar, and sprinkle the shavings on top. Give it a good shake. The little metal fibers will prefer to line up along the North-South lines they feel. This allows us to "see" the pattern of the magnetic field.

A computer program can do a similar thing numerically, using short line segments in place of iron filings, and using any differential equation in place of the magnetic field. That's the idea of a slope field. Differential equations arise frequently and naturally in many practical disciplines, including physics, biology, economics, and chemistry. Often a plot like this can be enormously helpful, especially if we believe Richard Hamming, who said "The purpose of computing is insight, not numbers." --KSmrq^T 11:18, 22 February 2007 (UTC)[reply]

Lempel-Ziv-Welch compression algorithm example on Wikipedia

Moved to Computing ref desk. Please sign your posts with --~~~~ --TotoBaggins 18:24, 23 February 2007 (UTC)[reply]

mathematical practical applications

Could mathematics calculate the date and place of a cure for cancer or any other biological disease ?

Yes, it can, but the derivation is too long to fit on this margin. – b_jonas 13:16, 23 February 2007 (UTC)[reply]

You seem to be asking if the universe is deterministic. My understanding is that even if in principle you could figure out the exact current state of a system (you can't), the randomness inherent in quantum mechanics would prevent you from making predictions based on that state. However, math does have a few practical applications besides allowing omniscience, so we should probably keep it around. --TotoBaggins 18:30, 23 February 2007 (UTC)[reply]

Since "cancer" is many different diseases, it will be cured over a long time period, perhaps a century. Early portions of the cure have already arrived, such as the new vaccine against HPV, which causes cervical cancer. StuRat 00:20, 27 February 2007 (UTC)[reply]

Size of next prime number

I'm looking for results about the size of prime numbers. Specifically, I want this:

If $p_{n}$ is the $n$ th prime number then for $n>3$ ,

$p_{n+1}<{\sqrt {\prod _{i=1}^{n}p_{i}}}$

This is true, right? If so is this provable using not too much maths? (Not too much = half a page and no mention of Euler)

--87.194.21.177 22:22, 22 February 2007 (UTC)[reply]

Depends on what you mean by "not too much maths". Using Bertrand's postulate, we know that

p_{n-1}\geq p_{n}/2

, so for

n>4

{\sqrt {\prod _{i=1}^{n}p_{i}}}\geq {\sqrt {2\cdot 3\cdot 5\cdot (p_{n}/2)\cdot p_{n}}}=p_{n}{\sqrt {15}}>2p_{n}>p_{n+1},

in the last step using Betrand's postulate again.--80.136.136.113 22:32, 22 February 2007 (UTC)[reply]