User:TentativeTypist/sandbox
Derivative
[edit]
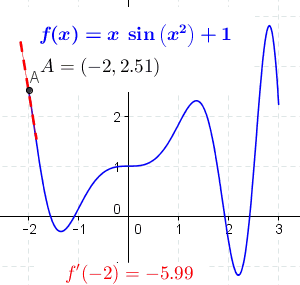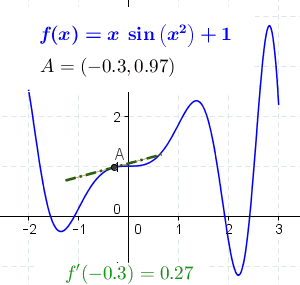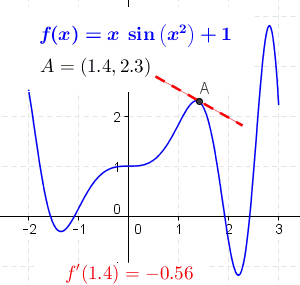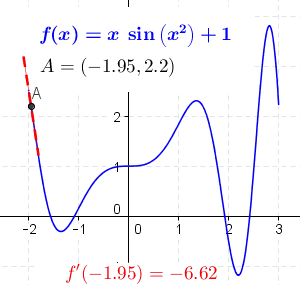
The derivative of at the point , denoted ,[1] is defined as the slope of the tangent to .[2] In order to gain an intuition for this definition, one must first be familiar with finding the slope of a linear equation, written in the form . The slope of an equation is its steepness. It can be found by picking any two points and dividing the change in by the change in , meaning that . As an example, the graph of has a slope of , as shown in the diagram below:

For brevity, is often written as , with being the Greek letter Delta, meaning 'change' or 'increment'.[3] The slope of a linear equation is constant, meaning that the steepness is the same everywhere. However, many graphs, including , vary in their steepness. This means that one can no longer pick any two arbitrary points and compute the slope. Instead, the slope of the graph is defined using a tangent line—a line that 'just touches' a particular point. The slope of a curve at a particular point is defined as the slope of the tangent to that point. For example, has a slope of at , because the slope of the tangent line to that point is equal to :

The derivative of a function is defined as the slope of this tangent line.[Note 1] Even though the tangent line only touches a single point, it can be approximated by a line that goes through two points. This is known as a secant line. If the two points that the secant line goes through are close together, then the secant line closely resembles the tangent line, and, as a result, its slope is also very similar:

The advantage of using a secant line is that its slope can be calculated directly. Consider the two points on the graph and , where is a small number. As before, the slope of the line passing through these two points can be calculated with the formula . This gives
As gets closer and closer to , the slope of the secant line gets closer and closer to the slope of the tangent line. This is formally written as[3]
The above expression means 'as gets closer and closer to 0, the slope of the secant line gets closer and closer to a certain value'. The value that is being approached is the derivative of ; this can be written as .[1][4] If , the derivative can also be written as , with representing an infinitesimal change. For example, represents an infinitesimal change in x.[Note 2] In summary, if , then the derivative of is[3]
provided such a limit exists.[4][Note 3] Differentiating a function using the above definition is known as differentiation from first principles. Here is a proof, using differentiation from first principles, that the derivative of is :
As , . Therefore, . This proof can be generalised to show that , if and are constants. This is known as the power rule. For example, . However, many other functions cannot be differentiated as easily as polynomial functions, meaning that sometimes further techniques are needed to find the derivative of a function. These techniques include the chain rule, product rule, and quotient rule. Other functions cannot be differentiated at all, giving rise to the concept of differentiability.
Derivative
[edit]

The derivative of at the point , denoted ,[1] is defined as the slope of the tangent to .[5] In order to gain an intuition for this definition, one must first be familiar with finding the slope of a linear equation, written in the form . The slope of an equation is its steepness. It can be found by picking any two points and dividing the change in by the change in , meaning that . As an example, the graph of has a slope of , as shown in the diagram below:

For brevity, is often written as , with being the Greek letter Delta, meaning 'change' or 'increment'.[3] The slope of a linear equation is constant, meaning that the steepness is the same everywhere. However, many graphs, including , vary in their steepness. This means that one can no longer pick any two arbitrary points and compute the slope. Instead, the slope of the graph is defined using a tangent line—a line that 'just touches' a particular point. The slope of a curve at a particular point is defined as the slope of the tangent to that point. For example, has a slope of at , because the slope of the tangent line to that point is equal to :

The derivative of a function is defined as the slope of this tangent line.[Note 4] Even though the tangent line only touches a single point, it can be approximated by a line that goes through two points. This is known as a secant line. If the two points that the secant line goes through are close together, then the secant line closely resembles the tangent line, and, as a result, its slope is also very similar:

The advantage of using a secant line is that its slope can be calculated directly. Consider the two points on the graph and , where is a small number. As before, the slope of the line passing through these two points can be calculated with the formula . This gives
As gets closer and closer to , the slope of the secant line gets closer and closer to the slope of the tangent line. This is formally written as[3]
The above expression means 'as gets closer and closer to 0, the slope of the secant line gets closer and closer to a certain value'. The value that is being approached is the derivative of ; this can be written as .[1][4] If , the derivative can also be written as , with representing an infinitesimal change. For example, represents an infinitesimal change in x.[Note 5] In summary, if , then the derivative of is[3]
provided such a limit exists.[4][Note 6] Differentiating a function using the above definition is known as differentiation from first principles. Here is a proof, using differentiation from first principles, that the derivative of is :
As , . Therefore, . This proof can be generalised to show that , if and are constants. This is known as the power rule. For example, . However, many other functions cannot be differentiated as easily as polynomial functions, meaning that sometimes further techniques are needed to find the derivative of a function. These techniques include the chain rule, product rule, and quotient rule. Other functions cannot be differentiated at all, giving rise to the concept of differentiability.
Exponentiation
[edit]Defining exponentiation via logarithms
[edit]The meaning of , where and are positive real numbers, can also come from natural logarithm. This avoids the difficulty surrounding the definition of for irrational . First, we may define, for ,
It can then be proven that satisfies the basic properties of logarithms, in particular . Then, can be defined as the inverse of , and can be defined as the number such that .

Finally, can be defined as . Since , can also be interpreted to mean . In any case, this approach sidesteps the issue surrounding the definition of for irrational ; in fact, has the same definition regardless of whether is a natural number, an integer, a rational number, or a real number.
Small-angle approximation
[edit]Algebraic
[edit]
The Maclaurin series expansions of the main trigonometric functions are
where θ is the angle in radians.
It is readily seen that the second most significant (third-order) term falls off as the cube of the first term; thus, even for a not-so-small argument such as 0.01, the value of the second most significant term is on the order of 0.000001, or 1/10000 the first term. One can thus safely approximate:
By extension, since the cosine of a small angle is very nearly 1, and the tangent is given by the sine divided by the cosine,
- ,
Overview
[edit]Unlike factoring by inspection, completing the square can be used to solve any quadratic equation. Consider the example
In order to isolate for , it helps to consider this problem geometrically. The first term, can be interpreted as the area of square with side length . The second term, can be interpreted as the area of a rectangle with lengths and , or, as the combined area of two rectangles that have lengths and :

This diagram suggests that is almost a perfect square with side length . If we add to both sides of the equation, then it becomes
The left-hand side of the equation can then be written as , and so
Notes
[edit]- ^ Though the technical definition of a function is somewhat involved, it is easy to appreciate what a function is intuitively. A function takes an input and produces an output. For example, the function takes a number and squares it. The number that the function performs an operation on is often represented using the letter , but there is no difference whatsoever between writing and writing . For this reason, is often described as a 'dummy variable'. When doing single-variable calculus, the function and the equation are essentially interchangeable.
- ^ The term infinitesimal can sometimes lead people to wrongly believe there is an 'infinitely small number'—i.e. a positive real number that is smaller than any other real number. In fact, the term 'infinitesimal' is merely a shorthand for a limiting process. For this reason, is not a fraction—rather, it is the limit of a fraction.
- ^ Not every function can be differentiated, hence why the definition only applies if 'the limit exists'. For more information, see the Wikipedia article on differentiability.
- ^ Though the technical definition of a function is somewhat involved, it is easy to appreciate what a function is intuitively. A function takes an input and produces an output. For example, the function takes a number and squares it. The number that the function performs an operation on is often represented using the letter , but there is no difference whatsoever between writing and writing . For this reason, is often described as a 'dummy variable'. When doing single-variable calculus, the function and the equation are essentially interchangeable.
- ^ The term infinitesimal can sometimes lead people to wrongly believe there is an 'infinitely small number'—i.e. a positive real number that is smaller than any other real number. In fact, the term 'infinitesimal' is merely a shorthand for a limiting process. For this reason, is not a fraction—rather, it is the limit of a fraction.
- ^ Not every function can be differentiated, hence why the definition only applies if 'the limit exists'. For more information, see the Wikipedia article on differentiability.
- ^ a b c d "List of Calculus and Analysis Symbols". Math Vault. 2020-05-11. Retrieved 2020-09-17.
- ^ Alcock, Lara (2016). How to Think about Analysis. New York: Oxford University Press. pp. 155–157. ISBN 978-0-19-872353-0.
- ^ a b c d e f "Differential calculus - Encyclopedia of Mathematics". encyclopediaofmath.org. Retrieved 2020-09-17.
- ^ a b c d Weisstein, Eric W. "Derivative". mathworld.wolfram.com. Retrieved 2020-09-17.
- ^ Alcock, Lara (2016). How to Think about Analysis. New York: Oxford University Press. pp. 155–157. ISBN 978-0-19-872353-0.




























































![{\displaystyle {\begin{aligned}\sin \theta &=\sum _{n=0}^{\infty }{\frac {(-1)^{n}}{(2n+1)!}}\theta ^{2n+1}\\&=\theta -{\frac {\theta ^{3}}{3!}}+{\frac {\theta ^{5}}{5!}}-{\frac {\theta ^{7}}{7!}}+\cdots \\[6pt]\cos \theta &=\sum _{n=0}^{\infty }{\frac {(-1)^{n}}{(2n)!}}\theta ^{2n}\\&=1-{\frac {\theta ^{2}}{2!}}+{\frac {\theta ^{4}}{4!}}-{\frac {\theta ^{6}}{6!}}+\cdots \theta \\[6pt]\tan \theta &=\sum _{n=1}^{\infty }{\frac {B_{2n}(-4)^{n}\left(1-4^{n}\right)}{(2n)!}}\theta ^{2n-1}\\&=\theta +{\frac {\theta ^{3}}{3}}+{\frac {2\theta ^{5}}{15}}+{\frac {17\theta ^{7}}{315}}+\cdots \\[6pt]\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/72cdc76b2990f1f29d7304f65001c910785071ae)















