User:Martin Hogbin/FLI section
The law of physics describing the process of electromagnetic induction is known as Faraday's law of induction and the most widespread version of this law states:
The induced electromotive force in any closed circuit is equal to the negative of the time rate of change of the magnetic flux through the circuit.
Faraday's law of induction makes use of the magnetic flux ΦB through a hypothetical surface Σ whose boundary is a wire loop. Since the wire loop may be moving, we write Σ(t) for the surface. The magnetic flux is defined by a surface integral:
where dA is an element of surface area of the moving surface Σ(t), B is the magnetic field, and B·dA is a vector dot product (the infinitesimal amount of magnetic flux). In more visual terms, the magnetic flux through the wire loop is proportional to the number of magnetic flux lines that pass through the loop.
When the flux changes—because B changes, or because the wire loop is moved or deformed, or both—Faraday's law of induction says that the wire loop acquires an EMF, , defined as the energy available from a unit charge that has travelled once around the wire loop.[1][2][3][4] Equivalently, it is the voltage that would be measured by cutting the wire to create an open circuit, and attaching a voltmeter to the leads.
Maxwell–Faraday equation
[edit]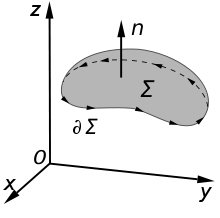
The Maxwell–Faraday equation is a generalisation of Faraday's law that states that a time-varying magnetic field is always accompanied by a spatially-varying, non-conservative electric field, and vice-versa. The Maxwell–Faraday equation is
(in SI units) where is the curl operator and again E(r, t) is the electric field and B(r, t) is the magnetic field. These fields can generally be functions of position r and time t.
The Maxwell–Faraday equation is one of the four Maxwell's equations, and therefore plays a fundamental role in the theory of classical electromagnetism. It can also be written in an integral form by the Kelvin-Stokes theorem:[5]
where, as indicated in the figure:
- Σ is a surface bounded by the closed contour ∂Σ,
- E is the electric field, B is the magnetic field.
- dℓ is an infinitesimal vector element of the contour ∂Σ,
- dA is an infinitesimal vector element of surface Σ. If its direction is orthogonal to that surface patch, the magnitude is the area of an infinitesimal patch of surface.
Both dℓ and dA have a sign ambiguity; to get the correct sign, the right-hand rule is used, as explained in the article Kelvin-Stokes theorem. For a planar surface Σ, a positive path element dℓ of curve ∂Σ is defined by the right-hand rule as one that points with the fingers of the right hand when the thumb points in the direction of the normal n to the surface Σ.
The integral around ∂Σ is called a path integral or line integral.
Original
[edit]The law of physics describing the process of electromagnetic induction is known as Faraday's law of induction and the most widespread version of this law states:
The induced electromotive force in any closed circuit is equal to the negative of the time rate of change of the magnetic flux through the circuit.
This version of Faraday's law strictly holds only when the closed circuit is a loop of infinitely thin wire,[1] and is invalid in some other circumstances. A different version, the Maxwell–Faraday equation (discussed below), is valid in all circumstances.
Faraday's law of induction makes use of the magnetic flux ΦB through a hypothetical surface Σ whose boundary is a wire loop. Since the wire loop may be moving, we write Σ(t) for the surface. The magnetic flux is defined by a surface integral:
where dA is an element of surface area of the moving surface Σ(t), B is the magnetic field, and B·dA is a vector dot product (the infinitesimal amount of magnetic flux). In more visual terms, the magnetic flux through the wire loop is proportional to the number of magnetic flux lines that pass through the loop.
When the flux changes—because B changes, or because the wire loop is moved or deformed, or both—Faraday's law of induction says that the wire loop acquires an EMF, , defined as the energy available from a unit charge that has travelled once around the wire loop.[1][2][6][7] Equivalently, it is the voltage that would be measured by cutting the wire to create an open circuit, and attaching a voltmeter to the leads.
Maxwell–Faraday equation
[edit]
The Maxwell–Faraday equation is a generalisation of Faraday's law that states that a time-varying magnetic field is always accompanied by a spatially-varying, non-conservative electric field, and vice-versa. The Maxwell–Faraday equation is
(in SI units) where is the curl operator and again E(r, t) is the electric field and B(r, t) is the magnetic field. These fields can generally be functions of position r and time t.
The Maxwell–Faraday equation is one of the four Maxwell's equations, and therefore plays a fundamental role in the theory of classical electromagnetism. It can also be written in an integral form by the Kelvin-Stokes theorem:[5]
where, as indicated in the figure:
- Σ is a surface bounded by the closed contour ∂Σ,
- E is the electric field, B is the magnetic field.
- dℓ is an infinitesimal vector element of the contour ∂Σ,
- dA is an infinitesimal vector element of surface Σ. If its direction is orthogonal to that surface patch, the magnitude is the area of an infinitesimal patch of surface.
Both dℓ and dA have a sign ambiguity; to get the correct sign, the right-hand rule is used, as explained in the article Kelvin-Stokes theorem. For a planar surface Σ, a positive path element dℓ of curve ∂Σ is defined by the right-hand rule as one that points with the fingers of the right hand when the thumb points in the direction of the normal n to the surface Σ.
The integral around ∂Σ is called a path integral or line integral.
- ^ a b c "The flux rule" is the terminology that Feynman uses to refer to the law relating magnetic flux to EMF.Richard Phillips Feynman, Leighton R B & Sands M L (2006). The Feynman Lectures on Physics. San Francisco: Pearson/Addison-Wesley. Vol. II, pp. 17-2. ISBN 0-8053-9049-9.
{{cite book}}: Unknown parameter|nopp=ignored (|no-pp=suggested) (help) - ^ a b Griffiths, David J. (1999). Introduction to Electrodynamics (Third ed.). Upper Saddle River NJ: Prentice Hall. pp. 301–303. ISBN 0-13-805326-X.
- ^ Tipler and Mosca, Physics for Scientists and Engineers, p795, google books link
- ^ Note that different textbooks may give different definitions. The set of equations used throughout the text was chosen to be compatible with the special relativity theory.
- ^ a b Roger F Harrington (2003). Introduction to electromagnetic engineering. Mineola, NY: Dover Publications. p. 56. ISBN 0-486-43241-6.
- ^ Tipler and Mosca, Physics for Scientists and Engineers, p795, google books link
- ^ Note that different textbooks may give different definitions. The set of equations used throughout the text was chosen to be compatible with the special relativity theory.





