User:Jono4174

Scoring Potential as a function of wickets and overs

thou
english verbs
English conjugation tables
plant chemical &spades

This page is about the nobility title. For other uses, see Duke (disambiguation).

This page is about comic book. For other uses, see Large numbers.

{{a|b|c|d|e|f}} { {tld | a | b | c | d | e | f } } {{a|b|c|d|e|f}} User:Jono4174#Knockout stage

[1]

Suffrage

Main article: Mateship

Mateship can be defined as the code of contact, particularly between men, although more recently also between men and women, stressing egalitarianism, equality and friendship. Mateship is seen as an important element of the qualities that the Australian Defence Force values in its soldiers, sailors, airmen and officers.

Theory

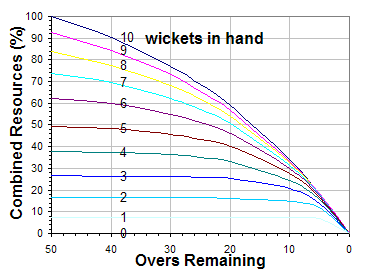
The cycle $x$ or $y$essence of the D/L method is "resources". Each team is taken to have two "resources" to use to make as many runs as possible: the number of overs they have to receive; and the number of wickets they have in hand. At any point in any innings, a team's ability to score more runs depends on the combination of these two resources. Looking at historical scores, there is a very close correspondence between the availability of these resources and a team's final score, a correspondence which D/L exploits.

Using a published table which gives the percentage of these combined resources remaining for any number of overs (or, more accurately, balls) left and wickets lost, the target score can be adjusted up or down to reflect the loss of resources to one or both teams when a match is shortened one or more times. This percentage is then used to calculate a target (sometimes called a "par score") that is usually a fractional number of runs, which is then rounded down. If the second team passes the target then the second team is taken to have won the match; if the match ends when the second team has exactly met (but not passed) the target then the match is taken to be a tie.

Cases

The most significant bit of the mantissa is determined by the value of exponent. If $0<$ exponent $<2^{e}-1$, the most significant bit of the mantissa is 1, and the number is said to be normalized. If exponent is 0, the most significant bit of the mantissa is 0 and the number is said to be de-normalized. Three special cases arise:

1. if exponent is 0 and mantissa is 0, the number is ±0 (depending on the sign bit)
2. if exponent = $2^{e}-1$ and mantissa is 0, the number is ±infinity (again depending on the sign bit), and
3. if exponent = $2^{e}-1$ and mantissa is not 0, the number being represented is not a number (NaN).

±(2¹²⁸ − 2¹⁰⁴) ≈ ±3.4028235×10³⁸

This can be summarized as:

Type	Exponent	Mantissa	Value
Zero	0000 0000	0000 0000 0000 0000 0000 000	0.0
Denormalized number	0000 0000	1000 0000 0000 0000 0000 000	5.9×10−39
Large normalized number	1111 1110	1111 1111 1111 1111 1111 111	3.4×1038
Small normalized number	0000 0001	0000 0000 0000 0000 0000 000	1.18×10−38
Infinity	1111 1111	0000 0000 0000 0000 0000 000	Infinity
NaN	1111 1111	0100 0000 0000 0000 0000 000	NaN

Here is the summary table from the previous section with some 32-bit single-precision examples:

Type	Sign	Exponent	Significand	Value
Zero	0	0000 0000	000 0000 0000 0000 0000 0000	0.0
One	0	0111 1111	000 0000 0000 0000 0000 0000	1.0
Minus One	1	0111 1111	000 0000 0000 0000 0000 0000	−1.0
Smallest denormalized number	*	0000 0000	000 0000 0000 0000 0000 0001	±2−23 × 2−126 = ±2−149 ≈ ±1.4×10−45
"Middle" denormalized number	*	0000 0000	100 0000 0000 0000 0000 0000	±2−1 × 2−126 = ±2−127 ≈ ±5.88×10−39
Largest denormalized number	*	0000 0000	111 1111 1111 1111 1111 1111	±(1−2−23) × 2−126 ≈ ±1.18×10−38
Smallest normalized number	*	0000 0001	000 0000 0000 0000 0000 0000	±2−126 ≈ 1.18×10−38
Largest normalized number	*	1111 1110	111 1111 1111 1111 1111 1111	±(1−2−24) × 2128 ≈ ±3.4×1038
Positive infinity	0	1111 1111	000 0000 0000 0000 0000 0000	$+\infty$
Negative infinity	1	1111 1111	000 0000 0000 0000 0000 0000	$-\infty$
Not a number	*	1111 1111	non zero	NaN
* Sign bit can be either 0 or 1 .

Here is the summary table from the previous section with some 32-bit single-precision examples: got rid of annoying +/- crap. It is supposed to be an example table.

Type	Sign	Exponent	Significand	Value
Zero	0	0000 0000	000 0000 0000 0000 0000 0000	0.0
One	0	0111 1111	000 0000 0000 0000 0000 0000	1.0
Minus One	1	0111 1111	000 0000 0000 0000 0000 0000	−1.0
Smallest denormalized number	0*	0000 0000	000 0000 0000 0000 0000 0001	2−23 × 2−126 = 2−149 ≈ 1.4×10−45
"Middle" denormalized number	0*	0000 0000	100 0000 0000 0000 0000 0000	2−1 × 2−126 = 2−127 ≈ 5.88×10−39
Largest denormalized number	0*	0000 0000	111 1111 1111 1111 1111 1111	(1−2−23) × 2−126 ≈ 1.18×10−38
Smallest normalized number	0*	0000 0001	000 0000 0000 0000 0000 0000	2−126 ≈ 1.18×10−38
Largest normalized number	0*	1111 1110	111 1111 1111 1111 1111 1111	(1−2−24) × 2128 ≈ 3.4×1038
Positive infinity	0	1111 1111	000 0000 0000 0000 0000 0000	$+\infty$
Negative infinity	1	1111 1111	000 0000 0000 0000 0000 0000	$-\infty$
Not a number	0*	1111 1111	non zero	NaN
(0*) Sign bit can be either 0 or 1. 0 is assumed for the value in the value column  .

Knockout stage

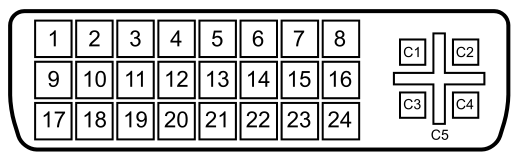
SCART_Connector_Pinout.svg File:Jono417.svg

Frequency on a logarithmic scale vs Position on Treble Clef. Each marker has a frequency of the previous note multiplied by (${\sqrt[{12}]{2}}$)

‎