User:Jcarroll/Dr. Edward Kramer

Title

Order and Disorder in Monolayers of Block Copolymer Cylinders Confined by Channels in SiOx

Abstract

Monolayer arrays of poly(styrene-b-2vinylpyridine) diblock copolymer cylinders with excellent orientational order and a very low density of dislocations are prepared by cooling slowly from above the bulk order-disorder temperature (ODT) ~212 °C within silicon oxide channels one cylinder spacing in depth and from 1 to 3 μm in width. If such an array is heated to a temperature above the glass transition temperature of the block copolymer (100 °C) but well below the ODT, a finite density of thermally generated dislocations is observed, which leads to a decrease in the translational correlation length and an appearance of quasi-long range orientational order such that the orientational correlation function g2(r) decays as a power law. The state of disorder at any given temperature is an equilibrium one since cylinder arrays with similar dislocation densities and correlation functions can be obtained either by heating from the well-ordered state or by slowly cooling directly to the final temperature and holding at that temperature for a sufficient time. Above a temperature of 195 °C, the orientational order becomes short range (g2(r) decays exponentially) and a large density of disclinations is observed in addition to the dislocations. The cylinder array becomes isotropic above this temperature, approximately 20 °C below the bulk ODT. These results are in qualitative agreement with the theory of Toner and Nelson that describes the thermal generation of disorder and ultimate melting of a two dimensional smectic.

Bio

Professor Kramer received a B.Ch.E. degree in Chemical Engineering from Cornell University in 1962 and his Ph.D. in Metallurgy and Materials Science from Carnegie-Mellon University in 1966.

He was a NATO Postdoctoral Fellow at Oxford with Sir Peter Hirsch, FRS, before joining the faculty of Cornell University in 1967 where he was appointed the Samuel B. Eckert Professor of Materials Science and Engineering in 1988. Nine years later he joined the faculty of UCSB holding a joint appointment in Materials and Chemical Engineering.

Professor Kramer's current research activities focus on polymer deformation and fracture as well as the structure and properties of polymer surfaces and polymer interfaces using a variety of depth profiling, diffraction and microscopic imaging methods. His group is interested in the deformation of block copolymers from a micromechanical and molecular viewpoint, the kinetics of grafting reactions and instabilities at polymer melt interfaces and the ordering of block copolymer films as templated by interfacial interactions and external fields as well as their interactions with inorganic nanoparticles whose surfaces are coated with short polymer chains.

Professional Awards and Honors:

Elected Fellow, American Physical Society (1983)
Polymer Physics Prize of the American Physical Society (1985)
Distinguished U.S. Senior Scientist Award of the Alexander von Humboldt Stiftung (1987)
Elected Guggenheim Fellow (1988)
Elected Member, National Academy of Engineering (1989)
Elected Fellow, American Association for the Advancement of Science (1994)
Docteur honoris causa awarded by L’Ecole Polytechnique Fédérale de Lausanne (1995)
Swinburne Award, Institute of Materials, UK (1996)
Chaire-Paris Sciences (2000)
Polymeer Technologie Nederland Medema Award (Dutch Polymer Society) (2007)
American Chemical Society Polymer Materials: Science and Engineering, Cooperative Research Award (with D. Hucul, S. F. Hahn, G. H. Fredrickson and F. S. Bates) (2008).

Technical Advisory Boards:

Related Terms

Monolayer

A monolayer is a single, closely packed layer of atoms, molecules, or cells. [1]. A Langmuir monolayer or insoluble monolayer is a one-molecule thick insoluble layer of an organic material spread onto an aqueous subphase. A Gibbs monolayer or soluble monolayer is a monolayer formed by a compound that is soluble in one of the phases separated by the interface on which the monolayer is formed.

Dislocation

In materials science, a dislocation is a crystallographic defect, or irregularity, within a crystal structure. The presence of dislocations strongly influences many of the properties of materials. The theory was originally developed by Vito Volterra in 1905. Some types of dislocations can be visualised as being caused by the termination of a plane of atoms in the middle of a crystal. In such a case, the surrounding planes are not straight, but instead bend around the edge of the terminating plane so that the crystal structure is perfectly ordered on either side. The analogy with a stack of paper is apt: if a half a piece of paper is inserted in a stack of paper, the defect in the stack is only noticeable at the edge of the half sheet.

There are two primary types: edge dislocations and screw dislocations. Mixed dislocations are intermediate between these.

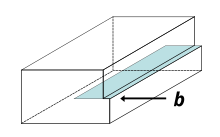
Mathematically, dislocations are a type of topological defect, sometimes called a soliton. The mathematical theory explains why dislocations behave as stable particles: they can be moved about, but maintain their identity as they move. Two dislocations of opposite orientation, when brought together, can cancel each other (this is the process of annihilation), but a single dislocation typically cannot "disappear" on its own.