Jump to content

User:JTiago/Sandbox

From Wikipedia, the free encyclopedia

(sandbox)


Overview

[edit]
Hexagonal Hanksite crystal, with three-fold c-axis symmetry

A lattice system is a class of lattices with the same point group. In three dimensions there are seven lattice systems: triclinic, monoclinic, orthorhombic, tetragonal, rhombohedral, hexagonal, and cubic. The lattice system of a crystal or space group is determined by its lattice but not always by its point group.

A crystal system is a class of point groups. Two point groups are placed in the same crystal system if the sets of possible lattice systems of their space groups are the same. For many point groups there is only one possible lattice system, and in these cases the crystal system corresponds to a lattice system and is given the same name. However, for the five point groups in the trigonal crystal class there are two possible lattice systems for their point groups: rhombohedral or hexagonal. In three dimensions there are seven crystal systems: triclinic, monoclinic, orthorhombic, tetragonal, trigonal, hexagonal, and cubic. The crystal system of a crystal or space group is determined by its point group but not always by its lattice.

A crystal family also consists of point groups and is formed by combining crystal systems whenever two crystal systems have space groups with the same lattice. In three dimensions a crystal family is almost the same as a crystal system (or lattice system), except that the hexagonal and trigonal crystal systems are combined into one hexagonal family. In three dimensions there are six crystal families: triclinic, monoclinic, orthorhombic, tetragonal, hexagonal, and cubic. The crystal family of a crystal or space group is determined by either its point group or its lattice, and crystal families are the smallest collections of point groups with this property.

In dimensions less than three there is no essential difference between crystal systems, crystal families, and lattice systems. There are 1 in dimension 0, 1 in dimension 1, and 4 in dimension 2, called oblique, rectangular, square, and hexagonal.

The relation between three-dimensional crystal families, crystal systems, and lattice systems is shown in the following table:

Crystal family Crystal system Required symmetries of point group point groups space groups bravais lattices Lattice system
Triclinic None 2 2 1 Triclinic
Monoclinic 1 twofold axis of rotation or 1 mirror plane 3 13 2 Monoclinic
Orthorhombic 3 twofold axes of rotation or 1 twofold axis of rotation and two mirror planes. 3 59 4 Orthorhombic
Tetragonal 1 fourfold axis of rotation 7 68 2 Tetragonal
Hexagonal Trigonal 1 threefold axis of rotation 5 7 1 Rhombohedral
18 1 Hexagonal
Hexagonal 1 sixfold axis of rotation 7 27
Cubic 4 threefold axes of rotation 5 36 3 Cubic
Total: 6 7 32 230 14 7

Crystal systems

[edit]

The distribution of the 32 point groups into the 7 crystal systems is given in the following table.

crystal family crystal system point group / crystal class Schönflies Hermann-Mauguin orbifold Type order structure
triclinic triclinic-pedial C1 1 11 enantiomorphic polar 1 trivial
triclinic-pinacoidal Ci 1 1x centrosymmetric 2 cyclic
monoclinic monoclinic-sphenoidal C2 2 22 enantiomorphic polar 2 cyclic
monoclinic-domatic Cs m 1* polar 2 cyclic
monoclinic-prismatic C2h 2/m 2* centrosymmetric 4 2×cyclic
orthorhombic orthorhombic-sphenoidal D2 222 222 enantiomorphic 4 dihedral
orthorhombic-pyramidal C2v mm2 *22 polar 4 dihedral
orthorhombic-bipyramidal D2h mmm *222 centrosymmetric 8 2×dihedral
tetragonal tetragonal-pyramidal C4 4 44 enantiomorphic polar 4 Cyclic
tetragonal-disphenoidal S4 4 2x   4 cyclic
tetragonal-dipyramidal C4h 4/m 4* centrosymmetric 8 2×cyclic
tetragonal-trapezoidal D4 422 422 enantiomorphic 8 dihedral
ditetragonal-pyramidal C4v 4mm *44 polar 8 dihedral
tetragonal-scalenoidal D2d 42m or 4m2 2*2   8 dihedral
ditetragonal-dipyramidal D4h 4/mmm *422 centrosymmetric 16 2×dihedral
hexagonal trigonal trigonal-pyramidal C3 3 33 enantiomorphic polar 3 cyclic
rhombohedral S6 (C3i) 3 3x centrosymmetric 6 cyclic
trigonal-trapezoidal D3 32 or 321 or 312 322 enantiomorphic 6 dihedral
ditrigonal-pyramidal C3v 3m or 3m1 or 31m *33 polar 6 dihedral
ditrigonal-scalahedral D3d 3m or 3m1 or 31m 2*3 centrosymmetric 12 dihedral
hexagonal hexagonal-pyramidal C6 6 66 enantiomorphic polar 6 cyclic
trigonal-dipyramidal C3h 6 3*   6 cyclic
hexagonal-dipyramidal C6h 6/m 6* centrosymmetric 12 2×cyclic
hexagonal-trapezoidal D6 622 622 enantiomorphic 12 dihedral
dihexagonal-pyramidal C6v 6mm *66 polar 12 dihedral
ditrigonal-dipyramidal D3h 6m2 or 62m *322   12 dihedral
dihexagonal-dipyramidal D6h 6/mmm *622 centrosymmetric 24 2×dihedral
gyroidal O 432 432 enantiomorphic 24 symmetric
hexoctahedral Oh m3m *432 centrosymmetric 48 2×symmetric

Lattice systems

[edit]

The distribution of the 14 Bravais lattice types into 7 lattice systems is given in the following table.

The 7 lattice systems The 14 Bravais Lattices
triclinic (parallelepiped) 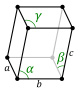Triclinic
monoclinic (right prism with parallelogram base; here seen from above) simple base-centered
Monoclinic, simple Monoclinic, centered
orthorhombic (cuboid) simple base-centered body-centered face-centered
Orthohombic, simple Orthohombic, base-centered Orthohombic, body-centered Orthohombic, face-centered
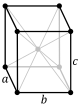
tetragonal (square cuboid) simple body-centered
Tetragonal, simple Tetragonal, body-centered
rhombohedral
(trigonal trapezohedron) 		Rhombohedral
hexagonal (centered regular hexagon) Hexagonal
cubic
(isometric; cube) 		simple body-centered face-centered
Cubic, simple Cubic, body-centered Cubic, face-centered

In geometry and crystallography, a Bravais lattice is a category of symmetry groups for translational symmetry in three directions, or correspondingly, a category of translation lattices.

Such symmetry groups consist of translations by vectors of the form

where n1, n2, and n3 are integers and a1, a2, and a3 are three non-coplanar vectors, called primitive vectors.

These lattices are classified by space group of the translation lattice itself; there are 14 Bravais lattices in three dimensions; each can apply in one lattice system only. They represent the maximum symmetry a structure with the translational symmetry concerned can have.

All crystalline materials must, by definition fit in one of these arrangements (not including quasicrystals).






For convenience a Bravais lattice is depicted by a unit cell which is a factor 1, 2, 3 or 4 larger than the primitive cell. Depending on the symmetry of a crystal or other pattern, the fundamental domain is again smaller, up to a factor 48.

The Bravais lattices were studied by Moritz Ludwig Frankenheim (1801-1869), in 1842, who found that there were 15 Bravais lattices. This was corrected to 14 by A. Bravais in 1848.

See also

[edit]

References

[edit]
  • Hahn, Theo, ed. (2002), International Tables for Crystallography, Volume A: Space Group Symmetry, vol. A (5th ed.), Berlin, New York: Springer-Verlag, doi:10.1107/97809553602060000100, ISBN 978-0-7923-6590-7
[edit]
Retrieved from "https://en.wikipedia.org/w/index.php?title=User:JTiago/Sandbox&oldid=1063514288"