User:Guy vandegrift/draft/Kepler and Newton

Johannes Kepler
Johannes Kepler
	A 1610 portrait of Johannes Kepler by an unknown artist
Born	December 27, 1571; Free Imperial City of Weil der Stadt, Holy Roman Empire
Died	November 15, 1630 (aged 58); Regensburg, Electorate of Bavaria, Holy Roman Empire
Nationality	German
Alma mater	University of Tübingen
Known for	Kepler's laws of planetary motion; Kepler conjecture
	Scientific career
Fields	Astronomy, astrology, mathematics and natural philosophy
Institutions	University of Linz
Signature

This is a Wikiversity resource

If you got here from Wikipedia click at right to see explanation

This is page is part of v:Astronomy college course, and was created by copying and editing some Wikipedia articles for use in Wikiversity. It could be transported over to Wikiversity, but that would require the tedious correction of redlinks and templates not found on Wikiversity. Is Wikipedia OK with this?

If you somehow reached this page through Wikipedia, you are welcome to take this wikiquiz.

Even though this is on my userspace, you may treat it as a true wiki, editable by anybody who is interested in improving open source learning. Be bold!

Kepler

from https://en.wikipedia.org/w/index.php?title=Johannes_Kepler&oldid=614434307

Johannes Kepler (German: [ˈkʰɛplɐ]; December 27, 1571 – November 15, 1630) was a German mathematician, astronomer, and astrologer. A key figure in the 17th century scientific revolution, he is best known for three laws of planetary motion that provided one of the foundations for Isaac Newton's theory of universal gravitation.

Kepler began his career as a mathematics teacher at a seminary school in Graz, Austria. Later he became an assistant to astronomer Tycho Brahe, and eventually the imperial mathematician to Emperor Rudolf II and his two successors Matthias and Ferdinand II. In addition to his contributions to astronomy, he invented an improved version of the refracting telescope (the Keplerian Telescope).

Kepler lived in an era when there was no clear distinction between astronomy and astrology, but there was a strong division between astronomy (a branch of mathematics and physics (a branch of natural philosophy). Kepler incorporated religious arguments and reasoning into his work, motivated by the religious conviction and belief that God had created the world according to an intelligible plan that is accessible through the natural light of reason.^[1]

Johannes Kepler's grandfather, Sebald Kepler, had been Lord Mayor of that town but, by the time Johannes was born, he had two brothers and one sister and the Kepler family fortune was in decline. His father, Heinrich Kepler, earned a precarious living as a mercenary, and he left the family when Johannes was five years old. He was believed to have died in the Eighty Years' War in the Netherlands. His mother Katharina Guldenmann, an inn-keeper's daughter, was a healer and herbalist. Born prematurely, Johannes claimed to have been weak and sickly as a child. Nevertheless, he often impressed travelers at his grandfather's inn with his phenomenal mathematical faculty.^[2]

He was introduced to astronomy at an early age, and developed a love for it that would span his entire life. At age six, he observed the Great Comet of 1577, writing that he "was taken by [his] mother to a high place to look at it."^[3] At age nine, he observed another astronomical event, a lunar eclipse in 1580, recording that he remembered being "called outdoors" to see it and that the moon "appeared quite red".^[3] However, childhood smallpox left him with weak vision and crippled hands, limiting his ability in the observational aspects of astronomy.^[4]

In 1589, Kepler attended Tübinger Stift at the University of Tübingen, where he studied philosophy and theology. He also proved himself to be a superb mathematician and earned a reputation as a skillful astrologer, casting horoscopes for fellow students. He learned both the Ptolemaic system and the Copernican system of planetary motion, and became a Copernican. Despite his desire to become a minister,Kepler was recommended for a position as teacher of mathematics and astronomy at the Protestant school in Graz (later the University of Graz). He accepted the position in April 1594, at the age of 23.^[5]

Mysterium Cosmographicum (The Cosmic Mystery)

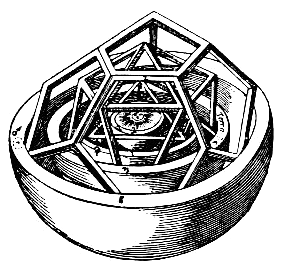
Not all of Kepler's ideas withstood the test of time. In an book with a title often translated as The Cosmic Mystery (1596), Kepler attempted to find a mathematical pattern among the distances of the planets to the Sun using the five Platonic solids. The figure to the left shows an outer sphere representing the orbit of Jupiter around the Sun (at the center) Just inside is a sphere representing the orbit of Saturn. A detailed drawing of the inner sphere contains the orbits of the terrestrial planets, as shown to the right.

Newton and Kepler's three laws of planetary motion

From https://en.wikipedia.org/w/index.php?title=Kepler%27s_laws_of_planetary_motion&oldid=613937431

Johannes Kepler published his first two laws about planetary motion in 1609, having found them by analyzing the astronomical observations of Tycho Brahe.^[6]^[7]^[8] Kepler's third law was published in 1619.^[9]^[7]

Kepler's three laws can be stated as:

The orbit of a planet is an ellipse with the Sun at one of the two foci.
A line segment joining a planet and the Sun sweeps out equal areas during equal intervals of time.^[10] This implies that planets speed up as they get closer to the Sun.
The square of the orbital period of a planet is proportional to the cube of the semi-major axis of its orbit. This implies that the planets farthest from the Sun have the longest periods of orbit around the Sun. It also implies that these distant planets are moving at a slower speed.

All three laws were discovered by analyzing the position of planets in the sky, not by establishing laws that govern motion. That task was left to Isaac Newton, who developed principles that seemed to govern the motion of all objects, including those on Earth. We can state Newton's theories about motion and gravity in modern notation using vector calculus:

{\frac {d^{2}}{dt^{2}}}{\vec {\mathcal {R}}}=-(m+M)G{\frac {\hat {R}}{R^{2}}}

Very few people need to know vector calculus, but a solution to this equation^[11] is essential to our understanding of the cosmos. Without going into details, it allows one to observe the motion of a distant object and deduce it's mass. In this equation, $M+m$ is the total mass of two objects orbiting each other. Loosely speaking, this equation allows us to "weigh" almost any object we see in the universe.

The constant "G" tells us how strong the force of gravity is. For example, if a person of mass M₁ is standing a distance R away from a person of mass M₂, then the force of attraction, F, between them is calculated using:

F=G{\frac {M_{1}M_{2}}{R^{2}}}

For objects weighing only a few hundred kilograms, and standing a few centimeters apart, this is a very small force because G is a very small number.

Uniting heaven and earth

Without providing any evidence supporting either system, Copernicus offered the heliocentric system as a model that seems to offer a simple explanation for the odd motion of planets in the sky.

By using the telescope Galileo offered the opportunity to carefully study the sky just as humans carefully study and inspect objects they find on earth. Galileo sketches indicated that the Moon has mountains, Venus has phases, and Jupiter has moons.

Kepler found mathematical equations that match the speed and shapes of the paths taken by the planets as they orbit the sun, but offered no explanation for why these equations hold.

Newton wrote succinct rules for how all objects move and invented the mathematics (called calculus) needed to solve these equations. The constant "G" in his equation is called the universal constant of gravity because this law holds everywhere in the universe. and for all time. (Or at least it might hold everywhere and for all time...). Newton did not know the numerical value of "G", and for that reason could only speak of ratios. For example, when the distance to Jupiter was later measured, Newton's laws could be used to show that the Sun is about 1000 times more massive than Jupiter. But without a knowledge of "G", the actual mass of the Sun (or Jupiter) was unknown. Cavandish finally measured the minute force between two lead balls on earth and further refinements of that measurement have established that G≈6.67×10⁻¹¹ N·(m/kg)².

And that, in a nutshell, is how we know the mass of the Sun and other stars.

Quiz

Quiz

References

references are hidden

^ Barker and Goldstein. "Theological Foundations of Kepler's Astronomy", pp. 112–13.
^ Caspar. Kepler, pp. 29–36; Connor. Kepler's Witch, pp. 23–46.
^ ^a ^b Koestler. The Sleepwalkers, p. 234 (translated from Kepler's family horoscope).
^ Caspar. Kepler, pp. 36–38; Connor. Kepler's Witch, pp. 25–27.
^ Caspar. Kepler, pp. 38–52; Connor. Kepler's Witch, pp. 49–69.
^ In his Astronomia nova, Kepler presented only a proof that Mars' orbit is elliptical. Evidence that the other known planets' orbits are elliptical was presented only in 1621.
See: Johannes Kepler, Astronomia nova … (1609), p. 285. After having rejected circular and oval orbits, Kepler concluded that Mars' orbit must be elliptical. From the top of page 285: "Ergo ellipsis est Planetæ iter; … " (Thus, an ellipse is the planet's [i.e., Mars'] path; … ) Later on the same page: " … ut sequenti capite patescet: ubi simul etiam demonstrabitur, nullam Planetæ relinqui figuram Orbitæ, præterquam perfecte ellipticam; … " ( … as will be revealed in the next chapter: where it will also then be proved that any figure of the planet's orbit must be relinquished, except a perfect ellipse; … ) And then: "Caput LIX. Demonstratio, quod orbita Martis, … , fiat perfecta ellipsis: … " (Chapter 59. Proof that Mars' orbit, … , is a perfect ellipse: … ) The geometric proof that Mars' orbit is an ellipse appears as Protheorema XI on pages 289-290.
Kepler stated that all planets travel in elliptical orbits having the Sun at one focus in: Johannes Kepler, Epitome Astronomiae Copernicanae [Summary of Copernican Astronomy] (Linz ("Lentiis ad Danubium"), (Austria): Johann Planck, 1622), book 5, part 1, III. De Figura Orbitæ (III. On the figure [i.e., shape] of orbits), pages 658-665. From p. 658: "Ellipsin fieri orbitam planetæ … " (Of an ellipse is made a planet's orbit … ). From p. 659: " … Sole (Foco altero huius ellipsis) … " ( … the Sun (the other focus of this ellipse) … ).
^ ^a ^b Holton, Gerald James; Brush, Stephen G. (2001). Physics, the Human Adventure: From Copernicus to Einstein and Beyond (3rd paperback ed.). Piscataway, NJ: Rutgers University Press. pp. 40–41. ISBN 0-8135-2908-5. Retrieved December 27, 2009.
^
In his Astronomia nova … (1609), Kepler did not present his second law in its modern form. He did that only in his Epitome of 1621. Furthermore, in 1609, he presented his second law in two different forms, which scholars call the "distance law" and the "area law".
- His "distance law" is presented in: "Caput XXXII. Virtutem quam Planetam movet in circulum attenuari cum discessu a fonte." (Chapter 32. The force that moves a planet circularly weakens with distance from the source.) See: Johannes Kepler, Astronomia nova … (1609), pp. 165-167. On page 167, Kepler states: " … , quanto longior est αδ quam αε, tanto diutius moratur Planeta in certo aliquo arcui excentrici apud δ, quam in æquali arcu excentrici apud ε." ( … , as αδ is longer than αε, so much longer will a planet remain on a certain arc of the eccentric near δ than on an equal arc of the eccentric near ε.) That is, the farther a planet is from the Sun (at the point α), the slower it moves along its orbit, so a radius from the Sun to a planet passes through equal areas in equal times. However, as Kepler presented it, his argument is accurate only for circles, not ellipses.
- His "area law" is presented in: "Caput LIX. Demonstratio, quod orbita Martis, … , fiat perfecta ellipsis: … " (Chapter 59. Proof that Mars' orbit, … , is a perfect ellipse: … ), Protheorema XIV and XV, pp. 291-295. On the top p. 294, it reads: "Arcum ellipseos, cujus moras metitur area AKN, debere terminari in LK, ut sit AM." (The arc of the ellipse, of which the duration is delimited [i.e., measured] by the area AKM, should be terminated in LK, so that it [i.e., the arc] is AM.) In other words, the time that Mars requires to move along an arc AM of its elliptical orbit is measured by the area of the segment AMN of the ellipse (where N is the position of the Sun), which in turn is proportional to the section AKN of the circle that encircles the ellipse and that is tangent to it. Therefore, the area that is swept out by a radius from the Sun to Mars as Mars moves along an arc of its elliptical orbit is proportional to the time that Mars requires to move along that arc. Thus, a radius from the Sun to Mars sweeps out equal areas in equal times.
In 1621, Kepler restated his second law for any planet: Johannes Kepler, Epitome Astronomiae Copernicanae [Summary of Copernican Astronomy] (Linz ("Lentiis ad Danubium"), (Austria): Johann Planck, 1622), book 5, page 668. From page 668: "Dictum quidem est in superioribus, divisa orbita in particulas minutissimas æquales: accrescete iis moras planetæ per eas, in proportione intervallorum inter eas & Solem." (It has been said above that, if the orbit of the planet is divided into the smallest equal parts, the times of the planet in them increase in the ratio of the distances between them and the sun.) That is, a planet's speed along its orbit is inversely proportional to its distance from the Sun. (The remainder of the paragraph makes clear that Kepler was referring to what is now called angular velocity.)
^ Johannes Kepler, Harmonices Mundi [The Harmony of the World] (Linz, (Austria): Johann Planck, 1619), book 5, chapter 3, p. 189. From the bottom of p. 189: "Sed res est certissima exactissimaque quod proportio qua est inter binorum quorumcunque Planetarum tempora periodica, sit præcise sesquialtera proportionis mediarum distantiarum, … " (But it is absolutely certain and exact that the proportion between the periodic times of any two planets is precisely the sesquialternate proportion [i.e., the ratio of 3:2] of their mean distances, … ")
An English translation of Kepler's Harmonices Mundi is available as: Johannes Kepler with E.J. Aiton, A.M. Duncan, and J.V. Field, trans., The Harmony of the World (Philadelphia, Pennsylvania: American Philosophical Society, 1997); see especially p. 411.
^ Bryant, Jeff; Pavlyk, Oleksandr. "Kepler's Second Law", Wolfram Demonstrations Project. Retrieved December 27, 2009.
^ Here, ${\vec {\mathcal {R}}}\;$ is the displacement vector pointing from one (infinitely small) object to the next, and ${\hat {\mathcal {R}}}\;$ is a unit vector.

[1] Barker and Goldstein. "Theological Foundations of Kepler's Astronomy", pp. 112–13.

[2] Caspar. Kepler, pp. 29–36; Connor. Kepler's Witch, pp. 23–46.

[Koestler234-3] Koestler. The Sleepwalkers, p. 234 (translated from Kepler's family horoscope).

[4] Caspar. Kepler, pp. 36–38; Connor. Kepler's Witch, pp. 25–27.

[5] Caspar. Kepler, pp. 38–52; Connor. Kepler's Witch, pp. 49–69.

[6] In his Astronomia nova, Kepler presented only a proof that Mars' orbit is elliptical. Evidence that the other known planets' orbits are elliptical was presented only in 1621.
See: Johannes Kepler, Astronomia nova … (1609), p. 285. After having rejected circular and oval orbits, Kepler concluded that Mars' orbit must be elliptical. From the top of page 285: "Ergo ellipsis est Planetæ iter; … " (Thus, an ellipse is the planet's [i.e., Mars'] path; … ) Later on the same page: " … ut sequenti capite patescet: ubi simul etiam demonstrabitur, nullam Planetæ relinqui figuram Orbitæ, præterquam perfecte ellipticam; … " ( … as will be revealed in the next chapter: where it will also then be proved that any figure of the planet's orbit must be relinquished, except a perfect ellipse; … ) And then: "Caput LIX. Demonstratio, quod orbita Martis, … , fiat perfecta ellipsis: … " (Chapter 59. Proof that Mars' orbit, … , is a perfect ellipse: … ) The geometric proof that Mars' orbit is an ellipse appears as Protheorema XI on pages 289-290.
Kepler stated that all planets travel in elliptical orbits having the Sun at one focus in: Johannes Kepler, Epitome Astronomiae Copernicanae [Summary of Copernican Astronomy] (Linz ("Lentiis ad Danubium"), (Austria): Johann Planck, 1622), book 5, part 1, III. De Figura Orbitæ (III. On the figure [i.e., shape] of orbits), pages 658-665. From p. 658: "Ellipsin fieri orbitam planetæ … " (Of an ellipse is made a planet's orbit … ). From p. 659: " … Sole (Foco altero huius ellipsis) … " ( … the Sun (the other focus of this ellipse) … ).

[Holton-7] Holton, Gerald James; Brush, Stephen G. (2001). Physics, the Human Adventure: From Copernicus to Einstein and Beyond (3rd paperback ed.). Piscataway, NJ: Rutgers University Press. pp. 40–41. ISBN 0-8135-2908-5. Retrieved December 27, 2009.

[8] In his Astronomia nova … (1609), Kepler did not present his second law in its modern form. He did that only in his Epitome of 1621. Furthermore, in 1609, he presented his second law in two different forms, which scholars call the "distance law" and the "area law".
His "distance law" is presented in: "Caput XXXII. Virtutem quam Planetam movet in circulum attenuari cum discessu a fonte." (Chapter 32. The force that moves a planet circularly weakens with distance from the source.) See: Johannes Kepler, Astronomia nova … (1609), pp. 165-167. On page 167, Kepler states: " … , quanto longior est αδ quam αε, tanto diutius moratur Planeta in certo aliquo arcui excentrici apud δ, quam in æquali arcu excentrici apud ε." ( … , as αδ is longer than αε, so much longer will a planet remain on a certain arc of the eccentric near δ than on an equal arc of the eccentric near ε.) That is, the farther a planet is from the Sun (at the point α), the slower it moves along its orbit, so a radius from the Sun to a planet passes through equal areas in equal times. However, as Kepler presented it, his argument is accurate only for circles, not ellipses.

His "area law" is presented in: "Caput LIX. Demonstratio, quod orbita Martis, … , fiat perfecta ellipsis: … " (Chapter 59. Proof that Mars' orbit, … , is a perfect ellipse: … ), Protheorema XIV and XV, pp. 291-295. On the top p. 294, it reads: "Arcum ellipseos, cujus moras metitur area AKN, debere terminari in LK, ut sit AM." (The arc of the ellipse, of which the duration is delimited [i.e., measured] by the area AKM, should be terminated in LK, so that it [i.e., the arc] is AM.) In other words, the time that Mars requires to move along an arc AM of its elliptical orbit is measured by the area of the segment AMN of the ellipse (where N is the position of the Sun), which in turn is proportional to the section AKN of the circle that encircles the ellipse and that is tangent to it. Therefore, the area that is swept out by a radius from the Sun to Mars as Mars moves along an arc of its elliptical orbit is proportional to the time that Mars requires to move along that arc. Thus, a radius from the Sun to Mars sweeps out equal areas in equal times.
In 1621, Kepler restated his second law for any planet: Johannes Kepler, Epitome Astronomiae Copernicanae [Summary of Copernican Astronomy] (Linz ("Lentiis ad Danubium"), (Austria): Johann Planck, 1622), book 5, page 668. From page 668: "Dictum quidem est in superioribus, divisa orbita in particulas minutissimas æquales: accrescete iis moras planetæ per eas, in proportione intervallorum inter eas & Solem." (It has been said above that, if the orbit of the planet is divided into the smallest equal parts, the times of the planet in them increase in the ratio of the distances between them and the sun.) That is, a planet's speed along its orbit is inversely proportional to its distance from the Sun. (The remainder of the paragraph makes clear that Kepler was referring to what is now called angular velocity.)

[9] His "distance law" is presented in: "Caput XXXII. Virtutem quam Planetam movet in circulum attenuari cum discessu a fonte." (Chapter 32. The force that moves a planet circularly weakens with distance from the source.) See: Johannes Kepler, Astronomia nova … (1609), pp. 165-167. On page 167, Kepler states: " … , quanto longior est αδ quam αε, tanto diutius moratur Planeta in certo aliquo arcui excentrici apud δ, quam in æquali arcu excentrici apud ε." ( … , as αδ is longer than αε, so much longer will a planet remain on a certain arc of the eccentric near δ than on an equal arc of the eccentric near ε.) That is, the farther a planet is from the Sun (at the point α), the slower it moves along its orbit, so a radius from the Sun to a planet passes through equal areas in equal times. However, as Kepler presented it, his argument is accurate only for circles, not ellipses.

[10] His "area law" is presented in: "Caput LIX. Demonstratio, quod orbita Martis, … , fiat perfecta ellipsis: … " (Chapter 59. Proof that Mars' orbit, … , is a perfect ellipse: … ), Protheorema XIV and XV, pp. 291-295. On the top p. 294, it reads: "Arcum ellipseos, cujus moras metitur area AKN, debere terminari in LK, ut sit AM." (The arc of the ellipse, of which the duration is delimited [i.e., measured] by the area AKM, should be terminated in LK, so that it [i.e., the arc] is AM.) In other words, the time that Mars requires to move along an arc AM of its elliptical orbit is measured by the area of the segment AMN of the ellipse (where N is the position of the Sun), which in turn is proportional to the section AKN of the circle that encircles the ellipse and that is tangent to it. Therefore, the area that is swept out by a radius from the Sun to Mars as Mars moves along an arc of its elliptical orbit is proportional to the time that Mars requires to move along that arc. Thus, a radius from the Sun to Mars sweeps out equal areas in equal times.

[Kepler_1619-9] Johannes Kepler, Harmonices Mundi [The Harmony of the World] (Linz, (Austria): Johann Planck, 1619), book 5, chapter 3, p. 189. From the bottom of p. 189: "Sed res est certissima exactissimaque quod proportio qua est inter binorum quorumcunque Planetarum tempora periodica, sit præcise sesquialtera proportionis mediarum distantiarum, … " (But it is absolutely certain and exact that the proportion between the periodic times of any two planets is precisely the sesquialternate proportion [i.e., the ratio of 3:2] of their mean distances, … ")
An English translation of Kepler's Harmonices Mundi is available as: Johannes Kepler with E.J. Aiton, A.M. Duncan, and J.V. Field, trans., The Harmony of the World (Philadelphia, Pennsylvania: American Philosophical Society, 1997); see especially p. 411.

[Wolfram2nd-10] Bryant, Jeff; Pavlyk, Oleksandr. "Kepler's Second Law", Wolfram Demonstrations Project. Retrieved December 27, 2009.

[11] Here, ${\vec {\mathcal {R}}}\;$ is the displacement vector pointing from one (infinitely small) object to the next, and ${\hat {\mathcal {R}}}\;$ is a unit vector.

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]