From Wikipedia, the free encyclopedia
Category:Statistics templates
Category:Sidebar templates
Category:Statistics templates
Category:Sidebar templates by topic
Category:Statistics templates
Category:Sidebar templates
Category:Statistics templates
Category:Sidebar templates by topic
Symmetry properties
[edit] Symmetry poperties of the Fourier series.
Symmetry poperties of the Fourier series.
- If
 is a real function, then
is a real function, then  (Hermitian symmetric) which implies:
(Hermitian symmetric) which implies:
 (real part is even symmetric)
(real part is even symmetric) (imaginary part is odd symmetric)
(imaginary part is odd symmetric) (absolut value is even symmetric)
(absolut value is even symmetric) (argument is odd symmetric)
(argument is odd symmetric)
- If
 is a real and even function (
is a real and even function ( ), then all coefficients
), then all coefficients  are real and
are real and  (even symmetric) which implies:
(even symmetric) which implies:
 for all
for all 
- If
 is a real and odd function (
is a real and odd function ( ), then all coefficients
), then all coefficients  are purely imaginary and
are purely imaginary and  (odd symmetric) which implies:
(odd symmetric) which implies:
 for all
for all 
- If
 is a purely imaginary function, then
is a purely imaginary function, then  which implies:
which implies:
 (real part is odd symmetric)
(real part is odd symmetric) (imaginary part is even symmetric)
(imaginary part is even symmetric) (absolut value is even symmetric)
(absolut value is even symmetric) (argument is odd symmetric)
(argument is odd symmetric)
- If
 is a purely imaginary and even function (
is a purely imaginary and even function ( ), then all coefficients
), then all coefficients  are purely imaginary and
are purely imaginary and  (even symmetric).
(even symmetric).
- If
 is a purely imaginary and odd function (
is a purely imaginary and odd function ( ), then all coefficients
), then all coefficients  are real and
are real and  (odd symmetric).
(odd symmetric).
Table of Fourier Series coefficients
[edit]Some common pairsof periodic functions and their Fourier Series coefficients are shown in the table below. The following notation applies:
 designates a periodic function defined on
designates a periodic function defined on  .
. designates a ...
designates a ... designates a ...
designates a ...
Time domain

|
Plot
|
Frequency domain (sine-cosine form)

|
Remarks
|
Reference
|

|
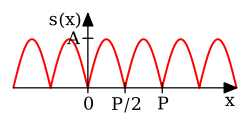
|

|
Full-wave rectified sine
|
[1]: p. 193
|

|

|

|
Full-wave rectified sine cut by a phase-fired controller

|
|

|
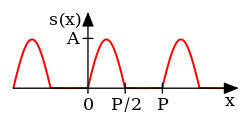
|

|
Half-wave rectified sine
|
[1]: p. 193
|

|

|

|

|
|

|
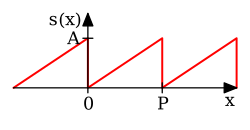
|

|
|
[1]: p. 192
|

|

|

|
|
[1]: p. 192
|

|

|

|
|
[1]: p. 193
|

|

|

|

|
|

|

|

|

|
|
|
|

|
|
|
 denotes the Dirac delta function. denotes the Dirac delta function.
|
This table shows some mathematical operations in the time domain and the corresponding effects in the frequency domain.
![{\displaystyle x[n]^{*}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/edad1abfdca495a8e9ecb60b39a6e79acf203073) is the complex conjugate of
is the complex conjugate of ![{\displaystyle x[n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/864cbbefbdcb55af4d9390911de1bf70167c4a3d) .
. designate a
designate a  -periodic functions defined on
-periodic functions defined on  .
.![{\displaystyle {\hat {f}}[n],{\hat {g}}[n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f50ab8d7e2fd3015f90e7784881bb7f154efe7ba) designates the Fourier series coefficients (exponential form) of
designates the Fourier series coefficients (exponential form) of  and
and  as defined in equation TODO!!!
as defined in equation TODO!!!
| Property
|
Time domain
|
Frequency domain (exponential form)
|
Remarks
|
Reference
|
| Linearity
|

|
![{\displaystyle a\cdot {\hat {f}}[n]+b\cdot {\hat {g}}[n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/78b071572e3e6cd2544abd96944994db48c1ce4c)
|
complex numbers 
|
|
| Time reversal / Frequency reversal
|

|
![{\displaystyle {\hat {f}}[-n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/60add86c025d75f59d9ddd3f471344c8e0c90505)
|
|
[2]: p. 610
|
| Time conjugation
|

|
![{\displaystyle {\hat {f}}[-n]^{*}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ff4724032767a7a90eca846e00b35ecb0822eb8c)
|
|
[2]: p. 610
|
| Time reversal & conjugation
|

|
![{\displaystyle {\hat {f}}[n]^{*}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c31ad5b2648f054500e5636d0a6893e40e9c06dd)
|
|
|
| Real part in time
|

|
![{\displaystyle {\frac {1}{2}}({\hat {f}}[n]+{\hat {f}}[-n]^{*})}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5af06127ce44129006367cc5ffa3d6e961f2cba2)
|
|
|
| Imaginary part in time
|

|
![{\displaystyle {\frac {1}{2i}}({\hat {f}}[n]-{\hat {f}}[-n]^{*})}](https://wikimedia.org/api/rest_v1/media/math/render/svg/40d95a967088459740cd89175c83267195aef828)
|
|
|
| Real part in frequency
|

|
![{\displaystyle \Re {({\hat {f}}[n])}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/521be13a110acf89cdcc47f6086a09e777ec8ab4)
|
|
|
| Imaginary part in frequency
|

|
![{\displaystyle \Im {({\hat {f}}[n])}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e5a3e657f288f8b1de1ee49e36a62516673af2a6)
|
|
|
| Shift in time / Modulation in frequency
|

|
![{\displaystyle {\hat {f}}[n]\cdot e^{-i{\frac {2\pi x_{0}}{T}}n}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/42bab8c6b012881f474a232f203ab318d108beb7)
|
real number 
|
[2]: p. 610
|
| Shift in frequency / Modulation in time
|

|
![{\displaystyle {\hat {f}}[n-n_{0}]\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/77c91fdb8e5db05fd4fe38802b08df8955d99c2b)
|
integer 
|
[2]: p. 610
|
| Differencing in frequency
|
|
|
|
|
| Summation in frequency
|
|
|
|
|
| Derivative in time
|

|
![{\displaystyle j{\frac {2\pi n}{T}}{\hat {f}}[n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b2b962c8a84b66e888ce08013fff9b0491f2cc31)
|
|
|
Derivative in time ( times) times)
|

|

|
|
|
| Integration in time
|

|

|
|
|
| Convolution in time / Multiplication in frequency
|

|
![{\displaystyle {\frac {1}{T}}{\hat {f}}[n]\cdot {\hat {g}}[n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/447767b1d2a75139ece136539e2c7e8a0ab30913)
|
 denotes continuous circular convolution. denotes continuous circular convolution.
|
|
| Multiplication in time / Convolution in frequency
|

|
![{\displaystyle ({\hat {f}}*{\hat {g}})[n]=\sum _{k=-\infty }^{\infty }{\hat {f}}[k]{\hat {g}}[n-k]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ee5ebe46e1c135d4dd411bab5bdcc88b6743ec90)
|
 denotes Discrete convolution. denotes Discrete convolution.
|
|
| Cross correlation
|
![{\displaystyle \rho _{xy}[n]=x[-n]^{*}*y[n]\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/37d82c9624d4b16650c5652c000d0bb459f794c6)
|

|
|
|
| Parseval's theorem
|
![{\displaystyle E_{xy}=\sum _{n=-\infty }^{\infty }{x[n]\cdot y[n]^{*}}\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cbf0fbe742415c7175c4a84a280c32d1d713ae5c)
|

|
|
[3]: p. 236
|
- ^ a b c d e Papula, Lothar (2009). Mathematische Formelsammlung: für Ingenieure und Naturwissenschaftler. Vieweg+Teubner Verlag. ISBN 3834807575.
- ^ a b c d Shmaliy, Y.S. (2007). Continuous-Time Signals. Springer. ISBN 1402062710.
- ^ Cite error: The named reference
ProakisManolakis was invoked but never defined (see the help page).





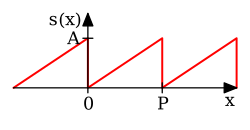



















































![{\displaystyle x[n]^{*}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/edad1abfdca495a8e9ecb60b39a6e79acf203073)
![{\displaystyle x[n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/864cbbefbdcb55af4d9390911de1bf70167c4a3d)



![{\displaystyle {\hat {f}}[n],{\hat {g}}[n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f50ab8d7e2fd3015f90e7784881bb7f154efe7ba)


![{\displaystyle a\cdot {\hat {f}}[n]+b\cdot {\hat {g}}[n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/78b071572e3e6cd2544abd96944994db48c1ce4c)


![{\displaystyle {\hat {f}}[-n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/60add86c025d75f59d9ddd3f471344c8e0c90505)

![{\displaystyle {\hat {f}}[-n]^{*}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ff4724032767a7a90eca846e00b35ecb0822eb8c)

![{\displaystyle {\hat {f}}[n]^{*}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c31ad5b2648f054500e5636d0a6893e40e9c06dd)

![{\displaystyle {\frac {1}{2}}({\hat {f}}[n]+{\hat {f}}[-n]^{*})}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5af06127ce44129006367cc5ffa3d6e961f2cba2)

![{\displaystyle {\frac {1}{2i}}({\hat {f}}[n]-{\hat {f}}[-n]^{*})}](https://wikimedia.org/api/rest_v1/media/math/render/svg/40d95a967088459740cd89175c83267195aef828)

![{\displaystyle \Re {({\hat {f}}[n])}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/521be13a110acf89cdcc47f6086a09e777ec8ab4)

![{\displaystyle \Im {({\hat {f}}[n])}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e5a3e657f288f8b1de1ee49e36a62516673af2a6)

![{\displaystyle {\hat {f}}[n]\cdot e^{-i{\frac {2\pi x_{0}}{T}}n}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/42bab8c6b012881f474a232f203ab318d108beb7)


![{\displaystyle {\hat {f}}[n-n_{0}]\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/77c91fdb8e5db05fd4fe38802b08df8955d99c2b)


![{\displaystyle j{\frac {2\pi n}{T}}{\hat {f}}[n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b2b962c8a84b66e888ce08013fff9b0491f2cc31)



![{\displaystyle {\frac {1}{T}}{\hat {f}}[n]\cdot {\hat {g}}[n]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/447767b1d2a75139ece136539e2c7e8a0ab30913)


![{\displaystyle ({\hat {f}}*{\hat {g}})[n]=\sum _{k=-\infty }^{\infty }{\hat {f}}[k]{\hat {g}}[n-k]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ee5ebe46e1c135d4dd411bab5bdcc88b6743ec90)
![{\displaystyle \rho _{xy}[n]=x[-n]^{*}*y[n]\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/37d82c9624d4b16650c5652c000d0bb459f794c6)

![{\displaystyle E_{xy}=\sum _{n=-\infty }^{\infty }{x[n]\cdot y[n]^{*}}\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cbf0fbe742415c7175c4a84a280c32d1d713ae5c)
