Talk:Spacetime/Archive 7
| This is an archive of past discussions about Spacetime. Do not edit the contents of this page. If you wish to start a new discussion or revive an old one, please do so on the current talk page. |
| Archive 1 | ← | Archive 5 | Archive 6 | Archive 7 | Archive 8 | Archive 9 | Archive 10 |
My apologies for trying
Apparently this article is owned, as is clear by the immediate petty reverts to an article asking for help. I tried. But I won't again. 75.139.254.117 (talk) 00:01, 15 March 2017 (UTC)
Asking for input on the revised introduction
I'm pretty much finished with what I was planning to do with the Introduction.
Could some of you provide comment?
- Have I committed any factual errors that need to be addressed?
- My imagined audience while I was re-writing the introduction was a typical High School science major. Have I succeeded in my aim of making the introduction understandable by my target audience?
Thanks!
Stigmatella aurantiaca (talk) 13:28, 26 March 2017 (UTC)
- I'm too new to get involved with editing this article, but there is a minor problem with the 3rd paragraph of the article. Keith o (talk) 05:52, 4 April 2017 (UTC)
- Could you specify more precisely what you consider to be a problem? Stigmatella aurantiaca (talk) 08:51, 4 April 2017 (UTC)
- @Keith o: Upon reading the paragraph carefully, yes, I do see an issue. Thanks, Keith! Let me see what I can do to fix the paragraph without introducing too much extra complexity! Stigmatella aurantiaca (talk) 09:14, 4 April 2017 (UTC)
- • It looks like a good improvementn to me. Thanks. Greg L (talk) 23:43, 4 April 2017 (UTC)
- Could you specify more precisely what you consider to be a problem? Stigmatella aurantiaca (talk) 08:51, 4 April 2017 (UTC)
- BRAVO! I think you have done a fabulous job. More on my thoughts are here on my talk page in response to your reaching out to me. I suggest other wikipedians sit back and watch as you do more of your heavy lifting for a while. Greg L (talk) 19:29, 26 March 2017 (UTC)
- The rest of the article is in too serious a mess for me to consider tackling, especially since a fair amount of it covers topics with which I cannot claim mastery. What I am considering is an "Introduction, Part 2" which will include gravity. Also, as you pointed out on your talk page, I need to pay attention to the lede. Being so focused on the Introduction, I didn't give the lede anywhere near as much attention as I should have. Stigmatella aurantiaca (talk) 19:45, 26 March 2017 (UTC)
- @Greg L: Feedback from others not being forthcoming, I incorporated into the lede various of your suggestions to reduce excessive pithiness and jargon that, as you put it, requires the reader to engage in the Wikipedia practice of Click to Learn©™®. Stigmatella aurantiaca (talk) 16:30, 27 March 2017 (UTC)
- What you have in the lede is a giant improvement. I did a little research to double check something; things seem correct.
- Very many readers read no more than the lede so what you've got there now is pretty much all one needs for a quick, one-stop tutorial. Wikipedia now has a pretty nice article on an exceedingly technical topic (where “quality” and “technical” seldom play well). I trifurcated your giant, one-paragraph lede at natural points; I hope you don't mind the quick intrusion and failure to allow you to get around to that.
- I wouldn't be shy about increasing the length of the lede so long as you use plain-speak targeted to intelligent, diligent 10th graders truly interested in the broad topic. Expanding upon the subject of not fearing lengthening the lede:
- I can slave for weeks on just a lede and leave the rest of the article untouched. This is an old version of Entropic gravity article that had a seriously abstruse lede. Carefully read it (don't read any further than the lede) and then consider just how much you truly got out of it and how much you really *understand* of the subject matter. Then read this version of the article after I would spend a few weeks editing, wait a few days, go back and read what I had written, and edit. Rinse & repeat. Rinse & repeat. Note how the accessible reading level (getting seriously technical only at the very end) allows learning important fundamentals without having to wade into the bowels of the article. Greg L (talk) 03:28, 28 March 2017 (UTC)
I took the liberty of revising the lede to streamline it and better make it consistent with both Minkowski space and Four-dimensional space. Once the lede is fully fleshed out and is well-focused on the proper distinctions between Einstein's view and Minkowski's view, I think the rest of the article will naturally fall into place. Greg L (talk) 18:22, 28 March 2017 (UTC)
- Einstein was well aware of invariants. You make it seem like it took Minkowski to point it out. Not true.
- Do not overemphasize the differences in how space and time are handled in the lede.
- It is not clear to what extent Einstein understood the geometric implications of his theory in 1905. Your sentence can be misconstrued.
- Lorentz understood his theory as a theory of the "electron". That gets into too much historical give and take, and the lede is not the proper place to disentangle the complex story behind this.
- OK. What's there now seems a decent framework for you to detail-out with your better understanding of the subject matter.
- Allow me to make a suggestion: You had something there regarding Einstein that read: and it would appear that he did not at first think geometrically about spacetime. That runs contrary to everything I've known about special relativity and I couldn't find such a statement in our special relativity article. I suggest that such a statement be avoided unless you truly think it is highly germane in the lede and it is buttressed with a citation to an exceedingly reliable source.
- I can document that statement for you. I'll upload a page to google drive from Bernard F. Schutz's book. It will probably have to be a photograph, since I'm having trouble with my scanner. Stigmatella aurantiaca (talk) 23:07, 28 March 2017 (UTC)
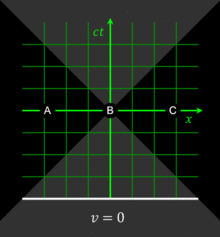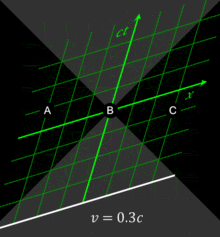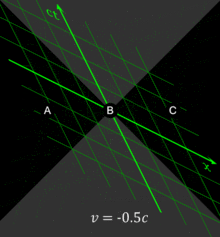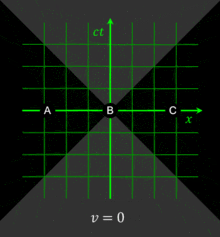
- Also, to help the reader in understanding the distinction between Minkowski space and Einstein's theory of relativity, I lifted the following right out of the article's lede on Minkowski space: In Minkowski's model, the spacetime interval between any two events is independent of the inertial frame of reference in which they are recorded. Don't you think that is the key distinction? Greg L (talk) 21:49, 28 March 2017 (UTC)
- Einstein was at first skeptical of the utility of Minkowski's geometric interpretation, because it didn't tell him anything that he didn't already know, including the fact that combining distance and time produced an invariant. Only later did he realize that the geometric interpretation fundamentally changed the mindset with which he could think through issues, and changed the sort of math that he could use. It is rather doubtful that Einstein could have developed general relativity without adopting the geometric interpretation of his theory. Stigmatella aurantiaca (talk) 23:13, 28 March 2017 (UTC)
- That's quite interesting. Because I think (very) geometrically myself. Two of my patents were geometric solutions to equations of states for gasses. One was a way of reversing-out the equation of state of a gas called sulfur hexafluoride, and the other was a new way of calculating the dew point of water (from relative humidity and temperature) using analog circuits (no microprocessor and lookup tables required).
- So whenever I saw NOVA programs on Einstein's theory of relativity, they often illustrated inertial frames of reference as graphs, like the one at right, and it is these graphs that I vividly remembered. I thought Einstein was responsible for this geometric understanding. If I'm understanding you correctly, Einstein didn't think of it this way.
- In any case, I would rely only upon an exceedingly reliable source for citations buttressing assertions as to what Einstein purportedly did not understand. Greg L (talk) 23:25, 28 March 2017 (UTC)
- Here is a page from Schutz's book, Gravity from the Ground Up.
- https://drive.google.com/file/d/0B8XIf0XcrpOcb2l3ckNfZWxDeHM/view?usp=sharing
- See the second sentence after the section header. I've seen similar statements by other authors, but I can immediately locate this statement because I've based most of my presentation on Schutz. This book, geared towards undergraduates, is an algebra-based text that includes downloadable computer programs to help students visualize topics that would otherwise require calculus. I've also taken a little bit from other, more advanced texts, but Schutz has been my "go to" source because of its unique style of presentation. The book has a web site: http://www.gravityfromthegroundup.org/ I based Fig. 1 and Fig. 5 on figures from this text. I wrote a computer program to draw the hyperbola and axes in Fig 5, hand-lettering the labels. Stigmatella aurantiaca (talk) 03:23, 29 March 2017 (UTC)
I see. You are very knowledgeable about the subject matter at hand. I'm very happy to see someone of your caliber giving some much-needed attention to this article. I agree that Schutz is a reliable source and he indeed wrote “Einstein himself did not at first seem to think geometrically about spacetime.” That is actually quite interesting. I note this text of Einstein's paper: ON THE ELECTRODYNAMICS OF MOVING BODIES. It is rich in formulas and I see no graphs. I can see why Schutz wrote that opinion. So all the graphs of spacetime we see today appear to be modern explanations of Einstein's math-based view of the subject matter?
I think it truly worthwhile mentioning this point somewhere in the article. However, I wouldn't personally use it in the lede because 1) of what it is (opinion of an expert on what Einstein seemed to think), 2) to avoid undo weight, and 3) ledes should be pithy places where this sort of nuance would be a bit premature and out of place. I suggest you write this little nugget on a card, stick it to your cork board, and use it somewhere in the Introduction section, near the fourth paragraph. Greg L (talk) 04:28, 29 March 2017 (UTC)
- Sounds good.
- By the way, please don't exaggerate my degree of expertise. I'm an amateur with an strong interest in the history of science. My degree is most definitely not in physics. I'm very cautious in limiting my Wikipedia contributions to things that I am reasonably knowledgeable about. :-)
- Stigmatella aurantiaca (talk) 05:05, 29 March 2017 (UTC)
These theories nonetheless successfully explained coordinate frames for observers moving at different velocities.
Regarding the recent edit removing: These theories nonetheless successfully explained coordinate frames for observers moving at different velocities. As well as I know it, and that might not be very well, the Lorentz transformation came from Maxwell's equations. Maxwell's equations are not Galilean invariant, and so it was an interesting question about what invariance they did have. But I don't know the history all that well. I suspect, though, that at the time Lorentz didn't expect his transformation to become famous. Gah4 (talk) 22:20, 28 March 2017 (UTC)
- I'm certainly no expert, but I look forward to the lede being crystal clear, entirely germane, sufficiently complete, and totally true so anyone can do a drive-by reading on the subject and leave with a basic understanding of spacetime and how various scientists contributed to our current understanding of it. Greg L (talk) 23:10, 28 March 2017 (UTC)
- Here is a page from Arthur I. Miller's "Albert Einstein's Special Theory of Relativity".
- https://drive.google.com/open?id=0B8XIf0XcrpOcTTRKZzBnQTFUcVU
- I'm sorry that I couldn't find a less technical reference, but basically (first paragraph, top of page 66) Lorentz was not seeking a theory of space/time/relativity, but rather was seeking a theory of a deformable electron that would explain a host of weird experimental results: the Trouton–Noble experiment, the Experiments of Rayleigh and Brace, the Kaufmann–Bucherer–Neumann experiments, the Fizeau experiment etc. quickly come to mind, in addition to (of course!) the Michelson–Morley experiment. His theory was not completely compatible with Maxwell's equations. Note Equation 1.178 near the bottom of page 67, which violates conservation of charge in reference systems other than the electron's rest system.
- On the other hand, Poincaré starting from Lorentz's formulation, corrected him on his various technical errors and came up with something that came very close to anticipating Einstein's interpretation. Poincaré, however, was philosophically indisposed to make the final leap, and remained opposed to Einstein's interpretation for the rest of his life. Stigmatella aurantiaca (talk) 04:12, 29 March 2017 (UTC)
- Jeez. You are miles ahead of me. I'll check in periodically. I think all I can do is offer a little bit of editing help you to ensure your text meat & potatoes, is editorial-wise, as accessible as possible given the advanced subject matter. I think the lede looks really good right now; even I understand it (I think). Greg L (talk) 04:41, 29 March 2017 (UTC)
Yes. I am not so sure how ready Einstein was to accept his own theory, but it does seem that others that could have done it weren't ready to go that far. Gah4 (talk) 09:52, 30 March 2017 (UTC)
- Einstein "simply cared far more than most of his colleagues that the laws of physics have to explain everything in nature coherently and consistently. As a result he was acutely sensitive to flaws and contradictions in the logical structure of physical theories."[1] As a result of his sense of rightness, Einstein, almost immediately after the publication of his 1905 paper, began a decade-long quest for a theory which would answer defects that he perceived in his special theory. These efforts culminated in the general theory of relativity. Yet even after publication of the general theory, Einstein was not happy, and he spent the rest of his life in quest of a unified theory. Stigmatella aurantiaca (talk) 10:16, 30 March 2017 (UTC)
References
- ^ Smolin, Lee. "Einstein's Legacy -- Where are the "Einsteinians?"". Logos Journal. Princeton University Press. Retrieved 30 March 2017.
Role of the Michelson-Morley experiment
I removed the following words from the lede:
The Michelson–Morley experiment showed that light traveled through space at precisely one fixed speed regardless of an observer's velocity towards or away from a light source. This fact had far-ranging implications for physics as it required that for two observers traveling at different relative speeds, particularly if that speed difference was a significant fraction of the speed of light, each observer would see the other's clock as ticking more slowly than their own. Furthermore, each observer would also see the other's lengths along the apparent velocity vector to be shorter than their own.
Issues:
- 1. MMX does not inevitably imply constant speed of light.
- a. The length contraction hypothesis was an attempt to preserve commonsense notions of the additivity of velocities and the concept of the stationary aether.
- b. In 1908, Walther Ritz proposed an alternative theory which satisfied the MMX, partly in opposition to relativity, but mostly in opposition to what he perceived as general defects in Maxwell's theory. One feature of his theory was that light is emitted at speed c relative to its source instead of following the invariance postulate. Ritz's theory is considered an emission theory, although in contrast to most such theories (which, starting with Newton, envision a corpuscular nature to light), Ritz's theory is a wave theory.
- c. What Michelson–Morley experiment did show was that within tight limits, within an inertial frame, the two-way speed of light is isotropic and independent of the closed path considered.
- 2. The excerpt overstates the importance of MMX in the historical development of relativity. When questioned about it in later years, Einstein was not sure whether he was even aware of MMX when he developed special relativity. In an interview with Einstein, Robert S. Shankland reported the following, in which Einstein emphasized the importance of the Fizeau experiment:
He continued to say the experimental results which had influenced him most were the observations of stellar aberration and Fizeau's measurements on the speed of light in moving water. "They were enough," he said.
- To the above, as evidenced by his 1905 paper, we can add the moving magnet and conductor problem.
- Stigmatella aurantiaca (talk) 09:44, 30 March 2017 (UTC)
- Hmmm... The article on the moving magnet and conductor problem implies that the problem was well-known in the 19th century. It certainly would have been a well-known observation, but to the best of my knowledge, nobody before Einstein considered it to be a problem that needed to be resolved. One way or another, this, stellar aberration, and the Fizeau experiment were far more important to Einstein than the MMX. Stigmatella aurantiaca (talk) 10:46, 30 March 2017 (UTC)
- Yes, as above, many people had idea, but weren't quite ready to claim them as agreeing with nature. Aether drag either partial or full, were also considered as theories that would give a small or no difference in Michelson-Morley. The choice was not only Newton vs. Einstein. Gah4 (talk) 16:41, 30 March 2017 (UTC)
- @ Stigmatella Very well. What I added kept my mind busy with whether it was fully and truly correct. But consider this: My read on this is that the set of Lorentz transformations was advanced to explain differences in observed time intervals for different observers. Also, the Lorentz–FitzGerald contraction was, of course, advanced to explain length changes for different observers. Are you saying these two ad hoc theories failed to explain that both time and length appeared different for different observers? Do you think the verbiage you removed falsely implied that both theories swept both adjustments together as a big “ah HA”?
- I don't need an expansive answer that requires way too much of your time to the above. Just consider the question and affirm that your removal of the text was appropriate in light of my questions.
- In 1889, FitzGerald proposed length contraction as a means of explaining MMX. If you read FitzGerald's paper, you will notice that for some reason he didn't give an exact expression for the magnitude of the contraction, although I imagine that he could have. Lorentz came up with the same idea independently in 1892, giving it an exact expression. For mathematical consistency, Lorentz found it necessary to introduce the concept of "local time" . Lorentz assumed that length contraction represented an actual physical contraction. On the other hand, Lorentz considered local time to be nothing more than a mathematical fiction.
- Most physicists believed that Lorentz contraction would be detectable by such experiments as the Trouton–Noble experiment or the Experiments of Rayleigh and Brace. These gave negative results, and in his 1904 theory of the electron, Lorentz was able to explain these negative results as an inevitable consequence of his transforms. However, due to an error in his analysis, he still considered that the aether should be detectable in a properly designed experiment.
- Overall, it should be apparent that Lorentz and his followers were far from understanding the meaning of the Lorentz transforms as was to be elucidated by Einstein.
- Poincaré saw much more than a mere mathematical trick in Lorentz's formulation of local time, and demonstrated the non-detectability of the aether. He came very, very close to deriving relativity, but failed at the final hurdle.
- To make a long story short, the correct interpretation of the MMX required decades of analysis. We can apply hindsight to conclude what you stated in the excerpt. But fin de siècle scientists didn't interpret the MMX the way that you did. Hindsight is a wonderful thing.
- Stigmatella aurantiaca (talk) 23:04, 30 March 2017 (UTC)
- Different subject: I've spent some time reading Twin paradox. Do you have your mind wrapped around that concept perfectly well? I can't help but think that an astronaut, lifting off from earth and going into orbit, would see earth clock transmissions to him [disclaimer] as running slow compared to his onboard clock. And of course, just like GPS satellite clocks, earth would see his clock signals beamed to earth as running too slow due to his relative velocity (as well as him being further from earth's center in a lesser gravity field). This would certainly be the case for two observers who had never shared the same inertial frame of reference at the beginning (before launch) and end (after landing), such as earthlings and say, a hypothetical Vulcan passing by; both would see each other's clocks as running slowly compared to their own. I would love to see a Minkowski spacetime diagram showing how the astronaut case differs from the passing alien.
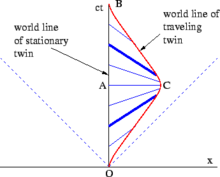
I don't see a good ready-made file to grab from Commons to match your description, but for the simple case of a twin going straight out and turning back, there is nothing puzzling about the twin paradox at all. The proper time measured along the path from O to C to B is less than from O to A to B. Simple?
I didn't want to go into length contraction and the twin paradox in my introduction, but it seems that I should. I suppose that I'll need another three figures. None of the illustrations in Commons are 100% what I want, but I could do with 75%.
Stigmatella aurantiaca (talk) 00:31, 31 March 2017 (UTC)
- The reason I want to understand the Twin Paradox is it seems central to understanding the naming of Einstein's frame of reference: inertial frame of reference. It appears that accelerating to a new frame of reference to make some protracted measurements and then decelerating to the original frame of reference to compare clocks is an exceedingly different thing from the passing alien. I've long wrestled with this. Greg L (talk) 16:47, 30 March 2017 (UTC)
- There are now clocks good enough that you can lift one 1ft (0.3m) and fairly easily measure the difference due to the change in g. That is, two clocks side by side, then lift one. Also, when GPS was originally designed, the designers didn't know if they needed to do the general relativity correction, so they added a switch for it. Fairly soon, it was found that the correction was needed, and the switch turned on. Gah4 (talk) 17:15, 30 March 2017 (UTC)
@ Stigmatella aurantiaca. Thank you very much for the thoughtful replies. Now I understand your wise deletion; “ad hoc” is almost a complement. As for adding the twin paradox to the Introduction section, May the Force Be With You, for I haven't yet seen a detailed explanation—anywhere—that is accessible to a general-interest readership. Perhaps something along the lines of “It may seem like there's a paradox, but there ain’t.” The detailed explanation for why it ain't so should go even further down the article, in my opinion. It's easy to ralph technical jargon onto Wikipedia's pages so wikipedians seem all smart-smart. Explaining technical stuff so that most people can easily understand the basic ‘Ah HA’ on their first pass is double-tough. Doing so on matters pertaining to relativity is crazy hard. Greg L (talk) 03:26, 31 March 2017 (UTC)
- Explaining things like the twin paradox is much easier from the geometric point of view. There is really only one postulate in the spacetime explanation of relativity: The geometry of the universe (in the absence of gravity) is that of Minkowski spacetime, where the invariant interval is given by
- We have grown up having a Euclidean intuition about the universe because spacetime is a manifold, and the scale factor relating space with time is a huge number compared with anything going on in our normal human experience.
- To be more precise, we can quote from Metric tensor (general relativity):
- "Mathematically, spacetime is represented by a 4-dimensional differentiable manifold M and the metric is given as a covariant, second-order, symmetric tensor on M, conventionally denoted by g. Moreover, the metric is required to be nondegenerate with signature (-+++). A manifold M equipped with such a metric is a type of Lorentzian manifold."
- It should be evident that the above scary words really break down to saying the same thing.
- We can contrast the geometric interpretation of spacetime against Einstein's original kinematic development, which began with two postulates, the second of which is a little difficult to swallow, at least as customarily taught today.[1]
- Stigmatella aurantiaca (talk) 06:18, 31 March 2017 (UTC)
References
- ^ To quote from https://phys.org/news/2006-03-relativity.html: “In 1905, Einstein made two plausible assumptions,” says Baierlein. “He assumed, first, that the laws of physics are the same in all uniformly moving frames of reference and, second, that the motion of the source of light has no effect on the speed of light itself. The second assumption is what he called ‘the constancy of the speed of light.’ ” ... In many current textbooks, however, the assumption is simply made that all uniformly moving observers measure the same speed of light. On top of that, the authors imply that Einstein made that assumption. [My boldface] ... “I’m suggesting that some of the textbook writers go back and see how Einstein actually derived his results. Instead of assuming that all observers measure the same speed for light, it is better to start with a plausible assumption that light operates independently of its source. If you do this, you set up something that is easier to believe.”
- @Stigmatella aurantiaca: I think Miranda Marquit of Phys.org is semi-correct but there is no real distinction. One need look no further than what Einstein himself wrote in 1905. I often find that in technical and science papers, the papers’ abstracts are good nuggets on what is going on in the authors’ minds. Einstein wrote as follows (with my blanking of less relevant material):
- Use the following syntax to ping: "{{reply to|Username}}". For example, "{{Reply to|Stigmatella aurantiaca}}" generates "@Stigmatella aurantiaca:" including @ sign and colon. "@Stigmatella aurantiaca:" doesn't work. Stigmatella aurantiaca (talk) 10:05, 2 April 2017 (UTC)
| “ | …the same laws of electrodynamics and optics will be valid for all frames of reference for which the equations of mechanics hold good. We will raise this conjecture (the purport of which will hereafter be called the “Principle of Relativity”) . . . . that light is always propagated in empty space with a definite velocity c which is independent of the state of motion of the emitting body. | ” |
- I once read that Einstein, when first pondering the conundrum, imagined himself riding on a light beam and envisioned what one would experience. In his paper, he wrote that light's speed in space is irrespective of the motion of the emitting body; ergo, Miranda Marquit is correct. However, Einstein obviously understood that when it comes to the speed of light, everything is relative: the speed of light is also independent of the state of motion of the observing body; ergo, there doesn't seem to be a practical distinction and I don't get what Miranda is harping about. Greg L (talk) 16:48, 1 April 2017 (UTC)
- Marquit is a science reporter who was covering Baierlein's critque of current pedagogical practice in the teaching of special relativity. He wasn't reporting on his own thoughts.
- It took a bit of subtle reasoning in Einstein's 1905 paper to get from "light is always propagated in empty space with a definite velocity c which is independent of the state of motion of the emitting body" to "all uniformly moving observers measure the same speed of light." A lot of students just don't "get" the reasoning, but go away thinking that Einstein is somehow using circular reasoning. He isn't, of course. Because of this difficulty, most elementary textbooks (and by far the great majority of popular expositions) go straight to the stronger (and weirder) statement of the second postulate in order to spare the reader having to go through this reasoning. For example, in my old (1988) edition of Halliday and Resnick, I read, "The Postulate of the Speed of Light. The speed of light in free space has the same value c in all directions and in all inertial reference frames." On the Hyperphysics website, I read, "The velocity of light is independent of source or detector velocity - a universal constant."
- Such misstatements of the second postulate are rampant. The distinction is quite important from a pedagogical standpoint because the original weak version of the 2nd postulate is very easy to accept, since most students understand about water waves, sound waves etc. On the other hand, the strong version of the 2nd postulate is just plain weird.
- Wikipedia is relatively free of misstatements of the second postulate only because of the efforts of a small group of Wikipedia editors (of whom I was one) who worked collaboratively to improve the coverage of special relativity several years ago. Mostly we worked on the experimental basis of special relativity, but things like correcting misstatements of the 2nd postulate were just part of what we did.
- As an example of our work, check out the article on MMX. Although the 14:15, 26 March 2012 version of Michelson–Morley experiment didn't misstate the 2nd postulate, compare that terrible version with the 10:16, 17 December 2013 version after User:D.H and I finished a twenty-month collaboration. D.H was the real science expert in the collaboration, while I provided multiple illustrations (including animated) and added historical facts including corrections to the sections on Miller's, Kennedy's, Illingworth's, and Joos's repetitions of the MMX.
I carefully read each point of your response and appreciate it all. Very thoughtful. Some of my take-aways are as follows:
- You and the wikipedians you are collaborating with are doing paradigm-example work on Wikipedia.
- I actually very much enjoyed reading your 2013 version of article on the Michelson–Morley experiment, which is a rare experience for me since I find that many of Wikipedia's technical articles are written in a pompous style (like they were written by Cardinal Bellarmine), tedious (“we’re not even gonna parenthetically explain that obscure specialty-lingo word, you gotta Click To Learn©™® peon”), and flawed.
- Upon reading that Michelson and Morley were floating their optical benches on pools of mercury in highly isolated rooms in basements, I went in search of what those guys died of. I couldn't find anything like “he died crapping his pants at a memory-care facility,” but I did see that “Michelson suffered a nervous breakdown in September 1885,” which he ascribed “to the intense work . . . . during the preparation of the experiments.”
- As for why I find Wikipedia's technical articles so tedious and flawed, the true experts by and large steer far clear of Wikipedia. While I was slaving away on articles like our Thermodynamic temperature and Fuzzball (string theory), I actually emailed and spoke over the phone with the Ph.D.s I was citing. Without exception, they were nearly aghast and could not fathom the moronic concept of wanting to edit on Wikipedia, where a 14-year-old kid carries as much editorial standing as a 45-year-old Ph.D. who wrote a peer reviewed paper. I completely relate. I have 17–18 patents and probably more than half of them pertain to PEM fuel cells. Believe it or not, I have never bothered to even look (literally) at the Wikipedia article on PEM fuel cells. It would without any doubt, be a profoundly frustrating experience. Nothing to learn… too much to fix… too many 14-year-old kids flush with excitement at their powers of insight. Indeed, I edit primarily upon articles I want to better learn; having to do research and write what amounts to a masters thesis on a subject is an outstanding way to learn something as well as hone one's technical writing skills. It appears you are doing the same.
- I really like what this article has become.
- Now I better understand the subject matter.
Greg L (talk) 16:01, 2 April 2017 (UTC)
Attempting some reorganization
I am going to attempt some reorganization so that the article progresses from simpler to more complex material, doing as little deletion as possible. To this end, I am going to use this space to temporarily "park" material that I don't know what to do with at the moment. I will return as much of this deleted material back into the main article as I can. Please bear with me.
Temporarily "parked" material follows:
Spacetime was described as an affine space with quadratic form in Minkowski space of 1908.[1] In his 1914 textbook The Theory of Relativity, Ludwik Silberstein used biquaternions to represent events in Minkowski space. He also exhibited the Lorentz transformations between observers of differing velocities as biquaternion mappings. Biquaternions were described in 1853 by W. R. Hamilton, so while the physical interpretation was new, the mathematics was well known in English literature, making relativity an instance of applied mathematics.
Description of the effect of gravitation on space and time was found to be most easily visualized as a "warp" or stretching in the geometrical fabric of space and time, in a smooth and continuous way that changed smoothly from point-to-point along the spacetime fabric. In 1947 James Jeans provided a concise summary of the development of spacetime theory in his book The Growth of Physical Science.[2]
Marcel Proust, in his novel Swann's Way (published 1913), describes the village church of his childhood's Combray as "a building which occupied, so to speak, four dimensions of space—the name of the fourth being Time".
In physical cosmology, the concept of spacetime combines space and time to a single abstract universe.
- Ehrenfest, Paul (1920) "How do the fundamental laws of physics make manifest that Space has 3 dimensions?" Annalen der Physik 366: 440.
- Kant, Immanuel (1929) "Thoughts on the true estimation of living forces" in J. Handyside, trans., Kant's Inaugural Dissertation and Early Writings on Space. Univ. of Chicago Press.
- Robb, A. A. (1936). Geometry of Time and Space. University Press.
- Wells, H.G. (2004). The Time Machine. New York: Pocket Books. ISBN 0-671-57554-6. (pp. 5–6)
- Isenberg, J. A. (1981). "Wheeler–Einstein–Mach spacetimes". Phys. Rev. D. 24 (2): 251–256. Bibcode:1981PhRvD..24..251I. doi:10.1103/PhysRevD.24.251.
- Tangherlini, F. R. (1963). "Schwarzschild Field in n Dimensions and the Dimensionality of Space Problem". Nuovo Cimento. 14 (27): 636.
- Erwin Schrödinger (1950) Space–time structure. Cambridge Univ. Press.
- Schutz, J. W. (1997). Independent axioms for Minkowski Space–time. Addison-Wesley Longman. ISBN 0-582-31760-6.
Stigmatella aurantiaca (talk) 11:00, 24 March 2017 (UTC)
Until the beginning of the 20th century, time was believed to be independent of motion, progressing at a fixed rate in all reference frames; however, following its prediction by special relativity, later experiments confirmed that time slows at higher speeds of the reference frame relative to another reference frame. Such slowing, called time dilation, is explained in special relativity theory. Many experiments have confirmed time dilation, such as the relativistic decay of muons from cosmic ray showers and the slowing of atomic clocks aboard a Space Shuttle relative to synchronized Earth-bound inertial clocks.[3] The duration of time can therefore vary according to events and reference frames.
Stigmatella aurantiaca (talk) 09:17, 25 March 2017 (UTC)
The points of spacetime correspond to physical events. In a local coordinate system whose domain is an open set of the spacetime manifold, three spacelike coordinates and one timelike coordinate typically emerge. Dimensions are independent components of a coordinate grid needed to locate a point in a certain defined "space". For example, on the globe the latitude and longitude are two independent coordinates which together uniquely determine a location. In spacetime, a coordinate grid that spans the 3+1 dimensions locates events (rather than just points in space), i.e., time is added as another dimension to the coordinate grid. This way the coordinates specify where and when events occur. However, the unified nature of spacetime and the freedom of coordinate choice it allows, imply that to express the temporal coordinate in one coordinate system requires both temporal and spatial coordinates in another coordinate system. Unlike in normal spatial coordinates, there are still restrictions for how measurements can be made spatially and temporally (see Spacetime intervals). These restrictions correspond roughly to a particular mathematical model which differs from Euclidean space in its manifest symmetry.
When dimensions are understood as mere components of the grid system, rather than physical attributes of space, it is easier to understand the alternative dimensional views as being simply the result of coordinate transformations.
Stigmatella aurantiaca (talk) 00:46, 26 March 2017 (UTC)
I'm not going to bother trying to find places for the material above. I'm reminded of the fairy tale "Stone Soup". I was originally just going to do a bit of rearrangement in the Introduction, but kept finding myself needing to add some onions, carrots, meat, turnips etc. etc. to the soup to make it just a little bit tastier... ![]()
Stigmatella aurantiaca (talk) 13:20, 26 March 2017 (UTC)
Potatoes are a New World crop, and do not belong in a traditional telling of "Stone Soup".
Stigmatella aurantiaca (talk) 13:31, 26 March 2017 (UTC)
References
- ^ Minkowski, Hermann (1909), , Physikalische Zeitschrift, 10: 75–88
- Various English translations on Wikisource: Space and Time.
- ^ "The Growth Of Physical Science". Archive.org. 2010-07-21. Retrieved 2016-12-17.
- ^ Ashby, Neil (2003). "Relativity in the Global Positioning System" (PDF). Living Reviews in Relativity. 6: 16. doi:10.12942/lrr-2003-1.
{{cite journal}}: CS1 maint: unflagged free DOI (link)
Parking deleted section
I deleted the "Basic concepts" section. Being a pack rat, I am parking the deleted material here to see what I can salvage.
I regenerated an anchor to "Spacetime intervals" as an alternative anchor to "Spacetime interval" in the introduction.
Stigmatella aurantiaca (talk) 09:53, 11 April 2017 (UTC)
==Basic concepts== The basic elements of spacetime are events. In any given spacetime, an event is a unique position at a unique time. Because events are spacetime points, an example of an event in classical relativistic physics is <math>(x,y,z,t)</math>, the location of an elementary (point-like) particle at a particular time. A spacetime itself can be viewed as the union of all events in the same way that a line is the union of all of its points, formally organized into a [[manifold]], a space which can be described at small scales using coordinate systems. Spacetime is independent of any [[Observer (special relativity)|observer]].<ref>{{cite book| last = Matolcsi| first = Tamás| title = Spacetime Without Reference Frames| publisher = Akadémiai Kiadó| year= 1994| location = Budapest}}</ref> However, in describing physical phenomena (which occur at certain moments of time in a given region of space), each observer chooses a convenient metrical [[coordinate system]]. Events are specified by four [[real numbers]] in any such coordinate system. The trajectories of elementary (point-like) particles through space and time are thus a continuum of events called the [[world line]] of the particle. Extended or composite objects (consisting of many elementary particles) are thus a union of many world lines twisted together by virtue of their interactions through spacetime into a "world-braid". However, in physics, it is common to treat an extended object as a "particle" or "field" with its own unique (e.g., center of mass) position at any given time, so that the world line of a particle or light beam is the path that this particle or beam takes in the spacetime and represents the history of the particle or beam. The world line of the orbit of the Earth (in such a description) is depicted in two spatial dimensions ''x'' and ''y'' (the plane of the Earth's orbit) and a time dimension orthogonal to ''x'' and ''y''. The orbit of the Earth is an [[ellipse]] in space alone, but its world line is a [[helix]] in spacetime.<ref>{{cite book|last1=Ellis|first1=George F.R.|last2=Williams|first2=Ruth M.|last3=Carfora|first3=Mauro|title=Flat and Curved Space-times|date=2000|publisher=Oxford University Press|location=Oxford|isbn=0198506570|edition=2nd|url=https://books.google.com/books?id=LKfvAAAAMAAJ|accessdate=30 January 2017}}</ref> The unification of space and time is exemplified by the common practice of selecting a metric (the measure that specifies the [[Interval (mathematics)|interval]] between two events in spacetime) such that all four dimensions are measured in terms of [[Units of measurement|units]] of distance: representing an event as <math>(x_0,x_1,x_2,x_3) = (ct,x,y,z)</math> (in the Lorentz metric) or <math>(x_1,x_2,x_3,x_4) = (x,y,z,ict)</math> (in the original Minkowski metric) where <math>c</math> is the [[speed of light]].<ref>{{cite book|last1=Petkov|first1=Vesselin|title=Minkowski Spacetime: A Hundred Years Later|date=2010|publisher=Springer|location=Dordrecht, Netherlands|isbn=9048134749|page=70|url=https://books.google.com/?id=trlsrl4mF3YC|accessdate=30 January 2017}}</ref> The metrical descriptions of [[Minkowski Space]] and spacelike, lightlike, and timelike intervals given below follow this convention, as do the conventional formulations of the [[Lorentz transformation]]. ==={{vanchor|Spacetime intervals}} in flat space=== <!-- This section is linked from [[Lorentz transformation]] --> <!-- This Anchor tag serves to provide a permanent target for incoming section links. Please do not move it out of the section heading, even though it disrupts edit summary generation (you can manually fix the edit summary before you save your changes). Please do not modify it, even if you modify the section title. It is always best to anchor an old section header that has been changed so that links to it won't be broken. See [[Template:Anchor]] for details. This template is {{subst:Anchor comment}} --> In a [[Euclidean space]], the separation between two points is measured by the distance between the two points. The distance is purely spatial, and is always positive. In spacetime, the [[4-position#Four-position|displacement four-vector]] Δ''R'' is given by the space [[displacement vector]] Δ''r'' and the time difference Δ''t'' between the events. The ''spacetime interval'', also called ''invariant interval'', between the two events, ''s''<sup>2</sup>,<ref group=note>Note that the term ''spacetime interval'' is applied by several authors to the quantity ''s''<sup>2</sup> and not to ''s''. The reason that the quantity ''s''<sup>2</sup> is used and not ''s'' is that ''s''<sup>2</sup> can be positive, zero or negative, and is a more generally convenient and useful quantity than the Minkowski norm with a timelike/null/spacelike distinguisher: the pair ({{sqrt|{{abs|''s''<sup>2</sup>}}}}, sgn(''s''<sup>2</sup>)). Despite the notation, it should not be regarded as the square of a number, but as a symbol. The cost for this convenience is that this "interval" is quadratic in linear separation along a straight line.</ref> is defined as: :<math>s^2 = \Delta r^2 - c^2\Delta t^2 \,</math> (spacetime interval), where ''c'' is the speed of light. The choice of signs for <math>s^2</math> above follows the [[Signature convention|space-like convention (−+++)]].<ref group=note>More generally the spacetime interval in flat space can be written as <math> s^2= g_{\alpha\beta}\Delta x^\alpha \Delta x^\beta </math> with [[metric tensor]] ''g'' independent of spacetime position.</ref> Spacetime intervals may be classified into three distinct types, based on whether the temporal separation (<math>c^2 \Delta t^2</math>) is greater than, equal to, or smaller than the spatial separation (<math>\Delta r^2</math>), corresponding to time-like, light-like, or space-like separated intervals, respectively. Certain types of [[world line]]s are called [[geodesic]]s of the spacetime – straight lines in the case of Minkowski space and their closest equivalent in the curved spacetime of general relativity. In the case of purely time-like paths, geodesics are (locally) the paths of greatest separation (spacetime interval) as measured along the path between two events, whereas in Euclidean space and Riemannian manifolds, geodesics are paths of shortest distance between two points.<ref group=note>This characterization is not universal: both the arcs between two points of a [[great circle]] on a sphere are geodesics.</ref><ref>{{cite book|last1=Berry|first1=Michael|title=Principles of Cosmology and Gravitation|date=1989|publisher=A. Hilger|location=Bristol, England|isbn=0852740379|page=58|edition=1st|url=https://books.google.com/books?id=wTvFxXguod0C|accessdate=30 January 2017}}</ref> The concept of geodesics becomes central in [[general relativity]], since geodesic motion may be thought of as "pure motion" ([[Fictitious force|inertial motion]]) in spacetime, that is, free from any external influences. ====Time-like interval==== :<math>\begin{align} \\ c^2\Delta t^2 &> \Delta r^2\\ s^2 &< 0 \\ \end{align}</math> For two events separated by a time-like interval, enough time passes between them that there could be a cause–effect relationship between the two events. For a particle traveling through space at less than the speed of light, any two events which occur to or by the particle must be separated by a time-like interval. Event pairs with time-like separation define a negative spacetime interval (<math>s^2 < 0</math>) and may be said to occur in each other's future or past. There exists a [[Frame of reference|reference frame]] such that the two events are observed to occur in the same spatial location, but there is no reference frame in which the two events can occur at the same time. The measure of a time-like spacetime interval is described by the [[proper time interval]], <math>\Delta\tau</math>: :<math>\Delta\tau = \sqrt{\Delta t^2 - \frac{\Delta r^2}{c^2}}</math> (proper time interval). The proper time interval would be measured by an observer with a clock traveling between the two events in an [[inertial]] reference frame, when the observer's path intersects each event as that event occurs. (The proper time interval defines a [[real number]], since the interior of the square root is positive.) ====Light-like interval==== :<math>\begin{align} c^2\Delta t^2 &= \Delta r^2 \\ s^2 &= 0 \\ \end{align}</math> In a light-like interval, the spatial distance between two events is exactly balanced by the time between the two events. The events define a spacetime interval of zero (<math>s^2 = 0</math>). Light-like intervals are also known as "null" intervals. Events which occur to or are initiated by a [[photon]] along its path (i.e., while traveling at <math>c</math>, the speed of light) all have light-like separation. Given one event, all those events which follow at light-like intervals define the propagation of a [[light cone]], and all the events which preceded from a light-like interval define a second (graphically inverted, which is to say "''pastward''") light cone. ====Space-like interval==== :<math>\begin{align} \\ c^2\Delta t^2 &< \Delta r^2 \\ s^2 &> 0 \\ \end{align}</math> When a space-like interval separates two events, not enough time passes between their occurrences for there to exist a [[causal]] relationship crossing the spatial distance between the two events at the speed of light or slower. Generally, the events are considered not to occur in each other's future or past. There exists a [[Frame of reference|reference frame]] such that the two events are observed to occur at the same time, but there is no reference frame in which the two events can occur in the same spatial location. For these space-like event pairs with a positive spacetime interval (<math>s^2 > 0</math>), the measurement of space-like separation is the [[proper distance]], <math>\Delta\sigma</math>: :<math>\Delta\sigma = \sqrt{s^2} = \sqrt{\Delta r^2 - c^2\Delta t^2}</math> (proper distance). Like the proper time of time-like intervals, the proper distance of space-like spacetime intervals is a real number value. ===Interval as area=== The interval has been presented as the [[area]] of an oriented [[rectangle]] formed by two events and [[isotropic line]]s through them. Time-like or space-like separations correspond to oppositely [[orientation (vector space)|oriented]] rectangles, one type considered to have rectangles of negative area. The case of two events separated by light corresponds to the rectangle degenerating to the segment between the events and zero area.<ref>{{cite book|last1=Yaglom|first1=I.M.|last2=Shenitzer|first2=Abe|title=A Simple Non-Euclidean Geometry and Its Physical Basis: An Elementary Account of Galilean Geometry and the Galilean Principle of Relativity|date=1979|publisher=Springer-Verlag|location=New York|isbn=0387903321|edition=1st}}</ref> The transformations leaving interval-length invariant are the area-preserving [[squeeze mapping]]s. The parameters traditionally used rely on quadrature of the hyperbola, which is the [[natural logarithm]]. This transcendental function is essential in mathematical analysis as its inverse unites [[circular function]]s and [[hyperbolic function]]s: The [[exponential function]], e<sup>''t''</sup>, ''t'' a real number, used in the [[hyperbola]] (e<sup>''t''</sup>, e<sup>–''t''</sup> ), generates [[hyperbolic sector]]s and the [[hyperbolic angle]] parameter. The functions cosh and sinh, used with [[rapidity]] as hyperbolic angle, provide the common representation of squeeze in the form <math>\begin{pmatrix}\cosh \phi & \sinh \phi \\ \sinh \phi & \cosh \phi \end{pmatrix},</math> or as the [[split-complex number|split-complex unit]] <math>e^{j \phi} = \cosh \phi \ + j \ \sinh \phi .</math> ===Generalized spacetime (extra dimensions === {{main|extra dimensions}} The term ''spacetime'' has taken on a generalized meaning beyond treating spacetime events with the normal 3+1 dimensions{{clarify|what is that meaning?|date=March 2017}}. Other{{which|date=March 2017}} proposed spacetime theories include additional dimensions—normally [[spatial dimension|spatial]] but there exist some{{which|date=March 2017}} theories that include additional temporal dimensions, and even some, such as [[superspace]] of [[supersymmetric theories]], that include dimensions that are neither temporal nor spatial. The number of dimensions required to describe the universe is still an [[open question in physics|open question]]{{why|date=March 2017}}. Theories such as [[string theory]] predict 10 or 26 dimensions (with [[M-theory]] predicting [[11-dimensional spacetime|11 dimensions]]{{why|date=March 2017}}: 10 spatial and 1 temporal). The existence of more than four dimensions only appears to make a difference at [[subatomic scale]]s.{{why|date=March 2017}}<ref>{{cite book|last1=Kopeikin|first1=Sergei M.|last2=Efroimsky|first2=Michael|last3=Kaplan|first3=George|title=Relativistic Celestial Mechanics of the Solar System|date=2011|publisher=Wiley-VCH|location=Weinheim|isbn=3527634576|url=https://books.google.com/books?id=uN5_DQWSR14C&pg=PT157|accessdate=2016-02-28}}</ref>
| This is an archive of past discussions about Spacetime. Do not edit the contents of this page. If you wish to start a new discussion or revive an old one, please do so on the current talk page. |
| Archive 1 | ← | Archive 5 | Archive 6 | Archive 7 | Archive 8 | Archive 9 | Archive 10 |


