Talk:Impedance matching/Archive 1

This is an archive of past discussions about Impedance matching. Do not edit the contents of this page. If you wish to start a new discussion or revive an old one, please do so on the current talk page.

Archive 1

Archive 2

Archive 3

Merge with Maximum power theorem

This should be merged with Maximum power theorem. - Omegatron 14:39, Jul 29, 2004 (UTC)

Efficiency

If Rload is infinite, it dissipates no power (currrent is zero) so how does this give max efficiency? Or does the efficiency APPROACH 100% as the load resistance approaches infinity. Reminds me of perfect voltage sources and ideal short circuits!!! :)Light current 18:52, 10 August 2005 (UTC)

~~"Efficiency" depends on what you're trying to transfer.~~

Silly me. I didn't even see the Efficiency section; busy at work and such. In this case, efficiency means something very specific.

Yes, the efficiency approaches 100% as the load resistance approaches infinity, as it says in the article: "but tends to 100% as the load resistance tends to infinity". - Omegatron 04:29, August 11, 2005 (UTC)

No echos on audio systems

Even if you called someone very far away, the "echo" would only be a few milliseconds, which is generally considered inaudible. For instance:

(1 000 miles) / ((2 / 3) * c) = 8.05229063 milliseconds

I guess that could be considered an echo, but more accurately would be thought of as a delay-line filter. In reality, the length from source to load will never be that far, anyway; only as far as the nearest telephone exchange.

Regardless, they were originally matched for maximum power, not minimal reflections:

"For the proper operation on long lines, good impedance matching is needed to keep those reflections at minimum. In the real-life telephone subscriber lines the line wire is so short compared to wavelength in the telephone frequencies, that the cables not not have the "true characteristic impedance" on the voice frequencies (few kilometers of able is short line for frequencies below 4 KHz that have 50 km or longer wavelength on cable). The history for 600 ohms is that early telephone system typically used AWG#6 wires spaced 12 inches (305 mm) apart, which made their characteristic impedance exactly 600 ohms at voice frequencies."

"Balanced (for noise rejection) and impedance-matched (for power transfer) transmission lines were clearly necessary for acceptable operation of the early telephone systems, which had no amplifiers. Later, as the telephone network grew, amplifiers, filters and "hybrid" transformers were added to enable long-distance transmission. Proper operation of these components depended critically on rather precise 600 ohms impedances. This 600 ohm impedance is here still nowadays to stay." - [1] - Omegatron 19:02, August 10, 2005 (UTC)

So why are echo supressors used on long distance lines then?? Light current 01:02, 11 August 2005 (UTC)

Please do not delete this from the talk page like it was deleted last time.

I quote from the book "Understanding Telephone Electronics" by John L Fike Ph.D, PE. Adj Professor of Electrical Engineering, Southern Methodist University, Staff Consultant , Texas Instruments Learning Center. and George .E. Friend, Consultant, Telecommunications, Dallas, Texas and Staff Consultant, Texas Instruments Learning Center. Chapter 1, page 15.

"The amount of echo delay depends upon the distance from the transmitter to the point of reflection. The effect of the delay on the talker may be barely noticable to very irritating, to downright confusing. Echo also affects the listener on the far end but to a lesser degree. Echos are caused by mismatches in transmission line impedances which usually occur at the hybrid interface between a two wire line and a 4 wire transmission system. The effect of echo is reduced by inserting a loss in the lines." (Italics and bolding all mine). I rest my case, but an admission of error and an apology from Omegatron would be nice. Light current 02:02, 12 August 2005 (UTC)

One aspect of the operation of a hybrid interface that depends critically on a rather precise 600 ohm impedance is the return loss. But like Omegatron said, the length of the TP from the home to the DLC or to the CO is usually not great enough to generate what is usually considered a distinct echo. What I believe he failed to consider is that the mismatch might be at the far end of the call. In the case of an international call, the cumulative round trip delay could be substantial. Alfred Centauri 19:49, 21 August 2005 (UTC)

You're absolutely right. I didn't consider that... — Omegatron 19:29, 24 January 2006 (UTC)

"when a source is driving a load that is far away compared to the wavelength of the energy being sent" can more introductorily be phrased "when the signal changes quickly compared to the time it takes to travel from source to load" since newcomers will not know what "the wavelength of the energy" means. - Omegatron 21:54, August 10, 2005 (UTC)

I'm sorry, I'm not going to cast any more 'pearls' amongst swine (New Testament)Light current 01:22, 11 August 2005 (UTC)

What do you mean? - Omegatron 03:19, August 11, 2005 (UTC)

Ask someone older

Let's try this again: What do you mean? (As in, "how can you be so persistently belligerent while claiming to be cooperative?") - Omegatron 07:39, August 12, 2005 (UTC)

"power transformers are not used for impedance matching but purely voltage transformation"

But why is the voltage transformed higher in the first place? - Omegatron 05:21, August 12, 2005 (UTC)

Ask someone in the electricity supply industry (like I used to be). You still dont want to take my word for anything. Do you?

You'll have to ask much more politely than that if you want an answer.

Which part of my question was not polite?? You're breaching assume good faith all over the place.

Stop responding to every question on every talk page with "go find out for yourself". If you don't know the answer or don't want to provide it, don't reply; someone else will.

It was a somewhat rhetorical question and note to self, anyway, to explore later, and which I see is irrelevant in retrospect. The voltage is kept high so that they can use a small amount of current for the same power output, to minimize resistive losses in the line. The resistive losses are not related to line impedance as far as matching is concerned, though.

I would imagine there are several impedance mismatches between the high voltage lines and residential lines, however. - Omegatron 07:39, August 12, 2005 (UTC)

Sorry, I thought you were asking me, as you seem to move stuff to the talk pages. My (sufficient) answers are provided in the material I write on the article pages. Unfortunately, some people are reluctant to believe me. With reference to my (apparently to you) cryptic comments -- why not try looking them up in a'pedia. I've heard Wikipedia is quite good. Just enter the words in the search box and pick the answer you think fits.Light current 13:22, 12 August 2005 (UTC)

I think you have answered your own question quite correctly. Bad matching does not really have much effect at 50/60 Hz. Now you are starting to think! Light current 22:52, 12 August 2005 (UTC)

Which cryptic comments are you referring to? You really need to try being more cooperative. - Omegatron 14:30, August 12, 2005 (UTC)

Suggestion

I suggest that we move the discussion of dynamical analogies in the two entries above to the discussion at the analogical models page, and maintain a link to analogical models from impedance matching. Anyone strongly disagree? Sholto Maud 22:32, 4 May 2006 (UTC)

I agree!--Light current 23:17, 4 May 2006 (UTC)

It shall be done. Sholto Maud 02:32, 5 May 2006 (UTC)

Engines, road wheels and gearboxes

The discussion about this topic and analogical applications of impedance matching and maximum power may now be found at the Talk:Analogical_models page. Sholto Maud 02:36, 5 May 2006 (UTC)

Sanity check

As I implied in my recent additions to the External links, Hyperphysics says that impedance matching is used in audio:

http://hyperphysics.phy-astr.gsu.edu/hbase/audio/imped.html

As far as I know (and I really should know), impedance matching is never used in audio anywhere except speaker distribution systems, but I just want to make sure I'm not missing something. Maybe it is used somewhere else that would have influenced their page, like alarms or something? Where the output power is more important than the distortion? I doubt it though. (Especially considering the output power would be lower than a regular audio amp in the system they describe.) — Omegatron 19:27, 24 January 2006 (UTC)

Do you remember our conversation about telephones many moons ago? I dont know if you want to call phones 'audio', but we did come to the conclusion that phone systems/lines were matched to stop echoes etc.

Also, are not hi-quality mics passed via 600R cable to 600R inputs on the mixing desks in recording studios? In fact most of the recording studio stuff is 600 R balanced and these circuits are usually matched. Attenuators are 600R etc. I also think that most broadcast audio systems are 600R balanced.

Ref: Audio Systems, Julian L Bernstien. Pub J. Wiley & Sons Inc.1966. Lib of Congress Cat card 66-17326 So you were wise to check your sanity!. Verdict: see a shrink! :-) --Light current 23:07, 24 January 2006 (UTC)

Thanks for putting me down. You're always so pleasant to interact with...

Anyway, yeah, I wasn't including telephones in "audio". I'm talking pro and consumer audio equipment; recording, PA systems, home stereo systems, and whatever.

Impedance matching of microphones like you described isn't done. In the situations it is/was used, it's not really "impedance matching" for maximum power or reflection; just "optimum loading". It definitely deserves mention in the article, though, because of all the confusion it generates.

I don't know about the broadcast stuff. Can you dig up some references? Can you think of anything else? — Omegatron 03:23, 25 January 2006 (UTC)

Balanced microphone technique Im sure is still used especially in studios. Microphones o/ps are transformed to 600R output impedance with transformers to drive the cable which is always 600R screened balanced cable. Im sure youll agree in these case that its sensible to terminate the cable at the studio desk in 600R. Whether this is actually done, I dont know but if I was designing such a system. I would termninate just to be safe (Its only a 6dB voltage loss). Its more important maybe on 'OB' setups where mic cable runs can be a few hundred yards. Again , whether you would notice any difference between terminated and unterminated cables (apart from the level) at audio, Im not sure (depends on cable length). But of course OB audio used to be sent over phone lines so this would make it more necessary to terminate properly. THe only real reference I have at the moment is the one I have given. Its a good book on the basics tho', and you would probably be interested to read it.

Generally speaking though, I dont think matching is that important at audio unless you have long cables (miles)--Light current 04:57, 25 January 2006 (UTC)

Of course balanced microphones are still used. They're not 600 Ω → 600 Ω, though. They use a voltage bridging connection, with maybe 3 kΩ at the input to the mixer and maybe 100 Ω output impedance from the mic.

Matching microphone and pre-amp impedances to the same value (power-matching) reduces both the level and the S/N ratio by 6dB and is not a technique that is used for those reasons. For dynamic and condenser microphones, the preferred preamp input impedance is generally about ten times that of the microphone output; normally around 1.2kO or 2kO. [2]
If a microphone has a low impedance of 200 ohms, a typical mixer mic input or preamplifier should have a low impedance value of between 5 to 10 times or higher that of the mic impedance giving a value of between 1000 and 2000 ohms or higher for best results. [3]
The electronics in the Active Series ribbons provides a perfect load on the ribbon element at all times, meaning that R-122’s are able to deliver 100% of their full sonic potential regardless of the input characteristics of the following mic-pre. Due to the low-impedance output, Active Series mics can also be used with extremely long cable runs with minimal signal loss.

A good impedance match is critical with ribbon microphones because impedance mis-matching “loads” a ribbon improperly, resulting in loss of low end, diminished body, lowered sensitivity and overall compromised performance. With the Active Series ribbons, the ribbon element lives in a perfect world; it sees an optimum impedance match at all times regardless of the following equipment, so its performance will never be compromised by the effects of improper loading. In addition, the ribbon element can’t be damaged by phantom power, electrical glitches or miswired cables. [4]
For audio circuits, is it important to match impedance?

Not any more. In the early part of the 20th century, it was important to match impedance. Bell Laboratories found that to achieve maximum power transfer in long distance telephone circuits, the impedances of different devices should be matched. Impedance matching reduced the number of vacuum tube amplifiers needed which were expensive, bulky, and heat producing.

In 1948, Bell Laboratories invented the transistor - a cheap, small, efficient amplifier. The transistor utilizes maximum voltage transfer more efficiently than maximum power transfer. For maximum voltage transfer, the destination device (called the "load") should have an impedance of at least ten times that of the sending device (called the "source"). This is known as BRIDGING. Bridging is the most common circuit configuration when connecting audio devices. With modern audio circuits, matching impedance can actually degrade audio performance. [5]
Impedance matching went out with vacuum tubes, Edsels and beehive hairdos. Modern transistor and op-amp stages do not require impedance matching. If done, impedance matching degrades audio performance.

Modern solid-state devices transfer voltage between products, not power. Optimum power transfer requires impedance matching. Optimum voltage transfer does not. Today's products have high input impedances and low output impedances. These are compatible with each other. Low impedance output stages drive high impedance input stages. This way, there is no loading, or signal loss, between stages. No longer concerned about the transfer of power, today's low output/high input impedances allow the almost lossless transfer of signal voltages. [6]
It has often been claimed that a 600 Ohm microphone should be matched to a 600 Ohm input for best performance. This is simply wrong, and microphone manufacturers specifications will support me on this. [7]
How about impedance matching in audio applications? There are a few applications where it?s important, but perhaps not as many as you might think. Because audio signals are quite low in frequency, it?s generally only where they have to be sent over quite long cables that transmission-line effects make it necessary to perform impedance matching to prevent reflections. And in most cases, we can get quite efficient signal transfer simply by arranging for the output impedance of our audio source (such as an amplifier) to be much lower than that of our load (such as a loudspeaker). [8]

I believe the only reason a low impedance would be connected to a microphone is as in #3; it's just an optimum load for the mic element so that the transducer can move current around, and is internal to the mic. You still connect it to a high-impedance input.

There might also be something involving vacuum tubes, as implied in #5? I'm not sure why the maximum power theorem would be important there.

We should also mention in the article that sometimes when people say "impedance match" (as in #3), they really mean "optimal impedance", and it refers to a bridging connection. "Matching" up the source and load for best voltage transfer, which doesn't mean making the source and load impedances equal.

What is an "OB" setup? — Omegatron 04:04, 26 January 2006 (UTC)

OB is outside broadcast. Of course the other place matching is used is loudspeakers being matched to the acoustic impedance of the air for high efficiency. For this purpose, large exponential horns were used. You can still se them on some PA bass bins and on hf horns. As regards #5 , I would have thought you still need a transformer to match the hih anode impedance to a low impedance LS.--Light current 16:41, 26 January 2006 (UTC)

Dude, impedance matching is definitely an issue in a professional audio environment! Not so much because of reflections (although those are a problem over longer runs), but more because Low-Z microphones and instrument outputs change their frequency response when connected to higher loads. You lose the characteristic sound of a mic or a pickup when you plug it in to a Hi-Z input. And vice-versa, if you take a Hi-Z output and plug it into an amp that's got a lower input impedance, you drive the amp differently and it sounds different. Admittedly, part of that is because of difference in line levels, but impedance still must be dealt with. There are TONS of products on the market to help recording engineers deal with these problems - DI's, line transformers, etc.

That's not impedance matching. Impedance matching sets the source and load impedances equal. You're using the colloquial definition covered in the Impedance matching#Terminology section. — Omegatron 21:40, 14 September 2006 (UTC)

"L-section"

Is there anywhere a definition of "L-section"??? If not this is quite unclear.

What it really needs is a schematic drawing. I think that would help considerably. I'll add it when I get a chance. Madhu 01:14, 4 November 2006 (UTC)

this should be generalized to impedances, not just resistances. i will do it eventually if no one else does, but i will have to review it. i forget how to match reactive loads. - Omegatron 19:10, Jun 24, 2004 (UTC)

Use a inductive load impedance to match an capacitive source impedances. In general, if the source impedance is (R+jX), you get the best matching with (R-jX), which is called the "complex conjugate". ... How can I say this in the article without making it sound far more complicated than it really is ?

Merging

The other page seems to be about other meanings of the word 'impedance', so Im not sure if a merge is wise. May I suggest a disambiguation page called Impedance matching?--Light current 21:33, 25 July 2006 (UTC)

Impedance mismatch is about impedance matching. It should be in this article. — Omegatron 13:35, 26 July 2006 (UTC)

Yeah but look at all the other crap it contains. What do you suggest doing with all that? 8-?--Light current 15:39, 26 July 2006 (UTC)

Ah. In my edit summary I said "merge the electrical and radio into impedance matching, copy the rest to articles that already exist, delete the essay on humans".

So the computer stuff would be moved to an article about computers, with a link to here, since their use of the term is derived from the electrical, and the human consciousness/communication stuff will be deleted as original research editorialism. — Omegatron 17:47, 26 July 2006 (UTC)

The term "impedance mismatch" is a common software term (different from the electrical meaning). I think it is best to have a "impedance mismatch" article. - Thebithead 15:53, 16 August 2006 (UTC)

Yes, but the software term is borrowed from the electrical term. I think what you are looking for is Object-Relational impedance mismatch. A disambiguation page might not be a bad idea, I suppose. Madhu 21:39, 16 August 2006 (UTC)

While the non-electrical meanings of "impedance mismatch" may have originated from the electrical/radio context, they have taken on distinct meanings of their own. The object-relational impedance mismatch is probably one of the best known, but to eliminate all the other meanings and say that the only non electrical/radio meaning refers to databases is going too far. For example, it's very useful to use the term "impedance mismatch" for human/computer interaction. A user has a mental model of the task they want to perform, and software user interface has a kind of object model in terms of the objects and methods it exposes to do its stuff; the difference between the two is impedance mismatch. The greater the impedance mismatch between a user's mental model and the application's object model, the harder the software is to use for that user. Look at the definition in the entry for Impedance mismatch:

"Impedance mismatch" is derived from the usage of impedance as a measurement of the ability of one system to efficiently accommodate the output (energy, information, etc.) of another. It is used and measured in many ways, but in general the most efficient exchanges between different systems happen when their impedances are closely matched.

That definition PERFECTLY captures the concept I'm talking about.

A good example is Photoshop -- until you augment your own mental model of how to edit images to include concepts like layers, brightness, contrast, hue, saturation, and the like, it's almost impossible to use, but once you do, you're very productive. There's a reason that word processors with a UI that resembles a piece of paper are easier to use than a text editor and a LaTeX processor -- for most people, the impedance mismatch is lower. Regardless, it's different than object-relational impedance mismatch, and "deleting the essay on humans" doesn't solve the problem either. Not defending the current text of Impedance mismatch, but "impedance mismatch" is distinct from "impedance matching." Billbl 03:19, 9 March 2007 (UTC)

I'm not sure how much your examples actually have to do with the physical idea of "impedance matching"/"impedance mismatch". To me it's just the question of having the right mental model of the tools you are using (Photoshop, LaTeX etc.) -- in simple language, just "learning to use the tools". 131.111.8.104 01:10, 4 April 2007 (UTC)

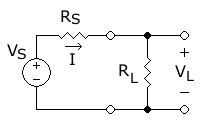
An impedance matching example

In this circuit:

If Rsource = 50 Ω, Vsource = 1 V:

Rload = 1 Ω

Vload = 1/51 * 1 V = 0.0196 V

I = Vsource / Rtotal = 1 V / 51 Ω = 0.0196 A

Powerload = Vload*I = 0.00038 watts

Rload = 50 Ω

Vload = 50/100 * 1 V = 0.5 V

I = Vsource / Rtotal = 1 V / 100 Ω = 0.01 A

Powerload = Vload*I = 0.005 watts

Rload = 100 Ω

Vload = 100/150 * 1 V = 0.666 V

I = Vsource / Rtotal = 1 V / 150 Ω = 0.006666 A

Powerload = Vload*I = 0.0044 watts

Yes, but a more elegant way of showing this is to just use symbols rather than numbers as the relationship is much more clear. For your circuit, find P_L as function of R_L. I'll leave it to the reader to use elementary circuit analysis to arrive at this relationship on their own:

$P_{L}(R_{L})=V_{S}^{2}{\frac {R_{L}}{(R_{S}+R_{L})^{2}}}$

Then, simply take the derivative of P_L with respect to R_L since we're trying to maximize P_L by varying R_L. Using basic calculus, this yields

${\frac {dP_{L}}{dR_{L}}}(R_{L})=V_{S}^{2}\left({\frac {1}{(R_{S}+R_{L})^{2}}}-{\frac {2R_{L}}{(R_{S}+R_{L})^{3}}}\right)$

Now, to find the value of R_L for which the power is a maximum, simply set this derivative equal to zero and solve for R_L. It's trivial to verify that doing so yields only one physically sensible result: R_L=R_S. For the intrepid, you can take the second derivative and verify that this is indeed a maximum and not a minimum. Hence, we conclude precisely (and much more elegantly than the numerical example above) that maximum power is delivered to the load when the resistance of the load matches that of the source. This is analagous to what happens in an AC circuit where impedance is analagous to resistance.Jrdx 21:07, 4 April 2007 (UTC)

Impedance mismatch deletion

I'm confused. Impedance Mismatch redirects to Impedance Matching, but the reference to Impedance Mismatch I followed was from the Cursor (Databases) page. That page suggests that many programming languages suffer from Impedance Mismatch. However, nothing on this page seems to be relevant to programming languages. --Tbannist 16:04, 12 April 2007 (UTC)

Take a look at Object-relational impedance mismatch. It's a colloquial term used in software development. Software developers borrowed this term, although it's more of a conceptual analogy than anything else. Madhu 22:05, 12 April 2007 (UTC)

Impedance mismatch was deleted a few days ago. — Omegatron 23:27, 12 April 2007 (UTC)

Voltage bridging

Moved to Talk:Impedance bridging. — Omegatron 19:49, 13 April 2007 (UTC)

impedance of line equal to that of load??

Re this equation in subsection "Reflectionless or broadband matching": Z_load = Z_line = Z_source

Is the equation correct?
If so, what definition of Z (impedance) is being used here?

If the equation is correct and Z means electrical impedance, then I'm confused. My understanding is that for a pure resistor, impedance is the same as the resistance. Suppose the load is a big resistor and the transmission line is an ordinary transmission line but over quite a short distance. The resistance of a transmission line over a short distance is close to zero. So the impedance of the load would be equal to a big resistance, but the impedance of the transmission line would be near zero. So they would not be equal. Maybe what is meant is "characteristic impedance" or something else rather than "Electrical impedance"? TIA for clarification. --Coppertwig 01:06, 5 January 2007 (UTC)

Yes you need to see characteristic impedance and Transmission line. Even if you have a short length of cable, its input and output impedance with not be zero nor infintiy (remember you are looking across the cable between its input terminals). It will be equal to Zo. This in turn is given by Zo = Sqrt(L/C). where L is the inductance per unit length of the cable and C is the capacitance per unit length of the cable. Come back to me for more clar if needed--Light current 01:33, 5 January 2007 (UTC)

Right. Characteristic impedance is a question of latitude, not longitude. When simple metallic telephone circuits ran across a continent, they had a different longitudinal impedance than a local circuit, but not a different characteristic impedance. I do wonder why there isn't a simpler word for characteristic impedance; the clumsiness of the phrase leads to confusion by elision.

Jim.henderson 03:07, 5 January 2007 (UTC)

There is: 'surge impedance'. This is more descriptive implying that it is the impedance seen under ac conditions or when there is a sudden change in the input voltage.--Light current 18:35, 5 January 2007 (UTC)

"Electrical impedance" and "characteristic impedance" are completely different properties of a component. Saying "impedance" when "characteristic impedance" is meant seems to me to be just plain wrong, and is certainly confusing, unless it has been made clear earlier in the article that the word "impedance" will always mean "characteristic impedance" in this article. I would appreciate it if someone a little less confused than me on this subject would edit the article (and related articles on "output impedance" and "input impedance") to make it clear what quantity is being referred to. Maybe a short section with a definition of "impedance" needs to be added near the beginning of the article. I've fixed two links to say "[[characteristic impedance|impedance]]" rather than "[[electrical impedance|impedance]]". --Coppertwig 12:46, 5 January 2007 (UTC)

To further clarify this discussion: I didn't say the impedance of a transmission line would be zero. I meant that the electrical impedance of a short transmission line is very near zero. Do you agree with this statement? I gather that "impedance" and "Z" in the article do not mean electrical impedance, but characteristic impedance. Also, I gather that in the comment by Light current above, "input and output impedance" do not mean electrical impedance, but characteristic impedance. I have already seen the articles "characteristic impedance" and "transmission line". After seeing these articles, I still thought the word "impedance" in this article meant "electrical impedance", and I incorrectly disambiguated the link to point to "electrical impedance". Someone else very recently made the same mistake on another link in this same article, so it isn't just me. The article "characteristic impedance" does not say "when you see the word "impedance" in an article, it means "characteristic impedance"; it does not mean "electrical impedance." Rather, it gives a definition of "characteristic impedance". This article needs to be edited to clarify what definition of "impedance" is meant. Physics is precise. Every time the word "impedance" is used, it should be clear to the reader what definition is meant. Maybe the word "characteristic" needs to be inserted here and there in the article, to correct it. I may not have enough knowledge to be confident enough to do this myself and would appreciate it if someone else would (or tell me that "electrical impedance" is never meant anywhere in this article, and then I can do it.) --Coppertwig 12:57, 5 January 2007 (UTC)

The article "input impedance" says: "For example, an amplifier with 100,000 ohm input impedance looks equivalent to a 100,000 ohm resistor to the device driving it". A short transmission line certainly does not look equivalent to a 100,000 ohm resistor. Therefore, I gather that "input impedance" does not mean "characteristic impedance". Since the terms "output impedance" and "input impedance" are used in this article, apparently "characteristic impedance" is not always what is meant. Therefore, whenever "characteristic impedance" is meant, it should be referred to as such, to distinguish the two.

You said "you need to see characteristic impedance"; does this mean that the answer to my question is that the quantity referred to by "Z" in the equation above is "characteristic impedance"? --Coppertwig 13:10, 5 January 2007 (UTC)

Well, characteristic impedance is electrical. It's not a matter of measuring a different kind of thing; it's a matter of measuring along a different direction. It's like the difference between the length of a road and its width.

Jim.henderson 18:43, 5 January 2007 (UTC)

(edcon) Usually the only things referred to as having a characteristic impedance are things like cables, filters etc. If you connect a source with Zout=0 to an amp with Zin=100k, via a piece of cable with Zo=50, it will still work ok at low frequencies. But as the frequency gets higher and higher, because the system is not matched, reflections will take place at the discontinuities and you wont get much signal into the amplifier. ::::The quantity referred to by "Z" in the equation above is "characteristic impedance"? That is correct. Basically cables have zero (or very low) series impedance and very high (infinite) shunt impedance at dc, but beyond a few tens of kHz, the shunt impedance falls and the series impedance rises towards its characteristic impedance. 8-)--Light current 18:36, 5 January 2007 (UTC)

Sorry, it's still not clear. You seem to be contradicting yourself. First you say (usually) only cables are referred to as having characteristic impedance; then you say the Z in the equation is characteristic impedance. So, are all three Z 's characteristic impedance? So for this equation, are we doing the rare thing of talking about characteristic impedance for a source and for a load, not only for a cable?

Based on some of this discussion, I changed some of the links in the article from "electrical impedance" to "characteristic impedance". Now I think maybe that was wrong and they need to be changed back. Anyway, for an electrical cable the two quantities electrical impedance and characteristic impedance can be extremely different amounts, so the article should make it clear which is which, every time the word impedance is used. Please help. Saying "impedance" here on the talk page without making clear which it is doesn't help, either.

I would like to add a sentence to the article, immediately after the equation, like this: "where Z_load is the electrical impedance of the load, Z_line is the characteristic impedance of the transmission line, and Z_source is the source impedance of the source, which is the negative of the impedance that would be measured if the source were treated as a load." Would this be OK?

Do some of the links in the article need to be changed back from [[characteristic impedance|impedance]] to [[electrical impedance|impedance]]? —The preceding unsigned comment was added by Coppertwig (talk • contribs) 02:40, 6 January 2007 (UTC).

A resistor equal to the characteristic impedance of the line attached across the load end will match the line perfectly. So a resistor connected across the line can also be said to have a characteristic impedance equal to its resistance. At the source end also, the source characteristic impedance will be equal to its output resitance since this resistance is also effectively across the line. --Light current 03:58, 6 January 2007 (UTC)

If a source and load are connected together directly, for proper matching Zload = Zsource. THese are simple resistances. Now if you want to connect via a cable, you can only do that properly using a transmission line. To get proper matching, the characteristic impedance of the cable must be equal to the load and source impedances. Is that any clearer.--Light current 04:10, 6 January 2007 (UTC)

I edited the article just now, based on the above discussion, and I believe the article is much clearer. I inserted "where Z_line is the characteristic impedance of the transmission line" after the equation. I think this resolves the whole issue. --Coppertwig 13:22, 9 January 2007 (UTC)

(Except that impedance in the text needs to be linked to electrical impedance, not characteristic impedance, I think) --Coppertwig 13:25, 9 January 2007 (UTC)

What's really needed is a diagram, something close to Transmission line, but better. That would clear things up. Transmission lines, amplifiers, filters and the like are four port components, which are a little more complicated than two port components like R, L, and C. Of course, I'm not much of an artist ;-) Madhu 22:15, 12 April 2007 (UTC)

Here you go:

:-)

Which style do you think is best? — Omegatron 02:20, 13 April 2007 (UTC)

Omegatron, your timing is impeccable! I like the third one, it's a classic. Madhu 18:39, 13 April 2007 (UTC)

I'll separate it into its own image for this article and create matching versions of the non-transmission line image. — Omegatron 19:30, 13 April 2007 (UTC)

Here you go:

Source with Zs, transmission line with Z0, and load Zl
Source with Zs and load with Zl
Source with Rs and load with Rl showing current and Vl

— Omegatron 00:43, 14 April 2007 (UTC)

False dichotomy?

The article is still divided up into "Reflectionless or broadband matching" and "Complex conjugate matching", which aren't really logical ways to break it up, are they? This is demonstrated by the sentence "This is used in cases where the source and load are reactive", which is followed by "For the case of purely resistive source and load impedances". How can it only be for reactive components if resistive components are also explained in the same section?

It also implies that transmission lines are only used, and reflectionless matching is only possible, when the sources and loads are resistive, which is certainly not true. — Omegatron 19:30, 13 April 2007 (UTC)

Material from nominal impedance

I am removing the following two paragraphs from nominal impedance as they are discussing problems with impedance matching rather than nominal impedance per se. Possibly, though, they might be useful in this article. SpinningSpark 13:37, 9 October 2009 (UTC)

Unmatched high frequency cables may become resonators, and interactions between resonance signals may produce interference patterns. This is commonly seen when an inappropriate, damaged, or incorrectly connected cable if sufficient length is used to connect a television to a VCR's composite video jack. In lengths of above 20 feet, visible beat patterns will appear on the television if the cable does not have a nominal impedance of 75 ohms (a cheap audio cable for example). Replacing the composite connection with standard RG-6 fitted with appropriate connectors is usually the cheapest and most effective way to eliminate this problem.

Early computer networks used 50ohm cable, which is somewhat thinner and less durable than RG-6 75ohm cable. These networks were plagued with high frequency effects, often leading installers to have to manually "tune" the cable, by adjusting its length in order to cancel out reflections. These problems often occurred due to damaged or irregularly manufactured cable, poorly crimped connections, or excessive length or population of a particular line. These factors all affect the impedance of the line, increasing the likelihood and amplitude of reflections and interference.

improper paragraph

the bold sentence doesn't render a meaning properly; Could someone look into it

Impedance matching is not always desirable. For example, if a source with a low impedance is connected to a load with a high impedance, then the power that can pass through the connection is limited by the higher impedance, but if electrical the voltage transfer is higher and less prone to corruption than if the impedances had been matched. This maximum voltage connection is a common configuration called impedance bridging or voltage bridging, and is widely used in signal pro —Preceding unsigned comment added by 59.96.60.133 (talk) 11:19, 19 January 2008 (UTC)

Yeah I agree. Besides I think the terminology is not well defined in this article. The article discusses 'power transfer' and 'voltage transfer' without properly defining what is meant by 'transfer'. For example, it reads "Bridging connections are used to maximize the voltage transfer, not the power transfer." It would be much more clear to say "Bridging connections are used to maximize the load voltage, not the load power", since power and voltage are well defined physical quantities. However, I think the sentence would not generally make sense, since power (in resistive case) is proportional to the voltage squared, and both quantities would be simultaneously maximized. So, I reckon the 'power transfer' must mean the ratio of the load power to the available source power, similar to mismatch loss as it is often called in radio engineering. What then voltage transfer would mean, I don't know. The article needs some clarifying! Lepokundi (talk) 18:18, 16 February 2008 (UTC)

While I agree that "voltage transfer" is unusual (and confusing) terminology, it is true that low-to-high impedances result in the maximum available voltage. Matched impedances do not maximise voltage, you get half the maximum available voltage in those cases. SpinningSpark 13:43, 9 October 2009 (UTC)

Delete 'Explanation' section

I see nothing worth keeping in that section that isn't somewhere else in this, or another, article. The first paragraph is a needless discussion of impedance, and the second is a special case of the discussion in the third. -Roger (talk) 16:46, 25 May 2010 (UTC)

I agree this is a bit belaboured, but it is worth a quick explanation of impedance, not all readers will be familiar. SpinningSpark 22:45, 28 May 2010 (UTC)

Perhaps a couple sentences, but I think most of it should go. -Roger (talk) 22:50, 28 May 2010 (UTC)

Agreed, as long as that is not taken as me volunteering to actually do it! SpinningSpark 11:30, 29 May 2010 (UTC)

I'll work on it once I feel a bit more motivated :) Just wanted to make sure everyone was in agreement before I invested any time. -Roger (talk) 16:33, 29 May 2010 (UTC)

Description of the EM field in the "Transmission lines" sounds like a standing wave.

"An electromagnetic wave consists of energy being transmitted down the transmission line. This energy is in two forms, an electric field and a magnetic field, which fluctuate constantly, with a continuing exchange between electrical and magnetic energy. ... Assume that voltage and current vary as sine waves. Inside the transmission line, the law of conservation of energy applies: the sum of magnetic and electric energy must always be the same (ignoring the effect of the small amount of energy converted to heat)."

That sounds like a standing wave to me.Constant314 (talk) 21:40, 30 November 2010 (UTC)

Dubious Section: Transmission Lines

This section has several dubiuos facts.

"An electromagnetic wave consists of energy being transmitted down the transmission line. This energy is in two forms, an electric field and a magnetic field, which fluctuate constantly, with a continuing exchange between electrical and magnetic energy" This is the description of a reactive field or standing wave. In a traveling wave, the magnetic and electric fields that simultaneously peak at he same places and simultaneously go to zero at the same places. See Harrington pages 42-48^[1] or Sadiku page 463^[2]

"Now consider two moments: 1). when the current is zero and the voltage is maximum; 2). when the current is maximum and the voltage is zero. The amount of energy stored in the electric field at 1). must be exactly the same as the amount of energy stored in the magnetic field at 2). The ratio between voltage and current at 1). and 2). determines the impedance (Z) of the line: $Z_{0}={\frac {V}{I}}$ " This is not correct. $Z_{0}$ is the ratio of V to I at the same place and time. If there is a phase difference between V and I that makes $Z_{0}$ complex.

"At a boundary, for example, where the line is connected to the receiver, the law of conservation of charge applies. The current just before the boundary must be the same as just after. " Correct, but misleading. If there is a reflection then both voltage and current are reflected. The current just before the boundary is the sum of the incident current and the reflected current. It is that sum which must be equaled by the load current.

"However, if the circuit at the receiver has a different impedance, ZL, than the line, the voltage will be VL = ZLI at the receiver" The voltage at the receiver will be

$V_{L}=V_{0}^{+}({\frac {2Z_{L}}{Z_{L}+Z_{0}}})=V_{0}^{+}(1+\Gamma )$ . See Harrington page 55^[1] or Sadiku page 505^[2]

Note, this reduces to $V_{L}=V_{0}^{+}\,$ if $Z_{L}=Z_{0}\,$ .

"The voltage of this reflected wave, $V_{0}^{-}$ , is calculated from the incident voltage $V_{0}^{+}$ and the reflection coefficient, $\Gamma$ (from the formula above):"

V_{0}^{-}=\Gamma V_{0}^{+}\,

This is correct. Notice that the sum of the incident voltage and the reflected voltage is : $(1+\Gamma )V_{0}^{+}\,$ which happens to also be the load voltage, which it must be because voltage as well as current is continuous across the boundary.

Constant314 (talk) 05:54, 5 December 2010 (UTC)

I agree it's screwed up; esp. the impedance part. I'm not so sure that first sentence is wrong; I'd have to study the diagram of field lines around the conductors, I guess. But certainly the I and V are in phase when the Z0 is real. Go ahead and make it right. Dicklyon (talk) 01:45, 6 December 2010 (UTC)

Notes

^ ^a ^b Harrington (1961, pp. 42–48) Cite error: The named reference "Harrington42-48" was defined multiple times with different content (see the help page).
^ ^a ^b Sadiku (1989, pp. 463) Cite error: The named reference "Sadiku463" was defined multiple times with different content (see the help page).

References

Harrington, Roger F. (1961), Time-Harmonic Electromagnetic Fields, McGraw-Hill
Sadiku, Matthew N. O. (1989), Elements of Electromagnetics (1st ed.), Saunders College Publishing, ISBN 993013846 {{citation}}: Check |isbn= value: length (help)

Resistive network-clarification requested

"Digital circuits" could include logic signals on a circuit board, ethernet, DSL, modems etc. Regarding logic signals, most of the parts with which I am familiar present a mismatch to the circuit. Constant314 (talk) 04:05, 31 December 2010 (UTC)

The bit about digital in the article did appear to be nonsense, so I took it out. There may be some logic gates with built-in impedance matching, but it's not the norm, for sure. There was also a random parenthetical about L pads that someone had stuck after it, so I took that out, too. Dicklyon (talk) 06:31, 31 December 2010 (UTC)

There's a lot of back-and-forth here. The section has been/is confused. There are logic circuits with built-in terminators to make a lossy match to the line; I'm not sure about their prevalence. Integrated IF amplifiers have shunt resistors to swamp process variations and present a controlled impedance; it wrecks the NF but allows controlled Q. "Limit the power" is poorly phrased; resistive matching networks are attenuators; that is the sense that is probably met. The L-pad can be min-loss dual match between resistive impedances. Pi or T can match and choose a different attenuation level. Using resistive matches on low-level circuits is studiously avoided - it degrades the SNR. In low level situations, the appropriate match may be to maximize SNR rather than power transfer. In serious broadband designs (eg, instumentation), attenuators are used to match nominal 50-ohm circuits to 50-ohm cable to minimize the effect of mismatch losses. Glrx (talk) 17:27, 31 December 2010 (UTC)

I think you will find that most of this was addressed in the cleanup edit I made before you posted and you may have not yet seen, but I missed the bit about radio receivers. Clearly it would never be used for an RF front end for the reasons you state. Such circuits are used, however, in "line-level" applications, the 6dB resistive "hybrid" for instance. I was reading low-power in that sense, as opposed to kilowatts. SpinningSpark 22:26, 31 December 2010 (UTC)

Loudspeaker amplifiers

This section confuses a tube's output impedance with the power impedance; parallel/series issue; amplifier mode issue.

In both tube and transistor power (large signal) amplifier designs, the power supply rails and the device current set the impedance. It's not the r₀ of the device.

Glrx (talk) 00:39, 13 February 2011 (UTC)

Some of the factual errors are:

Bipolar transistors have high output impedance at audio frequencies. Low output impedance is the result of feedback. Low output impedance at RF is by feedback through the miller capacitance.
Some semiconductor based amplifiers have transformer coupled outputs.
It is possible to build a dc coupled tube amplifier.Constant314 (talk) 04:31, 13 February 2011 (UTC)

You sure can have a DC coupled tube amplifier, though they are spendy - ever seen a tube 'scope that responded down to DC ? Every pocket transistor radio made for decades had an itsy bitsy output transformer. A transistor is a constant-current device, so high output impedance isn't inconsistent - depends on topology. --Wtshymanski (talk) 14:38, 13 February 2011 (UTC)

Yes, I was assuming common emitter topology. But you could have an emitter follower that would have lowish impedaance. I guess you could also have a similar tube circuit (cathode follower?) that would have lowish impedance. So why do tube amps tend to have transformers: I can speculate

There are no complimentary devices. Tubes come in only one variety. So two tubes driving a center tapped transformer is an easy way to get a push pull circuit.
Tubes tends to develop their maximum power at high voltage and low current compared to transistors. It is convenient to transform the load into a higher impedance.Constant314 (talk) 15:23, 13 February 2011 (UTC)

Valve amplifier is the place to go into details on this, but if you need 100 watts into an 8 ohm load, the final element would have to pass 3.5 amps - with a tube suitable to stand off 300 or 400 volts, that would be a device rated for a couple of kilowatts. So it's not economic to obtain only 1/10th of the rating of the tube without a matching transformer. --Wtshymanski (talk) 16:37, 13 February 2011 (UTC)

Right. So perhaps instead of "impedance macthing" we should call it "impedance scaling".Constant314 (talk) 16:51, 13 February 2011 (UTC)

Good point. But that's not what it's called in reliable sources. Dicklyon (talk) 17:10, 13 February 2011 (UTC)

See section 3 of Becciolini, which states (for power transistors), "The load value is primarily dictated by the required output power and the peak voltage; it is not matched to the output impedance of the device." I believe the same concern is used in tube amplfiers. The example claimed the output Z was 3.5 kilohms, but that number also fits 55 watts and 440 volts.

There is an impedance match being performed, but the impedance match is chosen to keep the device from clipping and provide the max power out.

Becciolini, B. (1993), Impedance Matching Networks Applied to RF Power Transistors (PDF), Application Note, vol. AN721/D, Motorola Semiconductor Products, Inc.

Terman 1943 gives a similar expression for tubes. Class A expression (23) on page 379. Class B express (33) on page 393. Terman refers to the "proper load resistance from plate to plate".

R_{L}=4{E_{b}-E_{min} \over I_{max}}

Where R_L is the plate to plate load resistance, E_b is supply voltage, E_min is minimum plate voltage for one tube, and I_max is the peak plate current.

Terman, Frederick (1943), Radio Engineers' Handbook, McGraw-Hill Book Company, Inc.

Glrx (talk) 21:11, 13 February 2011 (UTC)

So, it is not impedance matching of the load to the output impedance of the amplifier, but rather it is impedance matching of the load to the optimum load of the amplifier.Constant314 (talk) 02:09, 14 February 2011 (UTC)

It is an impedance match to optimize the power efficiency of the amplifier. The typical explanation of impedance matching is to optimize power transfer (get the most power out of the source), but there can be other goals of impedance matching such as maximizing SNR. Sometimes impedances are deliberately mismatched (which reduces gain) to make an amplifier unconditionally stable. Glrx (talk) 02:32, 14 February 2011 (UTC)

Distinction between "electric" and "magnetic" impedance is unphysical

Electric/magnetic impedances are a concept of electromagnetism, i.e. one cannot separate electric and magnetic components. The impedance of a medium is the ration of electric and magnetic fields; the impedance of a conductor is the ratio of applied voltage (E-field) and the flowing current (H-field). — Preceding unsigned comment added by 192.122.131.20 (talk) 09:53, 5 July 2011 (UTC)

Introductory definition of impedance is nonsense

In the first paragraph of the article, impedance is described as opposition to energy transfer. This is nonsense.

Energy in classical waves propagates as a combination of potential-like energy and kinetic-like energy, associated with two fields - pressure and velocity, stress and strain, electric field and magnetic field. Impedance is the ratio of two such components, expressed in the frequency domain. E.g. the electromagnetic impedance of free space is the ratio of electric and magnetic field strengths; acoustic impedance is the ratio of sound pressure and displacement velocity; etc. — Preceding unsigned comment added by 192.122.131.20 (talk) 10:06, 5 July 2011 (UTC)

I think you mean the first sentence of the explanation section. I agree it is a bad definition of impedance. What do you propose to change it to? Constant314 (talk) 15:13, 5 July 2011 (UTC)

unfamiliar w/ format, but must point out...

"In simple cases, such as low-frequency or direct-current power transmission, the reactance is negligible or zero and the impedance can be considered a pure resistance, expressed as a real number" -it should be obvious that at DC(f=0) X(c),(capacitive reactance), approaches infinity & at low freq's, it is very high...X(c)=1/2(~3.14)fc where f=hz & c=capacitance-right?

Tlinafelt (talk) 06:47, 26 August 2011 (UTC)

which section of the article are you referring to?Constant314 (talk) 10:28, 26 August 2011 (UTC)

I tweaked the explanation paragraph to not imply that DC implies the zero-reactance case in general. Dicklyon (talk) 05:58, 27 August 2011 (UTC)

I'm not sure where you are going with this, so let me guess. But first of all, the relevant paragraph lumps low frequency behavior in with DC behavior. I think perhaps there should be a paragraph for each case. But let me continue with my speculation. The general equation for the characteristic impedance, Zc, of a transmission line is:

Zc = sqrt {(R + jωL) / (G + jωC)}

In an ordinary transmission line both R and G are non zero so at DC

Zc = sqrt{ R / G } which is purely resistive.

But, mathematically we can assert that G ≡ 0 and R > 0. In that case the general equation reduces to

Zc = sqrt{ (R + jωL) / (jωC) }

At sufficiently low frequencies R >> ωL which reduces the equation to

Zc = sqrt{ R / (jωC) }

For any frequency above zero, the phase of Zc → 45 degrees as ω → zero. In practice, there are no DC sources; there are only sources with sufficiently low frequency that they can be treated as though they were DC sources. In other words, at frequencies that are arbitrarily close to DC, Zc has a substantial reactive component. Is that the sense that you mean the impedance may not be purely resistive? Constant314 (talk) 19:45, 28 August 2011 (UTC)

In truth, I wasn't even thinking about transmission lines at all, but just lumped circuits. In the case of an infinite transmission line with zero leakage conductance, you have a good point – it's not one of those situations where the reactance becomes negligible at low frequency. On the other hand, if you want to talk about transferring power to a load, through a finite stretch of transmission line, you again get to where there's a low-frequency or DC limit with negligible reactance. On the other hand, in any AC-coupled circuit the reactance dominates at low frequencies. Dicklyon (talk) 23:03, 28 August 2011 (UTC) Note, this comment was copied from User:Dicklyon's talk page with his permission Constant314 (talk) 23:59, 28 August 2011 (UTC)

I had not considered AC-coupled circuits. But, an ordinary capacitor has leakage so it looks resistive if you go to a low enough frequency. But now that I know what your thoughts are, I might have a go at it. I think I would make seperate paragraphs for low frequency and DC. Constant314 (talk) 23:59, 28 August 2011 (UTC)

impedance matching is a myth

The article currently says:

Whenever a source of power, such as an electric signal source, a radio transmitter, or even mechanical sound operates into a load, the greatest power is delivered to the load when the impedance of the load (load impedance) is equal to the internal impedance of the source. This arrangement is called impedance matching.

That is a common myth (although it is very close to being true).

Given a load impedance of 50 Ohms, the greatest power is delivered to that load when the internal impedance of the source is as small as possible (1 Volt at 0.1 Ohm delivers almost twice as much power as 1 Volt at 50 Ohm).

--DavidCary 01:16, 20 Jul 2004 (UTC)

It is not a myth. If the source impedance is unchangeable, then the maximum power you can transfer to the load is when Rload = Rsource. You can't usually change source impedances, unless you are magic. - Omegatron 03:26, Jul 20, 2004 (UTC)

Is so a myth. Naayaa Naayaa :-).

I can put a resistor on the power source, then use the other end of the resistor as my source. (see http://www.reed-electronics.com/ednmag/article/CA56674?pubdate=2%2F18%2F1999 for some good reasons for doing just that). Changing resistors now changes my source impedance.

:-P So you can increase the source impedance, making the power transferred to the load lower? I see that it is useful for transmission lines when the source and load impedances are fixed, but the differences and similarities between impedance matching for reflections and maximum power theorem needs to be explained better. - Omegatron 22:39, Aug 14, 2004 (UTC)

You are quite correct for the case where I can change the load impedance, but not the source impedance or source voltage. The "myth" comes when people incorrectly apply the statement in the article to other cases.

Is there some way to change the statement in the article so that it is always true ? Or to emphasize which situations it is true, and which it is not true ?

--DavidCary 21:36, 14 Aug 2004 (UTC)

I added a comment that the maximum power theorem applies only when the source impedance is fixed. There might be some other subtleties that I have overlooked, so please correct me if I'm wrong. -- Heron 22:10, 14 Aug 2004 (UTC)

There seems to be no argument that for a given power source, with a given source impedance the maximum power into the load is maximum when the source and load impedances are equal: that has been well proved mathematically. In many cases the 'power source' impedance cannot be changed, ie radiation resistance of space, impedance of a transmission line etc, so you just have to match to it. Of course, good matching, is also important to minimise reflections and standing waves for hf signals. However, in many cases efficiency is much more important than matching, as in the domestic mains supply or a automobile battery for example. If power transfer is viewed from the efficiency point of view, it is then true that the lower the internal resistance of the power souce the more efficient is the power transfer to the load. I suspect that this is where the debate arises. Ideally, the source resistance in this case should be zero, so efficiency is maximum (with any load). In fact, for many power sources, certainly those mentioned, the load varies greatly with time and they do have an internal resistance approximating to zero (try dropping a spanner/wrench across your auto batt). Most electronic PSUs also have a near zero output impedance even at relatively high frequencies. Audio power amps are often designed to have an output impedance near zero for maximum efficiency and to aid loudspeaker damping (another debateable topic). Perhaps a change to the 'Impedance Matching' page would clarify the point about efficiency and matching, which to me anyway, is not clearly explained at the moment? - CPES 01:01, 10 Feb 2005 (UTC)

By the way, low source impedance is addressed in the article impedance bridging. I named it that, and there is probably a better name, like voltage bridging. Google returns few for either term. - Omegatron 01:38, Feb 10, 2005 (UTC)

"Given a load impedance of 50 Ohms, the greatest power is delivered to that load when the internal impedance of the source is as small as possible (1 Volt at 0.1 Ohm delivers almost twice as much power as 1 Volt at 50 Ohm)."

This is not true. First of all, talking about different loads in Ohm it should be called resistance, not impedance. Impedance is a complex quantity. (http://en.wikipedia.org/wiki/Electrical_impedance) If you calculate however with simply resistances you are mixing up voltage with power by calculating twice as much power. If a source voltage (Vs) is 1V, source resistance (Rs) is 0.1Ohm and load resistance (Rl) is 50Ohm then the voltage drop on Rl is 0.998V. Using a 50Ohm Rs it gives a 0.5V voltage drop. So the voltage drop on the load by 0.1Ohm Rs is almost twice. Power however is calculated by the following equation: P=I*V (where I=V/R) wich means the power delivered on Rl by 0.1Ohm Rs is 0.02W and by 50Ohm ~0.005W wich means the power by 0.1Ohm RS is about 4 times bigger than with 50 Ohm. Talking about impedances however, as Omegatron mentioned the maximum power into the load is reached when the source and load impedances are equal with other words the line is terminated with a wave impedance. (http://en.wikipedia.org/wiki/Wave_impedance) [Peter] —Preceding unsigned comment added by 84.0.211.6 (talk) 10:02, 24 May 2009 (UTC)

In some systems, the load impedance can be easily adjusted, in which case maximum power will be transferred when the load is set to the complex conjugate of the source impedance. In other systems, the load impedance is fixed, e.g. an 8 ohm speaker, and the source impedance is adjustable. In this case, maximum power is transferred when the voltage across the load is maximized, which is when the source impedance is minimized (assuming low frequencies so no transmission line effects). Despite appearances, this does not violate the maximum power theorem because a reduced source impedance could transfer even more power if the load impedance were then reduced {or the smoke appears). In mathematical terms, the maximum power theorem assumes the source impedance is fixed. A different result appears if the load impedance is held fixed, namely that maximum power transfer occurs at a minimum source impedance (or perhaps at an impractical negative source impedance}.Overjive (talk) 10:22, 28 March 2010 (UTC)

This ain't rocket science, folks. (Sorry if I sound condescending--I'm actually disturbingly, uh, fallible.) It just seems like some people here still don't quite understand what's going on here.

First off, it is basically true (without going into the nitty gritty of it--it's not applicable in this context) that you get maximum power transfer with matched impedances. But, in audio, you generally don't want maximum power transfer! This would waste a lot more power as heat than we already have to, and "power" isn't the point anyway. Voltage is. Power concerns us mainly because, in the real world, speaker voice coils have finite impedance, dominated by DC resistance (e.g., about 6-7 ohms). And since Ohm's Law (the general case involving Z, not just R) is Ohm's LAW, not "Ohm's Rule of Thumb" or "Ohm's Thing That Usually Happens Like The Equation Says, But Common Sense Says This Case Can't Really Be True, Can It?," when we apply voltage across a finite impedance, a non-zero current flows, resulting in a non-zero product of E and I. Hence, it takes a lot more current, therefore power, to drive a speaker than to drive, say, the high-Z input of an amplifier. If there is insufficient power available, the voltage applied to the speaker will sag, and southward goes the SPL as well.

But the loudness of an audio signal, at the end of the day, isn't proportional to the power that goes into producing it, but the voltage of the signal. (Yes, these are usually, but not always, proportional to each other.) Think of the current as a necessary side-effect of applying signal voltage across a speaker (or anything else), and the same of the resulting power dissipated. The voltage is the thing. Ob1-kenogle (talk) 07:07, 4 March 2013 (UTC)

So you think that a 4 Ω speaker and a 16 Ω speaker connected in parallel to an amplifier will both produce the same SPL? They must both have the same voltage across them after all. I invite you to try that to satisfy yourself that you are wrong. Spinning Spark 07:41, 4 March 2013 (UTC)

(Sigh) Yes, indeed they do, but no, of course I don't think that--nor did I say I did, although upon reading my words again I see too much potential for misunderstanding, and I should therefore have been clearer. In particular, the sentence I so unwisely set in boldface above makes it much too easy to think I meant something else that I didn't mean and isn't true. I said that the SPL generated by, or loudness of, a speaker is proportional to the voltage across the coil, but that's not saying that any two speakers will be equally loud when equal signal voltage, or power, or anything else, is applied to them, but then we're not attempting--I hope--to account for all the factors that ultimately determine loudness, e.g. the speaker's efficiency, the strength of the magnet, the number of turns in the voice coil, the material the coil and cone are made of, what it had for lunch that day, etc., and so forth ad tedium. The amplitude of the movement of the speaker cone, plotted against time, will be proportional to the amplitude of the signal voltage across it plotted against time. (Please don't say that I think it will be exactly so, though.) Trouble is, this E/I/P stuff can get confusing because of the very Law that helps us understand it, because whenever one increases the voltage across a load, one necessarily also increases the current through it, and the power dissipated by it, IFF the load is invariant. The whole "correlation vs. causation" thing often cornfoozles people about what may be a cause, a consequence, or only a correlation. One could well interpret the data to mean that current or power is the determining factor, and now that we're beating this poor horse to death, I do think that it really doesn't matter enough to make an issue of it, at least not in this setting. In spite of having brought it up myself. I think.

I surely hope this isn't becoming the focus of more attention than it's worth. Hey, we could start a new thread! Or...not. Ob1-kenogle (talk) 15:46, 4 March 2013 (UTC)

Reflection-less matching should avoid "should"

I’m generally of the opinion that encyclopedic articles should stick to describing what is and has been and should avoid saying what should be. In particular, the assertion that the characteristic impedance of transmission lines should be resistive is nonsense. There are billions of twisted pair transmission lines connecting telephones to telephone offices. In the voice band the characteristic impedance has a substantial reactive component and it is exactly what it should be. Furthermore, impedance includes a pure resistance and there is no need to justify the use of impedance to refer to a purely real resistance. Accordingly I am removing the comments saying that the impedance should be resistive and the comments that it is conventional to use characteristic impedance even when the impedance is purely real. On the other hand it would be acceptable to post reasons while a pure resistance may be desirable in certain circumstances.Constant314 (talk) 00:27, 15 December 2013 (UTC)

I agree with you about "should" but I think you have been overly savage in your trimming of the passage. That a purely resistive characteristic impedance is the ideal is good information. That the ideal case is often assumed in calculation could also be mentioned. Spinning Spark 01:28, 15 December 2013 (UTC)

Yes, I had started out to cut one or two sentences but as I looked at what remained, it also seemed wrong and I cut some more. Here is a sentence by sentence look at what was cut and why:

1. Ideally, the source and load impedances should be purely resistive: in this special case reflection-less matching is the same as maximum power transfer matching. [Retained and modified to eliminate ideally.]

2. A transmission line connecting the source and load together must also be the same impedance: Zload = Zline = Zsource, where Zline is the characteristic impedance of the transmission line. [This is wrong. There are lots of mismatched transmission lines in operation.]

3. The transmission line characteristic impedance should also ideally be purely resistive. [This is wrong in the case of telephone cable where ideal is meeting the primary line parameters as called out by the customer which lead to a substantially reactive component in the characteristic impedance. In fact, the characteristic impedance of most transmission lines is complex for voice band frequencies.]

4. Cable makers try to get as close to this ideal as possible and transmission lines are often assumed to have a purely real characteristic impedance in calculations. [The first clause is definitely wrong for voice band telephone cable. The second clause could be salvaged but would need some qualifying explanation. Is this assumption applied even when it is wrong? Is it true because when the calculations are made the characteristic impedance is almost purely real?]

5.However, it is conventional to still use the term characteristic impedance rather than characteristic resistance. [Unneeded because impedance includes resistance. Wrong because often the characteristic impedance is complex.]Constant314 (talk) 18:26, 15 December 2013 (UTC)

I don't really want to get into a line by line discussion on this. I am happy to see it go, but as I said in my previous post which you deleted for some reason, there are a couple of points that are worth mentioning and you have not really replied to them.

I don't want to argue with you line by line, but I will take up one point. You have made a great deal of telecom analogue twisted pair having a large reactive part to characteristic impedance. This is very true, but that does not mean that this is ideal from the telecom engineers point of view. Trying to correct this is the whole point of line loading which in the era of analogue was pretty ubiquitous. It is also true that telecom designs frequently treated the line characteristic impedance as resistive and terminated it with 600 ohm anyway regardlesss of knowing that there was a reactive component. This situation explains the popularity of Zobel equalizer sections in telecoms: they guaranteee that the equalizer design will precisely equalize the line to the response measured with a 600 ohm test set despite the line not actually being 600 ohms. Spinning Spark 22:53, 15 December 2013 (UTC)

If I deleted your post, it was entirely by accident and I apologize.Constant314 (talk) 05:50, 16 December 2013 (UTC)

The clause I deleted “often assumed to have a purely real characteristic impedance in calculations” almost definitely does not apply to the majority of the subscriber lines. It’s true that if you terminate a subscriber line with 600 ohms, then it looks like 600 ohms at the other end and you can assume 600 ohms in your calculations, but that is not using the characteristic impedance in a calculation. Most people do not even know the value of the characteristic impedance in the voice band (about 1000 + j1000 at 400 Hz for plain 26 AWG air core for example) and fewer actually use it. They are just using the impedance of the terminated line.Constant314 (talk) 01:25, 17 December 2013 (UTC)

Make that 1000 -J1000. Constant314 (talk) 18:58, 17 December 2013 (UTC)

Impedance Matching Devices Sentence Needs A Verb

Under "Impedance Matching Devices," the following sentence needs a verb or some kind of rework: "Devices intended to present an apparent source resistance as close to zero as possible, or presenting an apparent source voltage as high as possible." Greg J7 (talk) 16:12, 17 June 2014 (UTC)

Conjugate Matching and Reflectionless Matching

The term 'reflectionless matching' I have not heard before but I think both terms describe the same desireable outcome. That is, when a source and load are matched, no energy whatsoever is reflected. In order to do this with a simple resistive source it only requires that the source and load resistances are made equal.

In the case of complex sources and loads however, it is neccessary to employ the technique of complex conjugate matching to ensure max power transfer. I believe its done all the time to match the output of transmitters to their antennas. If the source reactance is inductive, then the load reactance must be capacitive. etc. This will result again in max power transfer. Whether this power is just as great as if you had purely resistive source and load, I'm not sure without referring to my library articles. This can be seen if you go thro the math. I not going to do that here!! Light current 16:35, 10 August 2005 (UTC)

If you don't understand the difference between them, maybe I shouldn't have directed you here. The "Reflectionless matching or broadband matching" and "Complex conjugate matching" sections describe the difference pretty well.

In one, you are matching impedances exactly to prevent reflections down a transmission line. You set the line and load to the same impedance and there will be no reflections at the line-load boundary. You set the source and line to the same impedance so that if there are reflections from the load, they won't reflect back again when they get to the source. If you have a capacitive impedance at the load, you have a capacitive impedance in the source and line, too.
The maximum power theorem is entirely different. You match the complex conjugates of the impedances of a source and load so that, if the source has a fixed impedance, you get maximum power dissipation in the load. If your source has a capacitive impedance, you have to "balance it out" with an inductive impedance at the load. I believe this is the same thing as power factor correction, but I could be wrong.

The only thing that this article needs is to be cleaned up and separated into two good articles.

In the case of purely resistive impedances, the two ideas sound like the same thing, which causes a lot of confusion. Please be careful. We're trying to make it less confusing; not mix them up more. - Omegatron 18:28, August 10, 2005 (UTC)

No Im sorry I disagree, I have rewritten the firt half of the article. Please have a look. I dont think it needs separating yet until we see what we have got in total. I am in the process of sorting it. Is it OK by you if I carry on? :-)Light current 19:08, 10 August 2005 (UTC)

It is true that for maximum power transfer from the source to the load, the load impedance should be the conjugate of the source impedance. Now, insert a transmission line between the source and load. What should the load impedance be for maximum power transfer? The answer is that the load impedance should be such that the impedance seen looking into the line from the source is the conjugate of the source impedance. This guarantees that maximum power is delivered to the line and, if the line is lossless, on to the load. Thus, if the load is matched to the line, maximum power transfer from source to load only occurs if the source is also matched to the line. In the general case where the source is not matched to the line, maximum power transfer does not occur from the source to the load even though there is a 'reflectionless' match at the load end of the transmission line. Thus, it seems reasonable that the ideas of matching for zero reflection coefficient (no standing waves) and matching for zero total reactive component (maximum power transfer) are worthy of separate discussions. Alfred Centauri 19:10, 21 August 2005 (UTC)

It is incorrect to say that reflection-less matching is the condition where ZL = ZS. I believe that reflection-less matching is a special case of conjugate matching. I feel the whole theory part of this entry could be better written and here is my suggested wording starting from the beginning. (I have left out any formatting). "For some given complex source impedance Zs, maximum power transfer to a load impedance ZL is attained when ZL=Zs* (1). For a general load impedance ZL, impedance matching is the practice of providing a matching network before the load such that the impedance looking into the matching network and load is Zs*. In many microwave systems the load is connected to the source via a transmission line (TL). For maximum power transfer, (1) is still the necessary condition. (1) is now interpreted such that it applies at any plane between source and load: ZL and Zs are the is impedances looking towards load and source respectively. If (1) is true at any one plane it is true at any plane between source and load. Even under the condition of maximum power transfer (and conjugate matching),in general there is a reflection back from the load towards the source. The presence of forward and reflected waves on the TL results in increased line loss and increased maximum voltage and current on the line, all of which are undesirable. Reflection-less matching eliminates this reflection by providing two matching networks, one at either end of the TL. One matching network matches the source to the characteristic impedance Z0 of the TL and the other matching network matches the load to Z0. All matching is conjugate matching." Jkeevil (talk) 19:17, 23 May 2014 (UTC)

Are you assuming that the characteristic impedance of the transmission line is purely real such as 50 ohms? In that case, at the load end, conjugate matching and reflectionless matching are the same.Constant314 (talk) 22:28, 23 May 2014 (UTC)

I only consider the case when the TL Z0 is real. Complex Z0 is an unnecessary complication. It is also a very unusual case which need not be considered at this level. When the Z0 of the TL is real, conjugate matching and reflection-less matching are not the same. As I state above, reflection-less matching is a special case that uses conjugate matching at both ends of the TL so that there is no reflection on the line. Jkeevil (talk) 14:42, 24 May 2014 (UTC)

The telephone subscriber line is a transmission line with a characteristic impedance that has a substantial imaginary part. There are billions of these. The ordinary RG58 coaxial cable has a characteristic impedance that has a substantial imaginary part at audio. It is still about 10% reactive at 200 kHz. You can try to match it with a resister all day long and you still have a substantial reflection because you didn’t match the imaginary part. So yes, it is important. There is no reason for the article to be incorrect for these cases.

But I think that I am coming to understand what you are saying. Let’s start with a system that has a transmitter, a matching network, a transmission line, a matching network and then a load and consider narrow band situation. Let’s say the impedance of the TL is 50 ohms, the load is 100 -J10 and the source is 10 - J5. So at the load we put +J10 ohms (an inductor) in series with the load and then an ideal matching transformer. So the input to the matching transformer, as seen by the transmission line is 50 ohms. The load sees 100 +J10 so it sees its conjugate impedance. At the source end we put +J5 in series with the source and then an ideal matching transformer. So again the TL sees 50 ohms and the source sees it conjugate impedance. It all goes out the window though if the TL has a characteristic impedance that is not purely real. In that case, if you match for no reflection, the load and source do not see their own conjugate impedance.

The article is about matching whether you match both ends or not. We cannot tell the reader that they aren’t doing matching unless they match at both ends. Still, I think it is an interesting special case and if you want to add it as a special case I would not object.Constant314 (talk) 01:20, 25 May 2014 (UTC)

Consider ZS, ZL and TL Z0 are all complex then. I still don't find ZS=ZL a matched condition. My derivation for the matched case shows zero reflection and maximum power transfer are achieved when ZL=Z0=ZS* for arbitrary lossless TL length. I suggest you try both cases in a reliable circuit simulator and check for yourself. I can email you my derivation if you care to look at it. (It is also satisfying for me to see that if the TL length were reduced to zero and therefore removed from the system that the criterion I found is still ZL=ZS* which of course is the usual maximum power transfer criterion. Jkeevil (talk) 14:55, 25 May 2014 (UTC). Withdrawn.Jkeevil (talk) 14:55, 25 May 2014 (UTC)

That is precisely the arrangement shown in figure 6.30b of the source I linked below. It is still the case that we don't need to consider the matching at both ends simultaneously in the article, but now that we know it is used by practioners of the art, I agree with Constant314 that it could be used as an example of the application of matching. Note that waves returning down the TL in the reverse direction will see a mismatch at the source and will be reflected back towards the load. Spinning Spark 16:09, 25 May 2014 (UTC)

An semi-infinite length of transmission line is reflectionless. The impedance looking into that TL is Z0, the characteristic impedance of the TL. A finite length of TL terminated by an infinite length of the same type TL is also reflectionless. The impedance looking into it is also Z0. But the finite length is terminated by an infinite TL that looks like Z0 so the infinite length can be replaced with an impedance Z0 so you are left with a finite length of LT terminated by Z0 that is reflectionless.Constant314 (talk) 03:25, 26 May 2014 (UTC)

@Jkeevil:. I have restored the post you deleted. Please don't do this after others have replied to it—strike it out instead. Spinning Spark 14:56, 26 May 2014 (UTC)

Consider an infinite line with some complex characteristic impedance Z0. At a plane through some point in the line the impedance looking in either direction is Z0. Thus the join in the line at that point has Z0 facing Z0, not Z0 facing Z0*. Do you suppose that there is a reflection on the line at that point? If so, there will be a reflection at every point on the line. Spinning Spark 23:07, 23 May 2014 (UTC)

I am not familiar with what happens when TL Z0 is complex. I think a better 'thought experiment' is to consider what happens when the TL between the source and load tends to zero length. If ZL=ZS* then maximum power transfer occurs. If (as the entry on Impedance Matching now states) ZL=ZS for reflection-less matching, then max power transfer does not occur, so ZL=ZS for reflection-less matching cannot be correct.Jkeevil (talk) 14:42, 24 May 2014 (UTC)

Termination for max power transfer and termination for reflection-less matching do not mean the same thing.Constant314 (talk) 23:25, 24 May 2014 (UTC)

The concept of "reflection" has no meaning unless a transmission line (or some other object that is distributed through space) is considered. A reflected wave is a wave that travels somewhere. It is meaningless to talk of a reflection at a lumped load connected to a lumped source. By the way, I have corrected your indentation—this is a long and complicated thread so please either indent correctly or just post to the end of the thread to avoid confusion. Spinning Spark 15:59, 24 May 2014 (UTC)

Numerous sources define the reflection coefficient as (ZL-Zs)/(ZL+Zs). This is clearly zero when ZL = Zs and if Zs is complex then the reflection coefficient when ZL=Zs* is not zero.Constant314 (talk) 23:48, 23 May 2014 (UTC)

There is a reflection on the TL even when the system is matched: unless you use reflection-less matching.Jkeevil (talk) 14:42, 24 May 2014 (UTC)

But there is fix up needed on matching for maximum power transfer. ZL=Zs* only applies when Zs is fixed.Constant314 (talk) 23:59, 23 May 2014 (UTC)

I was beginning to doubt the correctness of the article because I can't get my head around more power being delivered when there is a reflection (I think it is something to do with the power wave having an imaginary part and energy stored on the line but I'm not sure). However, I found this source which pretty much explains the difference of the two matching schemes exactly as in our article. The book is on antenna design and if anyone knows the right answer on reflections it is antenna designers because if they get it wrong their shiny new expensive transmitter goes up in smoke. I think that is pretty much a slam dunk as far as this discussion goes unless someone can come up with an even more authoritative source. Spinning Spark 09:36, 25 May 2014 (UTC)

no authoritative source on my side but just a gut feeling about why more power is being delivered when there is a reflection: aren't the active components introduced by the matching network working in this direction? They are part of a resonant circuit whose frequency is the same as that of the source. — Preceding unsigned comment added by 95.237.211.244 (talk) 16:04, 7 August 2014 (UTC)

2 authoritative sources: link1 and link2

...when the load is equal to the complex conjugate of the source impedance, the reflection coefficient is zero.

It seems "Conjugate matching is not the same as reflectionless matching" as this new link states link3

"Reflectionless matching" refers to matching the load to the line impedance, ZL = Z0, in order to prevent reflections from the load => There are no reflected waves and the source (which is typically designed to operate into Z0) transmits maximum power to the load, as compared to the case when ZS = Z0 but ZL ≠ Z0.

"Conjugate matching" allows absolute maximum power transfer.

So, I deduct they cannot be applied together unless Z0 is real (lossless line).

Some sources are a bit misleading in this respect: link4

--95.237.211.244 (talk) 17:54, 7 August 2014 (UTC)

Clarifications to the L-match section

While I hesitate to get detailed about the information in the section, I found several statements which are dubious. I believe this info was brought over correctly from the cited reference so it is hard to know who is to blame, presumably the reference. The article states that the L-match is a narrow band solution. In the portion I just added, I compute the circuit Q and find it to be quite small, usually < 3. The bandwidth is approx. 1/3 or more of the operating frequency, which is reasonably wide. And there was a statement mentioning inductor Q as the limiting factor in the use of this circuit but the inductor Q is normally much higher than the circuit Q so it's losses are very small if the circuit is properly built. Therefore, I am unable to verify these statements.

Citations are a sore point with me, especially in fields which are mathematical. When the reference says one thing and the math says another, who am I to believe? And what about "reference unknown," i.e., something I was taught in school or work, a technique that works, and can be shown to be valid mathematically, but the original source is obscure? Isn't the point here to be accurate in the information? Citations are a false god, a form of idolatry. I am all for giving credit to the person who invented/discovered something useful but giving credit doesn't make something useful or accurate.

RDXelectric (talk) 23:36, 27 October 2016 (UTC)RDXelectric (2016/10/27)

The section has problems. The symbols in the figure do not match the symbols in the text. I will add a figure with the same symbols as the text. I'll leave it up to others to decide whether to remove the old figure.Constant314 (talk) 17:52, 28 October 2016 (UTC)

The recently added equations using Q are completely equivalent to the preceding equations and are visually simpler so I subsumed them into the preceding equations. Though it is typical that X₁ and X₂ are treated as unsigned values, in the references, with the understanding that one of them is a capacitor, the one of them that is a capacitor actually has a negative reactance. To be more mathematically rigorous, I put X₁ and X₂ inside absolute value signs. I would prefer to treat X₁ and X₂ as signed values, but that would be a greater departure from the references. Don't like it? Fine. Take away the absolute value signs and add an explanation that X₁ and X₂ are actually the absolute values of the reactance. Or use the fully signed treatment. I'll be happy to make that change if it is agreeable. Constant314 (talk) 19:34, 28 October 2016 (UTC)

Added reference. Hayward assumes R₁ < R₂ so the subscripts have to swapped around.Constant314 (talk) 21:21, 28 October 2016 (UTC)

This is better but I suggest the last statement, X_1 approx R_1/R_2 is superfluous, the only time that is true is when the Q is high and that's bad, it is always preferable to keep the Q low for efficiency, even if that means using more than one L-section. That maintains the broadband nature too. — Preceding unsigned comment added by RDXelectric (talk • contribs) 15:20, 29 October 2016 (UTC)

"The L-section is inherently a narrowband matching network with a greater impedance ratio resulting in a larger Q." I must take issue with this line at the end of the first paragraph, it simply is false. We used these networks when I worked at Collins Radio to match the output impedance of a high power transistor amplifier to the antenna (50 ohms) for military UHF radios that covered the 225-400 MHz band which is not narrow. RDXelectric (talk) 15:27, 29 October 2016 (UTC) RDXelectric

I know what you mean. It's only exact at one frequency, but its good enough over a wider band, especially if the impedance ratio is close to one. I have no problem with removing narrow band but there should probably be some statement that it is not broadband like a transformer that might cover three or four decades. Constant314 (talk) 17:20, 29 October 2016 (UTC)

Your example is just a bit less than an octave so requires a fractional bandwidth of 2/3, or a Q < 1.5. Plugging that in to the expression in the article (I'm assuming it's right) gets R1/R2 < 3.25. Should be achievable with a common base amplifier (but you'd be stuffed with common emitter). Filter design becomes difficult at more than an octave bandwidth, and impedance matching is an exercise in filter design, so that example is probably close to the practical limit. Here's a cite: Multi-octave impedance matching is almost exclusively done with transformers, although bandwidths up to 1-2 octaves may be possible with complex LC networks in conjunction with negative feedback etc. SpinningSpark 18:30, 29 October 2016 (UTC)

L-section diagram issues

The description for the first diagram in this section discusses the use of complex impedances - inductors/capacitors, but the American resistor symbol is used in these instances. I propose that these should be changed for boxes, as in the diagram below it. Benjamin J. Crawford (talk) 21:56, 6 November 2019 (UTC)

[Harrington42-48-1] Harrington (1961, pp. 42–48) Cite error: The named reference "Harrington42-48" was defined multiple times with different content (see the help page).

[Sadiku463-2] Sadiku (1989, pp. 463) Cite error: The named reference "Sadiku463" was defined multiple times with different content (see the help page).

[1]

[2]