Talk:Checker shadow illusion

Wrong picture

This article definitely needs a different picture, in the one showing at the moment square A is obviously darker than square B. —Preceding unsigned comment added by 129.240.146.29 (talk) 13:28, 7 December 2009 (UTC)

Sigh. -- 98.108.219.226 (talk) 05:49, 24 February 2010 (UTC)

Yes, it is in fact darker if the image is interpreted as a 3D projection. But the optical illusion is that it still looks darker on the 2D plane of the projection (i.e. pixels of the image file), which we cannot truly conceive as a whole, because we cannot entirely disable our spatial perception which automatically makes the shadowed area of lighter color because it does correct for 3D lighting. I added the projection logic to the article's explanation of the optical illusion.37.201.227.22 (talk) 07:52, 17 July 2014 (UTC)

Who removed the useful illusion deconstructed?

https://en.wikipedia.org/wiki/File:Optical_illusion_greysquares.gif

Sean McHugh (talk) 08:19, 5 March 2014 (UTC)

In my opinion too this animation is the only convincing demonstration that these two squares are really the same color. Without this animation, I couldn't convince myself they are. Nyh (talk) 09:08, 21 January 2020 (UTC)

Bad Joke?

Maybe I didn't get the whole thing, but are those squares really supposed to have the same color? Because any 'pick color' tool will tell you this is nothing but a joke: there's a gradient from #b9b9b9 to #6c6c6c between those 2 squares.

Are YOU joking? It's #787878 all the way. What image are you looking at exactly? This one [1] from A to B? It's #78. Try moving your hands on either side of the "gradient", and you'll see it. Herd of Swine 03:23, 14 June 2007 (UTC)

Sorry. I was using a modified pick color tool with average sample over a 20px radius. It obviously didnt show the right pixel values. Shall I delete what I said?

No, others might make the same mistake. It really does LOOK like there is a gradient, and it's hard to believe it's not. Herd of Swine 22:15, 15 June 2007 (UTC)

At the size in the article, it looks like there's a gradient, but this becomes less apparent the further in you zoom. When the bridged A-B shape is about 15mm tall, it almost appears to be the solid grey it is. I zoomed the full-size image to 400% on a 1280*1024 17" 4:3 monitor. boffy_b (talk) 11:19, 15 March 2008 (UTC)

I put it to you that when you think you are sampling square B and getting the #787878 reading you are in fact sampling the shadow cast over the square, not the square itself - or at least a combination of the two. The shadow is a distinct entity, not to be confused with the square. It comes and goes according to the light source and that green thing. The real illusion here is in seeing your colour picker as perfectly objective.
Andrew van der Merwe, calligrapher, --Beachscriber (talk) 19:36, 6 October 2009 (UTC)

It helps if you just put your fingers on boths sides of the "bridge" if you look on the proof picture, then it's immediately obvious. It's a pretty cool illusion. :) Altairantares (talk) 02:24, 10 November 2009 (UTC)

There is no "square itself", there are merely pixels -- and people misperceive the relationships between the colors of those pixels. And that (properly working) color pickers are perfectly objective about the color of pixels is no illusion. -- 98.108.219.226 (talk) 08:16, 24 February 2010 (UTC)

I just tried the fingers on/near the bridge thing... didn't work for me. I still saw two distinct shades of grey with a greyscale between. I haven't tried blowing up to 400% yet, but I don't see how that will change my perception of what is and is not there... —Preceding unsigned comment added by 74.62.116.104 (talk) 06:10, 11 September 2010 (UTC)

Move?

I think this page should be titled Same color illusion. NickelShoe 14:57, 16 December 2005 (UTC)

• I agree with you. --StevenL 04:00, 5 January 2006 (UTC)
  • Guys, everybody else in the world calls this the checker shadow illusion. There are many, many other brightness illusions that also result in two patches appearing to be different colors, when in fact they are the same; thus, the name "Same color illusion" is hardly descriptive. 128.197.81.69 15:00, 28 March 2007 (UTC)
    • Can I point out that the actual letter B is darker than the letter A, lending to the illusion of the patch difference?
      • You're wrong and it's off topic. -- 98.108.219.226 (talk) 05:53, 24 February 2010 (UTC)
    • So why was this incorrectly moved? -- 98.108.219.226 (talk) 05:53, 24 February 2010 (UTC)

Yay!

If I squint, and cover the green cylinder with my hand, I can see the identity! Try it! DS 14:55, 21 January 2006 (UTC)

You are a liar, sir. They are not the same color. This might work in a real-life example, but the picture is a mock-up to show the effect. —Preceding unsigned comment added by 138.163.0.43 (talk) 18:46, 15 January 2008 (UTC)

It works to me. I don't have to squint deliberately as I have a slight squint anyway, but if I put my finger between squares A and B they appear the same colour to me. Wouldnt that be the best way to invite people to test it?130.88.123.189 (talk) 12:04, 20 April 2009 (UTC)

Sigh. It's not a mock-up, they are the same color. -- 98.108.219.226 (talk) 05:54, 24 February 2010 (UTC)

Missing Links

There used to be links to Flash files and other animations that show the truth of the same-colorness much better than the animated gif does. Where did those links go??? 70.20.147.17 12:33, 7 August 2006 (UTC)

Physics or Psychology?

It seems like most other optical illusions stubs are classified as psychology stubs. Is there any particular reason why this one is called an optics stub instead? Stebbins 06:59, 8 November 2006 (UTC)

Visible simmilarity

Wouldn't joining squares A and B with line of the same color (in the green cylinder image) make the simmilarity more obvious ? I mean something like this---- Xil/talk 17:01, 7 May 2007 (UTC)

Yes, i agree, your image does make the similarity more obvious Rombust 09:20, 14 June 2007 (UTC)

Not entirely - To me it looks like the current bridge of the "same color" is actually a gradient of shades, lighter on one end than on the other. (Certainly I believe you that the bridge is just one color - There just isn't that "aha!" moment where I can see for myself it is.) -- 128.104.112.147 (talk) 14:36, 2 June 2008 (UTC)

(Should have clicked on Xil's link instead of assuming it was the same as the current image ([2])) To me, the wider band of Xil's image does a better job than the narrow one currently used. -- 128.104.112.147 (talk) 14:41, 2 June 2008 (UTC)

Best way to do is to use paint take the sample color tool and a make solid square with it. When u move the square between the 2 the shade changes. It wired i get he same effect by putting that same color square on one and a 2nd one on the opposite side and they will appear different.Kirbyroth (talk) 18:43, 5 November 2008 (UTC)

Removal of the Animated Gif

I feel that the animated gif on this article is not helpful at all. For one thing it animates way too fast, and it changes so much that it doesn't prove the illusion in my own personal opinion. Oh and what's that circle thing that comes into view on the far right on a few frames!?

Conflating Squares vs. Representations of Squares

When the article says the squares are the same color, it is making the same mistake it is trying to educate readers about.

It is only the physical parallelogram-shaped regions in the image that are the same color. There are no actual squares in the image.

The squares are in the perceived scene. Since the image has shading representing a cylinder and its shadow, you can't say that the squares are not the same color. —Preceding unsigned comment added by 216.1.16.126 (talk) 23:18, 3 February 2009 (UTC)

That's pointlessly pedantic -- and incorrect. This article is not about 2D representations of 3D objects. -- 98.108.219.226 (talk) 05:58, 24 February 2010 (UTC)

Explanation?

It seems to me that this article is missing something rather important. Does anyone know if this illusion has been explained and, if so, what the explanation is? This is what I came here to find. —Preceding unsigned comment added by 86.142.90.162 (talk) 12:19, 4 October 2009 (UTC)

I just noticed the "Explanation of the effect" reference but it still seems that some of the information from the reference should be added to the article. —Preceding unsigned comment added by 86.142.90.162 (talk) 12:23, 4 October 2009 (UTC)

Equivocation

This is not an optical illusion but an error of logic - an equivocation to be precise. The human eye makes no mistake here whatsoever. The squares are not the same colour and they are not "mistaken" to be different. It's all quite silly really. Obviously, any object, whatever its colour, is affected by its lighting conditions. The lighting conditions do not change its colour any more than an absence of light would make it black! We see and remember objects under different lighting conditions and are thus able to judge their colour more objectively. By objectively, I mean that we are usually able to judge, with reasonable accuracy, their colour, regardless of how they are lit at any particular time.

Observe that, apart from the light source, there are in fact 3 objects at play here, not just the As and Bs. We have A, B, and that green thing. The shadow is part of the green thing, not part of B. If you take the green thing away the shadow goes with it and, walla! - you will notice that your eye was right all along. (See my comment under Bad Joke)

The equivocation sneaks in where one switches between referring to the colour of the object and the colour per se, that is, between the colour and a colour. The squares represent themselves, while the grey strip running from A to B represents a colour. Obviously a colour can be the same across the differently coloured objects. How else would the off-white object B show shade? The equivocation is more easily fallen into if you have reductionist inclinations and Adelson was probably a typical scientist in this regard, tending to forget the object and its context, while considering only its parts. ;-)

The Wikipedia Optical Illusion entry is full of this reductionist, Cartesian, ghost-in-the-machine nonsense. It is quite astounding in its archaic quality: "An optical illusion (also called a visual illusion) is characterized by visually perceived images that differ from objective reality. The information gathered by the eye is processed in the brain to give a percept that does not tally with a physical measurement of the stimulus source." So, the "objective reality" is presumed to be only what can be measured by some instrument. The exact opposite is true: humans can perceive correctly where instruments are usually fooled. Any instrument measuring the squares in this diagram would be fooled into thinking that A and B were the same colour, because, like the typical scientist who uses it, it is, shall we say, not inclined to distinguish between the colour of the object and the colour per se, i.e. the colour and a colour. Not so, an ordinary human being with a good pair of eyes.

If I had to concede anything here, it would have to be the power of the diagram. It shows how wrong we can be about a colour when we attempt to imagine it outside of its context. We are more likely to get a colour wrong than we are likely to get the colour of an object wrong. Of course, this is less of a problem for an experienced artist who will easily be able to mix an appropriate colour for the object's context. This lesson was learned a long time ago and there are even miniatures in Gothic manuscripts from the "naive" 1400's which show some sense of shading.

- Andrew van der Merwe, calligrapher --Beachscriber (talk) 05:18, 7 October 2009 (UTC)

Umm, sorry, but you don't get it. It's the same color, same shade, same everything. As I said above try blocking everything but the two squares and it's immediately obvious that it's true. Altairantares (talk) 02:26, 10 November 2009 (UTC)

"If I had to concede anything here, it would have to be the power of the diagram. It shows how wrong we can be about a colour when we attempt to imagine it outside of its context." -- in which you have "conceded" everything, as this article, and the illusion, is all about "the diagram" and people's mistaken perception of it -- there are no objects here! -- 98.108.219.226 (talk) 08:11, 24 February 2010 (UTC)

What you are saying about the colour in question is already obvious to me. I'm sorry I didn't make that clearer. Let me state it outright:
1.) the colour is the same,
2.) but the squares in question are not the same colour,
3.) and this is not a contradiction. There is therefore no optical illusion.

1 and 2 are demonstrable, even obvious. 1 can be demonstrated by blocking out other parts of the scene or by using a colour picker. 2 can be demonstrated by removing the green thing, and thus the shadow. As for 3, in order to see why it is not a contradiction, you have to first see the equivocation.

Read the Wikipedia entry on equivocation [3] and then ask yourself what you are referring to by the word "it" in "It's the same colour." Are you referring to the objects or are you referring to the shadow? Or are you referring to the colour independently of either (the colour per se / that colour as it may be applied to any object / that colour as it may be measured by a colour picker)? Are you able to make the distinction between the colour of an object and the colour per se (in and of itself)? The distinction is there to be made and as long as you do not make it, you will equivocate, and ironically, try to avoid a contradiction which is not there by doing things like "blocking out everything but the two squares".

Ask yourself, are the whitish square and the shadow distinct entities or not? Once you have answered that question, ask yourself what colour is the shadow and what colour is the square? (I'm hoping you will see that you can't rely on your colour picker or blocking out of things for this.) You might also ask yourself if the square can be a different colour because of the shadow? The answer is no. It may be painted grey to represent the presence of the shadow on it's surface but it cannot suddenly be grey itself - no more than it can be black because of an absence of light.

The whitish square and the shadow are distinct entities which a colour picker cannot distinguish between. You, and others here, are able, but refuse to make the distinction, and I suspect it is because you fear the contradiction. If you make the distinction you will have to admit that the whitish square and the shadow are different colours and will have to deal with the fact that your colour picker tells you otherwise. This is a problem, because for some reason you trust your colour picker more than your eye! What you don't realise is that your eye and your colour picker are measuring different things. The eye sees through the shadow and correctly assesses the colour of the square as whitish. The colour picker has a sharp eye, but no brain, so it measures pixels but cannot see the squares and cannot therefore give you the colour of any square.

In fact, the whitish square in shade and the shadow cast over it would have to be different colours in order for the whitish square to be measured the same by a colour picker. That whitish square has to be whitish in order to end up measuring grey #787878 in the shade. If the whitish square in shade was actually grey #787878, the shadow would not leave it grey #787878. It would be measured darker.

As for suggesting that I try "blocking everything but the two squares": its like I saw how you did your card trick, but now you want me to close my eyes so I can be fooled the next time! Sure, this will demonstrate to me what is already obvious: that the colour per se is the same ( I am an artist and would have mixed more or less the same colour in order to achieve the effect of the shadow), but it will not fool me into thinking that the squares in question are the same colour. If you had done all that blocking out before I had seen the whole scene, then sure I might have been fooled, but only because the colour per se happened to be the same and I would have had no visual cues to read what was being presented to me any differently.

The upshot of all this is that the failure to make the distinction between the colour of the squares and colour per se leaves you thinking you have an illusion. The real illusion here is of having an illusion!

I don't know if I can make this any clearer. It's all pretty obvious to me and sometimes that makes it harder to explain.

I think this entry and the Wikipedia entry on optical illusion need to totally rewritten. To start with, the definition needs to be exorcized of the ghost-in-the-machine nonsense that colour pickers or spectrometers are objective while the human eye is not.

- Andrew van der Merwe --Beachscriber (talk) 12:45, 13 November 2009 (UTC)

I'm sorry, but you're still not getting it. This is especially revealed by your statements like, "The whitish square and the shadow are distinct entities which a colour picker cannot distinguish between." Here's the key: There is no whitish square, and there is no shadow! It's a two-dimensional image! You are speaking of this as if we're considering the colors of an actual object struck by light. We are not. Rather, we are considering the colors of a REPRESENTATION of an object, so your entire explanation (and problems with calling it an optical illusion) is one massive category error. Unequivocally, the two colors in this image are precisely identical. The optical illusion occurs precisely because the human mind sometimes has to force itself to see visual representations AS representations, and - to be blunt - I can't recall a more spectacular failure in this regard as demonstrated by your comments above. —Preceding unsigned comment added by 65.51.248.100 (talk) 19:48, 26 January 2010 (UTC)

Sorry, I haven't paid any attention to this for some time.

I'm glad you said it - "REPRESENTATION". Yes, obviously the diagram is a two-dimensional representation, and that begs the question: a representation of what? "an object", you said. Well, what object? May I be forgiven for thinking it represents a chequerboard and cylinder, lit from the side? And that A and B represent chequers on the chequerboard?

Frankly, I am disappointed to have you call it a representation and in the same breath deny the existence of what it represents!
A priori truth: A representation cannot be a representation if there is nothing to represent. It is in the nature of all diagrams, models, drawings, photographs, abstractions, etc. Real or fictitious, a representation must be a representation of something. Its a-priori; in the definition. That is what a representation is. A representation sans object is a two-sided triangle, a line of one point, the sound of one-handed clapping - a bit of nonsense.
A posteriori truth: It is patently obvious that the diagram represents a chequerboard and cylinder lit from the side
Conclusion: and in that context, A is a blackish square while B is a whitish one in shade.

The diagram refers to that and that is what I refer to. Common sense dictates it. When I meet with my architect, I don't discuss the paper he used, the colour of his pen, the fold across the middle. No, I refer to the house, our plans for the house, the size of the windows, the position of the tree, the lighting on the South side, etc. Similarly, in this case, I refer to what the diagram refers to when I say that the chequers are not the same colour. *If* I do refer to the diagram itself, I do certainly say that the colour at position A and B is the same. The difference between you and I is I do not equivocate between the diagram and what it represents. After all, the diagram is two-dimensional - a bunch of pixels - and what it represents is three-dimensional, and it should be quite difficult to confuse the two.

Is it perhaps the abstract quality of the diagram that leads you to think that it represents nothing in particular or nothing at all? Well, I fail to see how that it makes any difference. Whether this is a real chequerboard and cylinder or Bart Simpson's makes no difference at all. Give me the right instrumentation and I will create for you the identical effect in a real-life scene and then you can bring your spectrometer and you can measure the light on A and B and tell everyone how deluded they are ... until I remove the cylinder.

What reminded me about this discussion was seeing the new moon two evenings ago. It exhibits a nice optical illusion whereby it appears to have a slightly brighter outline around its darker, earthshine-lit portion. I can't remember the name of this illusion, but it has to do with the effect of placing areas of slightly darker or lighter colour next to each other. (In fact, there is a second, similar optical illusion there which few people notice, but I won't go into that here.) Is that outline an optical illusion? Yes, because it appears to have a slightly brighter outline, when in fact it does not. Even if you know this, the appearance remains. There is no cause out there for it to appear that way. The outline is an entirely subjective matter. Now compare this optical illusion with our "same colour" diagram: does it exhibit an optical illusion? No, because everything is exactly as it appears to be: squares A and B are different colours - grey and off-white - and this can be demonstrated by simply removing the shadow on B. There is ample, clear, physical reason why a colorimeter reading would produce the same reading despite the fact that A and B are different. The simple reason is the presence of the shadow on B.

The comparison between these two is instructive:
1. In the case of the new moon we have an optical illusion and no REPRESENTATION. We have the moon itself in the sky above us. However, if you insist on having a representation, I'm sure I can produce a photograph or even an abstract computer graphic which exhibits the same illusion. Either way, this shows that whether we are talking about a representation or the reality is irrelevant.
2. In the case of the diagram, the light has been deliberately tuned to have the colour values of a blackish square the same as a whitish square in shade. In real life, this would be an uncommon coincidence and in either case any confusion can be cleared by simply removing the cylinder, and along with it, the shadow. This cannot be done in the case of the new moon. There is absolutely nothing you can do to remove the appearance of the outline. Thus the new moon situation exhibits a real optical illusion which warrants some psychological explanation, while our so-called same colour illusion merely exhibits a confusion, a misunderstanding which can easily be cleared up.

Sorry, scratch "easily" there.

I find your inability to see the equivocation quite exasperating. Feel free to disagree with me, but please, at least try to understand. When you say things like "It's an optical illusion because two areas of the screen appear to be different colors when in fact they are the same color" you demonstrate that you have not at all understood me. At no stage have I said that the areas of the screen are different in colour. The colour in itself is the same. I have acknowledged, even insisted on this numerous times and in numerous ways. It is not the issue. Get used to it. Only when you understand how this is a paradox, not a contradiction, will you have understood the point I am trying to make. And only when you concede the existence of the object represented by the diagram (real or imagined) will you see that there is no optical illusion, only a little bit of surprise to those who have no art training ("Wow, who would have thought you'd have to mix the same colour to paint both black and white squares?!)

Beachscriber (talk) 21:28, 16 July 2010 (UTC)

So basically what you're saying is: a) If you take away all the things that fool your eyes/brain, then they aren't fooled and you therefore don't think it counts as an illusion. b) You think a shadow is an actual, physical thing? and c) That even if this were real, the shadow would not actually change the colour of the square but would only make it appear darker, this would still not be an optical illusion... 213.31.180.126 (talk) 15:04, 2 February 2010 (UTC)

a) No. I'm saying that it doesn't qualify as an optical illusion because there is nothing which appears to be what it is not. Squares A and B are different in exactly the way they look different. We remove the cylinder and its shadow not to prove anything to the eye, but to prove to the colour picker that it is wrong about the squares. Perhaps it will help to think of a colour picker or spectrometer as a pair of blinkers - the sort of thing you'd put on a donkey - only much narrower. When you look at the area occupied by squares A and B with the blinkers on, you don't see the chequer board, the cylinder, the scene in general and this leaves you unable to interpret what you see. All you see is an area which is consistent in colour. You cant even say that A and B are the same colour because you don't see A and B. The fact that both areas seem the same colour to you is not an illusion, but an ignorance, a confusion. This ignorance is forced on you by the way you are instructed to prove the so-called illusion: "By erasing everything except the two labeled parallelograms, the illusion is dispelled." This reminds me of the metaphor of the ostrich with its head in the sand. The realities of life, the context is not going to go away when you hide from it.

b) I don't see what you're getting at. A green cylinder is a physical object capable of casting a shadow.

c) Yes, in the real situation represented by this diagram, there is no optical illusion. It's actually quite funny: the illusion of an optical illusion is had when one confuses the real situation with the diagram. This is on a par with objecting to your architect's plans by saying, no ways, I want a three-dimensional house, please!

Beachscriber (talk) 21:33, 16 July 2010 (UTC)

The equivocation and logic errors are all yours, Beachscriber. It's an optical illusion because two areas of the screen appear to be different colors when in fact they are the same color. This illusion -- this appearance that pixels of the same color are of different color -- is so strong that we have a number of people claiming here that they really are different, that this is just a mockup to show the effect, that people are lying when they say they are the same color ... and then there is you, seeing squares and shadows and cylinders when there are no such things here, only a rendering, and it is the properties of the rendering that are at issue.

You ask what you are referring to by the word "it" in "It's the same colour." Are you referring to the objects or are you referring to the shadow? Or are you referring to the colour independently of either -- we are of course referring to none of those. There are no objects or shadows, only a rendering. And it's nonsensical to talk about a color per se being the same color. No, we are talking about the color of the pixels -- or, if this illusion were presented on paper, the color of the ink at those spots on the paper. They appear to be different when they are actually the same -- hence, an illusion.

Also, you are inverting the meaning of the phrase "ghost in the machine". Humans do not have some magical ability to perceive correctly what instruments fail to register -- a charge that is particularly ridiculous in this case because the two areas of the image are the same color by design; we don't need a color picker to determine that they are the same, we can look at the image data. No, human visual processing is not magical, not ghostly, it is algorithmic, and that algorithm employs heuristics -- which produce illusions.

In any case, this discussion doesn't belong here; it's a violation of WP:NOR. The Wikipedia articles reflect the cited literature, so what you think about the matter is irrelevant. -- 98.108.219.226 (talk) 08:06, 24 February 2010 (UTC)

1. "... and then there is you, seeing squares and shadows and cylinders when there are no such things here, only a rendering, ..." For my response to this, see what I have to say in response to the "REPRESENTATION" objection above. Everything there can be said about a rendering. It begs the question: a rendering of what?

It seems to me that a lot of the inability to understand the point I am trying to make comes from this nonsensical idea that we are not actually talking about a chequerboard and cylinder. The explanation in the article goes so far as to say "There is no three-dimensional object casting a shadow, and there are no squares." Thus the chequerboard and cylinder are an illusion and what we have is an illusion within an illusion! Good grief. How does one respond to such nonsense?! ... I give up. I suspect that even if I do manage to express myself cogently on the matter it would be written off as repetitive arrangement of pixels on a screen.

2. "And it's nonsensical to talk about a color per se being the same color. No, we are talking about the color of the pixels ..." Again, you have not understood my point. The colour of the pixels is what I meant by "the colour per se". I made that clear in my 13 November entry. "Per se" means "in and of itself", "by itself", "standing alone" - in other words, the colour sans its chequerboard and cylinder context, just the pixels. My point turns on the distinction between the colour per se and the colours of the object depicted by the diagram.

3. By "ghost in the machine", I am referring to the model of human nature assumed by the likes of Descarte, David Hume and explicitly expounded by Karl Popper, in which the sense organs take in raw "sense data" which is then interpreted by the mind. This is called dualism. This model inevitably leads to the view that there are no real objects out there (no chequerboards and cylinders) and all your interpretations are subjective and arbitrary. You are clearly operating under the same assumption and I think you might find it interesting to question it. We may never agree on this level, but you may at least come to understand the position I am taking before you write it off. It is unfair of you to pronounce my thoughts on this matter irrelevant without having demonstrated a reasonable understanding of them.

4. "... because the two areas of the image are the same color by design ..." Yes, and I could conceivably, with the aid of the right instrumentation, design and build a real life model which achieves the same effect as this diagram. Then I would like you lot to come and tell me that there is no actual cylinder, no actual squares!

5. As to a potential violation of WP:NOR, you have nothing to worry about because I have no intention of editing the article, and even if I did, I would not do so without first discussing possible amendments in this forum. This page is for discussion; this is where the discussion belongs.

Finally, I apologise for the length of my contributions. I feel like I have not been understood at all and am torn between the need to explain myself thoroughly and the fact that the length of my contributions will result in their not being read. In the end, I'd rather make myself as clear as possible than worry about half-baked responses.

Beachscriber (talk) 23:05, 16 July 2010 (UTC)

You just don't get it

I feel sorry for all you people who think that A is different than B. It is an illusion. The point of an illusion is to make you think that something is different than it really is, and this is doing just that to you. Paperfork ♠ 01:44, 21 March 2010 (UTC)

Paperfork, if you think I don't see that A and B have the same colour reading, same pixel value, then you have not understood a word of what I have said and, I suspect, have not read much of it. I have made it abundantly clear that it is obvious to me that the colour per se / pixel colour / colorimeter reading is the same. In fact, I have a reasonably well-trained eye and don't even need the assistance of a colour-picker to see this. The reason why I am not as a result contradicting myself is because I make the distinction between the colour per se and the colours of the object. I have also said this clearly and often. I make this distinction the same way an artist would when mixing colour to paint an object. It is common knowledge to an artist that the colour one mixes to paint an object is often different to the colour of the object, and this is simply a matter of context and lighting, not an optical illusion.

Beachscriber (talk) 20:46, 16 July 2010 (UTC)

I'd just like to say that I can see where Beachscriber is coming from. What is the REASON that when you look at this image, you see them as different colors? It's because your eyes are adjusting for what they perceive to be shade, in an attempt to see the actual color of the square. This isn't really an optical illusion, and more of a function of the human body. Of course, everything we call an optical illusion could be rejected on these basis, so I'm not advocating changing the article (but then, neither was Beachscriber) I just hope if someone else reads this talk page they'll understand the point. 80.101.97.103 (talk) 16:06, 4 November 2010 (UTC)

I too see here he's coming from and that he did get it, but would like to show the sort of a flip-side to the argument. He's just not right. He is assuming we are seeing with our eyes, but we are seeing with our minds. This is equally an optical ilusion as M.C.Escher's pictures are, or neither are. In the end his argument is argument about definition of the term Optical illusion -- and in that case actually, he's also wrong. —Preceding unsigned comment added by 87.250.108.155 (talk) 13:52, 19 November 2010 (UTC)

80.101.97.103, I have already explained why my objections to this being called an optical illusion do not affect all optical illusions. See my contribution of 16 July 2010 in which I compare this with a real optical illusion.
If I may attempt to clarify the distinction between an optical illusion and a bit of confusion:
My wife and I were once standing surveying the scenery in some wild place when a rabbit broke cover near us and ran off. She cried "Oh, look a bokkie!" (a buck) Was it an optical illusion? No, she just isn't that good at judging space, shape and stuff like that. I'm not particularly good at judging one brand of car from another. When I confuse a Toyota with a Honda, is it due to an optical illusion? No. Do you confuse the type on your screen with the words themselves? Do you make your word processing corrections with Tip-ex?! Ok, obviously not, then why is it suddenly an optical illusion when you confuse the colour of a square with its shading. If night fell, would you consider the white square black?!

--Beachscriber (talk) 13:23, 18 March 2011 (UTC)

87.250.108.155, I don't understand what you are saying about seeing with your mind not your eyes. Ask anyone to give you a common sense reply to the question of what we see with and they will tell you it's our eyes. Of course, in a sense, the eye, on it's own does not see, but then, neither does the mind, or the big toe for that matter. It is *you* who sees, not any part of you. To speak of the eye or the mind seeing is either a figure of speech or a category error.
But I'm very happy to have have you observe that this is about the definition of the term Optical Illusion. It certainly is and it is my sincere wish that this discussion will ultimately lead to a generally accepted revision or qualification of the definition. I'm not quite sure what it will be yet. A lot depends on receiving some contributions from real philosophers who have some experience in this area. We're all just amateurs here.

--Beachscriber (talk) 13:13, 18 March 2011 (UTC)

No, this was not about common sense nor was it philosophical. This entire subject has little to do with philosophy, at best it's about cognitive processes and perception, both covered in great lengths by medicine and more-less hard-scientific branches of psychology and neuro-psychology.

When I said that we are seeing with our mind I specifically meant the biological aspect of seeing being that the eyes capture small bits of the picture you are "witnessing" in front of you sampled one after another and the actual picture is being reconstructed in your visual cortex -- for better or for worse. So, in layman terms, you are seing with your mind. If you could only see the raw image captured by your eyes directly you'd find it very hard to perform even the most mundane tasks in life.

Back to the original subject, regardless of the bit about seeing apparatus, the facts remain: that the squares are of equal colors, that no physical object exists, that those are just flat 2D images -- so you are either wrong by not getting it or wrong in your understanding of what optical illusion, as a term, means. Because no physical objects exist in pictures of M.C.Escher either (only different colored lines and surfaces).

And no you couldn't be able to construct an objective reality that would fool the eye in that manner under all circumstances. Only under very limited lighting conditions and even then it would be a huge bet would it work on how many people. You could go to great lengths to make a photograph of such reality with this same effect, and that would in effect be an optical illusion as it's an artificial visual abstracting the reality in some way.

So, yes, in "objective" reality optical illusion is a totally useless sintagm as you correctly stated. But this image is not objective reality any more or any less than Eschers images are. And in that sense it IS an optical ilusion by premise, because this sort of stuff is exactly what's meant by the (human, culturally invented) term "optical illusion" -- an artificially produced visual, made with specific intent of fooling your visual apparatus into assuming some visual information is something, when in reality it's something entirely different.

Your whole argument fails precisely because there is no paradigmatic difference in intent nor execution of this piece of art compared to other pieces of art we termed optical illusions.

Another place where your misconceptions stem from is that, due to your specific education or otherwise lack of understanding of the concept, is that in any world outside art classes and philosophical discourse, there is no paradigmatic difference in "color" and "shade". Only lexical. —Preceding unsigned comment added by 87.250.108.155 (talk) 13:47, 29 April 2011 (UTC)

The squares are different colors. The letters aren't.

The squares in the illusion are, in fact, different colors. The letters, however, are a different matter. The letters are the same color. That's the illusion. If no-one contests this, I'm going to edit the article in a few days so it says that the letters on the squares are the same color. --- cymru lass ^{(hit me up)}⁄_{(background check)} 01:17, 25 August 2010 (UTC)

And yet another witless victim falls to the illusion. The squares are the same color (#787878), you only think they are different. 203.211.73.64 (talk) 10:29, 29 August 2010 (UTC)

cmru lass, I must protest this change as it will just confuse people more. The squares A and B have the same reading and the colour of the letters is irrelevant. You need to download a color picker - something like ColorCop - and do a precise sampling.

203.211.73.64, It is you who has fallen for the illusion of an illusion. Getting the same colorimeter reading on the whitish square as the blackish square does not does not make the whitish square blackish! That is a silly contradiction on par with calling a square triangular. The reason for the dark reading on the whitish square is simply the presence of the shadow. See my arguments above.

Beachscriber (talk) 08:10, 3 September 2010 (UTC)

Another shot at this

Imagine that instead of having a computer image or drawing of a checkerboard and a cylinder shadowing it, we had an actual checkerboard, cylinder and lamp. In the real checkerboard, we have the corresponding squares labeled A and B. We carefully set up the light intensity, checkerboard square tint and viewer position so that A and B reflect the same total amount of light back to the viewer.

Would you say that the viewer who judged that A is darker than B in this situation is falling for an optical illusion? Heck no. That viewer is correctly judging the color of the squares, understood as their surface reflectivity. Our vision is very good at judging the surface reflectivity under mixed light; if we simultaneously see a black cat in the sunshine and a white cat in the deep shadow under a car, we correctly judge which cat's hair is darker, despite the fact that the eye probably in fact receives more light from the black cat than from the white one.

But the naïve idea that there is a "same color illusion" in the checkboard-and-cylinder image would require us to conclude that the guy who sees the black cat in the sunshine and the white can in the shadow is experiencing an illusion. The black cat would be "objectively brighter" because it's in the sunshine, and thus, reflecting more light than the white one!

Or in other terms: the argument that A and B are "the same color" has this structure:

• The light from points A and B (A ≠ B) that reaches our retina at points A' and B' (A' ≠ B') has the same intensity and spectral composition.
• Ergo, A and B have the same color.

But again, it's trivial to construct examples where the premise is true and the conclusion is wrong. Even worse with the black and white cat example—you can construct examples where the darker point is the one with more light!

There are optical illusions in the image, but they're really just the illusions of depth and light. The image is seen as a depiction of a physical scene where square A is darker than B—i.e., where square A reflects a smaller percentage of the light that falls on it than B. In fact, you have to judge A as being darker than B to correctly see this image as a depiction of a scene, and not just a bunch of colored patches—just as you have to judge those irregular parallelograms as squares to perceive the perspective rendering as a checkerboard. 63.80.102.10 (talk) 23:50, 11 April 2011 (UTC)

One thing you might consider doing is posting a question like this on the Science reference desk. Bus stop (talk) 02:04, 12 April 2011 (UTC)

I do not see where the OP is asking a question. 86.148.155.92 (talk) 11:33, 26 June 2012 (UTC)

Moved and cleaned up names

I looked at the history, and sources, and couldn't find any sources calling it the "same color illusion" or "Adelson's checker shadow illusion" from before this article called it those things. Just "checker shadow illusion", or in a few cases preceded by the indentification as "Ted Adelson's" or "Edward Adelson's"; never just "Adelson's". So I simplified it and moved it what it's always called in sources, neglecting those recent ones that copied from wikipedia. Dicklyon (talk) 22:32, 17 April 2011 (UTC)

Possible explanation and example

I've come to this rather late I know, but I see there is still some activity here. It seems to me that the Explanation of the effect is OK, but I'll have another go. If you can, ignore the shadow effect. Look at the image as two squares: A is surrounded by light grey and B is surrounded by very dark grey to black. The shadow effect merely makes the surroundings of B even darker. It is known that, a shade surrounded by much lighter shades looks darker, while the same shade, surrounded by darker shades looks lighter. Sadly, the illusion I remember from 1st year Psychology a million years ago doesn't seem to be in WP - I'll look for it. However, a similar effect is shown by the picture in the article showing the two coloured circles on the checkerboard, and the colour-gradient effect. I've tried this in MS Paint, just using two grey squares, one surrounded with white, and the other surrounded by black. The two squares do look like different shades, but they are not. I did the same with two yellow circles too - same effect. Myrvin (talk) 13:58, 14 July 2014 (UTC)

I also tried another way to convince myself that the squares really are the same shade. Save the image as a .png file. Open this in MS Paint (or equivalent). Using the Free-form select tool, describe a chunk of square A. This can then be cut and pasted to a white area. Do the same with square B and put its section next to the section taken from square A. They look exactly the same shade. Myrvin (talk) 16:45, 14 July 2014 (UTC)

See the image.

Grey square optical illusion cut 2

Convinced? If you think I've fiddled something, try it yourself. Myrvin (talk) 17:32, 14 July 2014 (UTC)

I see this article is still annoying people. Maybe I have an idea about why the illusion is so powerful on a computer screen. On my LED screen, if you look at the image from low down, the whole thing looks darker. Looking at the screen at right angles to it, it lightens. Also, when you look at it from high up, the whole image (I guess the whole screen) is lighter. Now, since square A is higher that square B, A is going to look darker than B. I don't think this is an illusion, it must be something about light coming from the LEDs at different angles, or maybe it has to do with light reflecting from the screen. So, I printed out my image of the illusion. It looked to me as if the illusion still happened, but maybe not so strongly. Then I did what the vituperative editor below did with the two little holes, covering everything except the squares in question. The illusion disappears. Still a strong illusion, but today's computer screens may not be the best way to display it. By the way, I find it amusing how many critics say that the two squares are "obviously" different. Any illusion works "obviously", that's what an illusion is. Something that obviously looks like this, but is in fact really that. Look at the Ponzo illusion and the Müller-Lyer illusion. The top line is obviously different in length to the bottom, but they are not. Myrvin (talk) 08:31, 15 July 2014 (UTC)

The emperor has no clothes

This article is really funny. Obviously, the two squares have different colors. Anyone can see that. Giving this article the benefit of the doubt, I cut two little holes in a piece of paper, and took a look. Sure enough. Different colors! What a joke. There are famous psychology experiments that show how sheepishly people believe any kind of old nonsense, as long as it has the stamp of authority and other "subjects" (actually part of the experiment) believe it. Obviously, this article is not an experiment. It's a result of the incredibly flawed Wikipedia process. But the result is the same. I find it amusing how people believe anything they read or see on Wikipedia. If Wikipedia said 2 + 2 = 5, people would believe it. There is a sucker born every minute, and apparently gets their information from Wikipedia. If some wanted to make a satire web site of Wikipedia, they couldn't do any better than the original. Don't get me wrong. If I want to get the details on an episode of Buffy the Vampire Slayer, Wikipedia is my first choice. Wikipedia has a wealth of information on everything that does not matter. But for anything of a scientific or technical nature, Wikipedia is not a reliable source. This article is just one particularly striking example. 71.217.116.182 (talk) 23:33, 14 July 2014 (UTC)

Instead of just being critical, why not add some information you feel is missing? Wikipedia is only as good as the people who edit it. Not everyone is a scientist or engineer. As this comment has little to do with this article, I would suggest any further statements of dissatisfaction with Wikipedia be placed elsewhere. 331dot (talk) 23:34, 14 July 2014 (UTC)

More optical illusions?

Hello. Is there any reason to believe that the "Explanation" section is not a copyright violation and that the "Variations of the illusion" section is not original research? Thanks and regards, Biwom (talk) 07:02, 16 November 2017 (UTC)

So, after a 24-hour delay, I have removed both sections. Thanks and regards, Biwom (talk) 08:14, 17 November 2017 (UTC)

I have rolled back your edits. I don't think the section quoting the author of the illusion is a CV - it makes the correct attribution. And as for OR, that calls for some discussion. Eusebeus (talk) 10:24, 19 November 2017 (UTC)

Hello. I think our policies about copyvio and unsourced content are clear. If you want this in the article, you need to reword the "Explanations" and find sources for the "Variations". Thanks and regards, Biwom (talk) 10:41, 19 November 2017 (UTC)

And in an attempt to kill any discussion before it has even started, let me add this. The reason I strongly believe "Variations" is OR is because it actually contradicts what Adelson says in the copyvio quote. So on one hand, you have Adelson who, with I believe some legitimacy, tells you the illusion works because the cylinder is visible and your brain interprets the darker central zone as its shadow. And on the other hand, you have a new contributor with zero edit and zero source who tells you "most of the board and even the cylinder are actually redundant". Really? Well sorry, but no. So let me add WP:UNDUE and WP:FRINGE to WP:OR and WP:PROVEIT. Thanks and regards, Biwom (talk) 16:47, 19 November 2017 (UTC)

Hi,
Sorry I wasn't aware of this OR restriction. "Variations" was my own work, so I guess I'll just have to get it published somewhere first.
As to it contradicting Adelson, I didn't notice that he said the cylinder had to be in view (as opposed to being implied by its shadow).
As to my claim "most of the board and even the cylinder are actually redundant" and your comment "Really? Well sorry, but no" - I wonder did you even look at the picture I posted? If not I suggest you look again. I think you'll see the illusion remains very clear despite the fact that the cylinder is not in view.

Minimal version of checker shadow illusion

Davblo2 (talk) 10:49, 1 April 2018 (UTC)

Possible mistake after SVG conversion

I am unsure if at some point when the images got converted to SVG the examples didn't get converted right, or are displaying wrong. When I use a color picker on the File:Checker_shadow_illusion.svg the color of the A square is #6F6F6F while the color of the B square is #818181. On File:Grey_square_optical_illusion_proof2.svg both squares are #787877, and the illusion works when I remove the connecting bridge. I am unsure now if this is some quirk of how my browser (Chrome) and editing program (Affinity Designer) render the shadow or a mistake in the first example. I double-checked and what I'm picking is the displayed color after all SVG layers were mixed together. WhiteRailgun (talk) 19:58, 4 September 2019 (UTC)

Prior to 25-Dec-2018 both thumbnail images were identical except for the added rectangle. Ever since the replacement of File:Grey_square_optical_illusion.svg with File:Checker_shadow_illusion.svg, the upper thumbnail is now a bit larger and darker than the lower. Unknown what that accomplished other than complicating any direct comparison. My color picker shows #5C5C5C for both A and B squares in the current upper image, and #656564 for both A and B squares in the lower. I was unable to reproduce your A vs B difference. Rairden (talk) 22:10, 8 July 2020 (UTC)

Featured picture scheduled for POTD

Hello! This is to let editors know that File:Checker_shadow_illusion.svg, a featured picture used in this article, has been selected as the English Wikipedia's picture of the day (POTD) for June 14, 2022. A preview of the POTD is displayed below and can be edited at Template:POTD/2022-06-14. For the greater benefit of readers, any potential improvements or maintenance that could benefit the quality of this article should be done before its scheduled appearance on the Main Page. If you have any concerns, please place a message at Wikipedia talk:Picture of the day. Thank you! Adam Cuerden ^(talk)_{Has about 7.8% of all FPs} 13:39, 23 May 2022 (UTC)

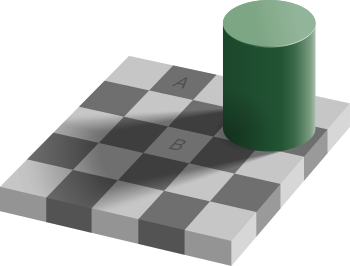
The checker shadow illusion is an optical illusion published in 1995 by Edward Adelson, an American professor of vision science at the Massachusetts Institute of Technology. The phenomenon features an image of a checkerboard with light and dark squares, partly shadowed by another object, such as a cylinder as in this illustration. The optical illusion is that the area labeled A appears to be darker than the area labeled B. However, within the context of the two-dimensional image, they are of identical brightness – in other words, they would be printed with identical mixtures of ink, or displayed on a screen with pixels of identical color.

Optical illusion credit: Edward Adelson; illustrated by Pbrks

Recently featured:

• Danube Delta
• Notre-Dame fire
• Julia Margaret Cameron

The featured picture, the current article picture, as of right now, does not demonstrate the phenomenon. The pixels in the A & B squares are not identical. Other versions of the image did have this, but the current one does not. — Preceding unsigned comment added by 2600:1015:B04B:56EF:95B2:EA7F:CC76:3731 (talk) 08:37, 14 June 2022 (UTC)

Hi. I’m not quite sure what you mean. The point is that there is a perceived shadow and grey values in the shadow need to be darker to give the same impression.

I have checked the grey values in Photoshop (save image in a raster format like png; then open in Photoshop and use the pipette tool):

White background: 255, 255, 255

White square in the “shadow”: 120, 120, 119

White square outside the “shadow”: 205, 205, 205

Black square inside the “shadow”: 62, 62, 62, to 67, 67, 67

Black square outside the “shadow”: 120, 120, 119

As you can see, the white squares in the perceived shadow (g=120) have the same grey value as the black squares outside the shadow (also g=120).

Thus, the physical grey values do not translate to perceived black/white. The “bridge” between A and B (in a previous graph version) does break the illusion; if you cover up the bridge with something, the illusion returns. Strasburger (talk) 10:00, 25 June 2022 (UTC)