Ellipse tangent to all sides of a triangle
Example of an inellipse In triangle geometry , an inellipse is an ellipse that touches the three sides of a triangle . The simplest example is the incircle . Further important inellipses are the Steiner inellipse , which touches the triangle at the midpoints of its sides, the Mandart inellipse and Brocard inellipse (see examples section ). For any triangle there exist an infinite number of inellipses.
The Steiner inellipse plays a special role: Its area is the greatest of all inellipses.
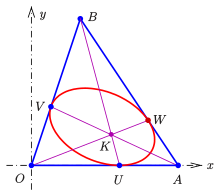
Because a non-degenerate conic section is uniquely determined by five items out of the sets of vertices and tangents, in a triangle whose three sides are given as tangents one can specify only the points of contact on two sides. The third point of contact is then uniquely determined.
[ edit ] An inellipse of a triangle is uniquely determined by the vertices of the triangle and two points of contact
U
,
V
{\displaystyle U,V}
The inellipse of the triangle with vertices
O
=
(
0
,
0
)
,
A
=
(
a
1
,
a
2
)
,
B
=
(
b
1
,
b
2
)
{\displaystyle O=(0,0),\;A=(a_{1},a_{2}),\;B=(b_{1},b_{2})}
and points of contact
U
=
(
u
1
,
u
2
)
,
V
=
(
v
1
,
v
2
)
{\displaystyle U=(u_{1},u_{2}),\;V=(v_{1},v_{2})}
on
O
A
{\displaystyle OA}
O
B
{\displaystyle OB}
rational parametric representation
(
4
u
1
ξ
2
+
v
1
a
b
4
ξ
2
+
4
ξ
+
a
b
,
4
u
2
ξ
2
+
v
2
a
b
4
ξ
2
+
4
ξ
+
a
b
)
,
−
∞
<
ξ
<
∞
,
{\displaystyle \left({\frac {4u_{1}\xi ^{2}+v_{1}ab}{4\xi ^{2}+4\xi +ab}},{\frac {4u_{2}\xi ^{2}+v_{2}ab}{4\xi ^{2}+4\xi +ab}}\right)\ ,\ -\infty <\xi <\infty \ ,}
where
a
,
b
{\displaystyle a,b}
a
=
1
s
−
1
,
u
i
=
s
a
i
,
b
=
1
t
−
1
,
v
i
=
t
b
i
,
0
<
s
,
t
<
1
.
{\displaystyle a={\frac {1}{s-1}},\ u_{i}=sa_{i},\quad b={\frac {1}{t-1}},\ v_{i}=tb_{i}\;,\ 0<s,t<1\;.}
The third point of contact is
W
=
(
u
1
a
+
v
1
b
a
+
b
+
2
,
u
2
a
+
v
2
b
a
+
b
+
2
)
.
{\displaystyle W=\left({\frac {u_{1}a+v_{1}b}{a+b+2}}\;,\;{\frac {u_{2}a+v_{2}b}{a+b+2}}\right)\;.}
The center of the inellipse is
M
=
a
b
a
b
−
1
(
u
1
+
v
1
2
,
u
2
+
v
2
2
)
.
{\displaystyle M={\frac {ab}{ab-1}}\left({\frac {u_{1}+v_{1}}{2}},{\frac {u_{2}+v_{2}}{2}}\right)\;.}
The vectors
f
→
1
=
1
2
a
b
a
b
−
1
(
u
1
+
v
1
,
u
2
+
v
2
)
{\displaystyle {\vec {f}}_{1}={\frac {1}{2}}{\frac {\sqrt {ab}}{ab-1}}\;(u_{1}+v_{1},u_{2}+v_{2})}
f
→
2
=
1
2
a
b
a
b
−
1
(
u
1
−
v
1
,
u
2
−
v
2
)
{\displaystyle {\vec {f}}_{2}={\frac {1}{2}}{\sqrt {\frac {ab}{ab-1}}}\;(u_{1}-v_{1},u_{2}-v_{2})\;}
are two conjugate half diameters and the inellipse has the more common trigonometric parametric representation
x
→
=
O
M
→
+
f
→
1
cos
φ
+
f
→
2
sin
φ
.
{\displaystyle {\vec {x}}={\vec {OM}}+{\vec {f}}_{1}\cos \varphi +{\vec {f}}_{2}\sin \varphi \;.}
Brianchon point
K
{\displaystyle K}
The Brianchon point
K
{\displaystyle K}
A
V
¯
,
B
U
¯
,
O
W
¯
{\displaystyle {\overline {AV}},{\overline {BU}},{\overline {OW}}}
K
:
(
u
1
a
+
v
1
b
a
+
b
+
1
,
u
2
a
+
v
2
b
a
+
b
+
1
)
.
{\displaystyle K:\left({\frac {u_{1}a+v_{1}b}{a+b+1}}\;,\;{\frac {u_{2}a+v_{2}b}{a+b+1}}\right)\ .}
Varying
s
,
t
{\displaystyle s,t}
U
,
V
{\displaystyle U,V}
s
,
t
{\displaystyle s,t}
a
,
b
{\displaystyle a,b}
−
∞
<
a
,
b
<
−
1
{\displaystyle -\infty <a,b<-1}
Remark: The parameters
a
,
b
{\displaystyle a,b}
Mandart inellipse For
s
=
t
=
1
2
{\displaystyle s=t={\tfrac {1}{2}}}
U
,
V
,
W
{\displaystyle U,V,W}
Steiner inellipse (its center is the triangle's centroid).
For
s
=
|
O
A
|
+
|
O
B
|
−
|
A
B
|
2
|
O
A
|
,
t
=
|
O
A
|
+
|
O
B
|
−
|
A
B
|
2
|
O
B
|
{\displaystyle s={\tfrac {|OA|+|OB|-|AB|}{2|OA|}},\;t={\tfrac {|OA|+|OB|-|AB|}{2|OB|}}}
incircle of the triangle with center
O
M
→
=
|
O
B
|
O
A
→
+
|
O
A
|
O
B
→
|
O
A
|
+
|
O
B
|
+
|
A
B
|
.
{\displaystyle {\vec {OM}}={\frac {|OB|{\vec {OA}}+|OA|{\vec {OB}}}{|OA|+|OB|+|AB|}}\;.}
For
s
=
|
O
A
|
−
|
O
B
|
+
|
A
B
|
2
|
O
A
|
,
t
=
−
|
O
A
|
+
|
O
B
|
+
|
A
B
|
2
|
O
B
|
{\displaystyle s={\tfrac {|OA|-|OB|+|AB|}{2|OA|}},\;t={\tfrac {-|OA|+|OB|+|AB|}{2|OB|}}}
Mandart inellipse of the triangle. It touches the sides at the points of contact of the excircles (see diagram).
Brocard inellipse For
s
=
|
O
B
|
2
|
O
B
|
2
+
|
A
B
|
2
,
t
=
|
O
A
|
2
|
O
A
|
2
+
|
A
B
|
2
{\displaystyle \ s={\tfrac {|OB|^{2}}{|OB|^{2}+|AB|^{2}}}\;,\quad t={\tfrac {|OA|^{2}}{|OA|^{2}+|AB|^{2}}}\;}
Brocard inellipse . It is uniquely determined by its Brianchon point given in trilinear coordinates
K
:
(
|
O
B
|
:
|
O
A
|
:
|
A
B
|
)
{\displaystyle \ K:(|OB|:|OA|:|AB|)\ }
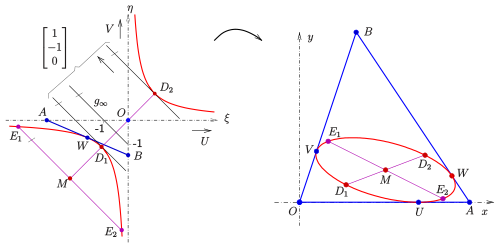
Derivations of the statements [ edit ] Determination of the inellipse by solving the problem for a hyperbola in an
ξ
{\displaystyle \xi }
η
{\displaystyle \eta }
x -y -plane.
M
{\displaystyle M}
D
1
D
2
,
E
1
E
2
{\displaystyle D_{1}D_{2},\;E_{1}E_{2}}
g
∞
{\displaystyle g_{\infty }}
x -y -plane. New coordinates
For the proof of the statements one considers the task projectively
ξ
{\displaystyle \xi }
η
{\displaystyle \eta }
hyperbola and the points
U
,
V
{\displaystyle U,V}
A
=
(
a
1
,
a
2
)
,
B
=
(
b
1
,
b
2
)
{\displaystyle A=(a_{1},a_{2}),\;B=(b_{1},b_{2})}
A
=
[
a
,
0
]
,
B
=
[
0
,
b
]
{\displaystyle A=[a,0],B=[0,b]}
ξ
a
+
η
b
=
1
{\displaystyle {\frac {\xi }{a}}+{\frac {\eta }{b}}=1}
a
,
b
{\displaystyle a,b}
A
B
¯
{\displaystyle {\overline {AB}}}
η
=
a
b
4
ξ
{\displaystyle \eta ={\frac {ab}{4\xi }}}
A
B
¯
{\displaystyle {\overline {AB}}}
W
=
[
a
2
,
b
2
]
{\displaystyle W=[{\tfrac {a}{2}},{\tfrac {b}{2}}]}
Coordinate transformation
The transformation of the solution into the x -y -plane will be done using homogeneous coordinates
[
u
1
v
1
0
u
2
v
2
0
1
1
1
]
{\displaystyle {\begin{bmatrix}u_{1}&v_{1}&0\\u_{2}&v_{2}&0\\1&1&1\end{bmatrix}}\quad }
A point
[
x
1
,
x
2
,
x
3
]
{\displaystyle [x_{1},x_{2},x_{3}]}
[
u
1
v
1
0
u
2
v
2
0
1
1
1
]
[
x
1
x
2
x
3
]
=
(
u
1
x
1
+
v
1
x
2
u
2
x
1
+
v
2
x
2
x
1
+
x
2
+
x
3
)
→
(
u
1
x
1
+
v
1
x
2
x
1
+
x
2
+
x
3
,
u
2
x
1
+
v
2
x
2
x
1
+
x
2
+
x
3
)
,
if
x
1
+
x
2
+
x
3
≠
0.
{\displaystyle {\begin{bmatrix}u_{1}&v_{1}&0\\u_{2}&v_{2}&0\\1&1&1\end{bmatrix}}{\begin{bmatrix}x_{1}\\x_{2}\\x_{3}\end{bmatrix}}={\begin{pmatrix}u_{1}x_{1}+v_{1}x_{2}\\u_{2}x_{1}+v_{2}x_{2}\\x_{1}+x_{2}+x_{3}\end{pmatrix}}\rightarrow \left({\frac {u_{1}x_{1}+v_{1}x_{2}}{x_{1}+x_{2}+x_{3}}}\;,\;{\frac {u_{2}x_{1}+v_{2}x_{2}}{x_{1}+x_{2}+x_{3}}}\right),\quad {\text{if }}x_{1}+x_{2}+x_{3}\neq 0.}
A point
[
ξ
,
η
]
{\displaystyle [\xi ,\eta ]}
ξ
{\displaystyle \xi }
η
{\displaystyle \eta }
[
ξ
,
η
,
1
]
T
{\displaystyle [\xi ,\eta ,1]^{T}}
homogeneous coordinates ). A point at infinity is represented by
[
⋯
,
⋯
,
0
]
T
{\displaystyle [\cdots ,\cdots ,0]^{T}}
Coordinate transformation of essential points
U
:
[
1
,
0
,
0
]
T
→
(
u
1
,
u
2
)
,
V
:
[
0
,
1
,
0
]
T
→
(
v
1
,
v
2
)
,
{\displaystyle U:\ [1,0,0]^{T}\ \rightarrow \ (u_{1},u_{2})\ ,\quad V:\ [0,1,0]^{T}\ \rightarrow \ (v_{1},v_{2})\ ,}
O
:
[
0
,
0
]
→
(
0
,
0
)
,
A
:
[
a
,
0
]
→
(
a
1
,
a
2
)
,
B
:
[
0
,
b
]
→
(
b
1
,
b
2
)
,
{\displaystyle O:\ [0,0]\ \rightarrow \ (0,0)\ ,\quad A:\ [a,0]\rightarrow \ (a_{1},a_{2})\ ,\quad B:\ [0,b]\rightarrow \ (b_{1},b_{2})\ ,}
(One should consider:
a
=
1
s
−
1
,
u
i
=
s
a
i
,
b
=
1
t
−
1
,
v
i
=
t
b
i
{\displaystyle \ a={\tfrac {1}{s-1}},\ u_{i}=sa_{i},\quad b={\tfrac {1}{t-1}},\ v_{i}=tb_{i}\;}
g
∞
:
ξ
+
η
+
1
=
0
{\displaystyle g_{\infty }:\xi +\eta +1=0\ }
x -y -plane; its point at infinity is
[
1
,
−
1
,
0
]
T
{\displaystyle [1,-1,0]^{T}}
[
1
,
−
1
,
0
]
T
→
(
u
1
−
v
1
,
u
2
−
v
2
,
0
)
T
{\displaystyle [1,-1,{\color {red}0}]^{T}\ \rightarrow \ (u_{1}-v_{1},u_{2}-v_{2},{\color {red}0})^{T}}
Hence the point at infinity of
g
∞
{\displaystyle g_{\infty }}
ξ
{\displaystyle \xi }
η
{\displaystyle \eta }
x -y -plane. That means: The two tangents of the hyperbola, which are parallel to
g
∞
{\displaystyle g_{\infty }}
x -y -plane, too. Their points of contact are
D
i
:
[
±
a
b
2
,
±
a
b
2
]
→
1
2
±
a
b
1
±
a
b
(
u
1
+
v
1
,
u
2
+
v
2
)
,
{\displaystyle D_{i}:\left[{\frac {\pm {\sqrt {ab}}}{2}},{\frac {\pm {\sqrt {ab}}}{2}}\right]\ \rightarrow \ {\frac {1}{2}}{\frac {\pm {\sqrt {ab}}}{1\pm {\sqrt {ab}}}}\;(u_{1}+v_{1},u_{2}+v_{2}),\;}
Because the ellipse tangents at points
D
1
,
D
2
{\displaystyle D_{1},D_{2}}
D
1
D
2
{\displaystyle D_{1}D_{2}}
diameter and its midpoint the center
M
{\displaystyle M}
M
:
1
2
a
b
a
b
−
1
(
u
1
+
v
1
,
u
2
+
v
2
)
.
{\displaystyle M:\ {\frac {1}{2}}{\frac {ab}{ab-1}}\left(u_{1}+v_{1},u_{2}+v_{2}\right)\;.}
One easily checks, that
M
{\displaystyle M}
ξ
{\displaystyle \xi }
η
{\displaystyle \eta }
M
:
[
−
a
b
2
,
−
a
b
2
]
.
{\displaystyle \ M:\;\left[{\frac {-ab}{2}},{\frac {-ab}{2}}\right]\;.}
In order to determine the diameter of the ellipse, which is conjugate to
D
1
D
2
{\displaystyle D_{1}D_{2}}
ξ
{\displaystyle \xi }
η
{\displaystyle \eta }
E
1
,
E
2
{\displaystyle E_{1},E_{2}}
M
{\displaystyle M}
ξ
+
η
+
a
b
=
0
{\displaystyle \xi +\eta +ab=0}
E
i
:
[
−
a
b
±
a
b
(
a
b
−
1
)
2
,
−
a
b
∓
a
b
(
a
b
−
1
)
2
]
{\displaystyle E_{i}:\left[{\tfrac {-ab\pm {\sqrt {ab(ab-1)}}}{2}},{\tfrac {-ab\mp {\sqrt {ab(ab-1)}}}{2}}\right]}
x -y -coordinates:
E
i
=
1
2
a
b
a
b
−
1
(
u
1
+
v
1
,
u
2
+
v
2
)
±
1
2
a
b
(
a
b
−
1
)
a
b
−
1
(
u
1
−
v
1
,
u
2
−
v
2
)
,
{\displaystyle \ E_{i}={\frac {1}{2}}{\frac {ab}{ab-1}}\left(u_{1}+v_{1},u_{2}+v_{2}\right)\pm {\frac {1}{2}}{\frac {\sqrt {ab(ab-1)}}{ab-1}}\left(u_{1}-v_{1},u_{2}-v_{2}\right)\;,}
From the two conjugate diameters
D
1
D
2
,
E
1
E
2
{\displaystyle D_{1}D_{2},E_{1}E_{2}}
conjugate half diameters
f
→
1
=
M
D
1
→
=
1
2
a
b
a
b
−
1
(
u
1
+
v
1
,
u
2
+
v
2
)
f
→
2
=
M
E
1
→
=
1
2
a
b
a
b
−
1
(
u
1
−
v
1
,
u
2
−
v
2
)
{\displaystyle {\begin{aligned}{\vec {f}}_{1}&={\vec {MD_{1}}}={\frac {1}{2}}{\frac {\sqrt {ab}}{ab-1}}\;(u_{1}+v_{1},u_{2}+v_{2})\\[6pt]{\vec {f}}_{2}&={\vec {ME_{1}}}={\frac {1}{2}}{\sqrt {\frac {ab}{ab-1}}}\;(u_{1}-v_{1},u_{2}-v_{2})\;\end{aligned}}}
and at least the trigonometric parametric representation of the inellipse:
x
→
=
O
M
→
+
f
→
1
cos
φ
+
f
→
2
sin
φ
.
{\displaystyle {\vec {x}}={\vec {OM}}+{\vec {f}}_{1}\cos \varphi +{\vec {f}}_{2}\sin \varphi \;.}
Analogously to the case of a Steiner ellipse one can determine semiaxes, eccentricity, vertices, an equation in x -y -coordinates and the area of the inellipse.
The third touching point
W
{\displaystyle W}
A
B
{\displaystyle AB}
W
:
[
a
2
,
b
2
]
→
(
u
1
a
+
v
1
b
a
+
b
+
2
,
u
2
a
+
v
2
b
a
+
b
+
2
)
.
{\displaystyle W:\left[{\frac {a}{2}},{\frac {b}{2}}\right]\ \rightarrow \ \left({\frac {u_{1}a+v_{1}b}{a+b+2}}\;,\;{\frac {u_{2}a+v_{2}b}{a+b+2}}\right)\;.}
The Brianchon point of the inellipse is the common point
K
{\displaystyle K}
A
V
¯
,
B
U
¯
,
O
W
¯
{\displaystyle {\overline {AV}},{\overline {BU}},{\overline {OW}}}
ξ
{\displaystyle \xi }
η
{\displaystyle \eta }
ξ
=
a
,
η
=
b
,
a
η
−
b
ξ
=
0
{\displaystyle \xi =a\;,\;\eta =b\;,\;a\eta -b\xi =0}
K
{\displaystyle K}
K
:
[
a
,
b
]
→
(
u
1
a
+
v
1
b
a
+
b
+
1
,
u
2
a
+
v
2
b
a
+
b
+
1
)
.
{\displaystyle K:\ [a,b]\ \rightarrow \ \left({\frac {u_{1}a+v_{1}b}{a+b+1}}\;,\;{\frac {u_{2}a+v_{2}b}{a+b+1}}\right)\ .}
Transforming the hyperbola
η
=
a
b
4
ξ
{\displaystyle \ \eta ={\frac {ab}{4\xi }}}
rational parametric representation of the inellipse:
[
ξ
,
a
b
4
ξ
]
→
(
4
u
1
ξ
2
+
v
1
a
b
4
ξ
2
+
4
ξ
+
a
b
,
4
u
2
ξ
2
+
v
2
a
b
4
ξ
2
+
4
ξ
+
a
b
)
,
−
∞
<
ξ
<
∞
.
{\displaystyle \left[\xi ,{\frac {ab}{4\xi }}\right]\ \rightarrow \ \left({\frac {4u_{1}\xi ^{2}+v_{1}ab}{4\xi ^{2}+4\xi +ab}},{\frac {4u_{2}\xi ^{2}+v_{2}ab}{4\xi ^{2}+4\xi +ab}}\right)\ ,\ -\infty <\xi <\infty \ .}
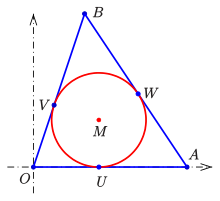
Incircle
Incircle of a triangle For the incircle there is
|
O
U
|
=
|
O
V
|
{\displaystyle |OU|=|OV|}
(1)
s
|
O
A
|
=
t
|
O
B
|
.
{\displaystyle \;s|OA|=t|OB|\;.\ }
(2)
(
1
−
s
)
|
O
A
|
+
(
1
−
t
)
|
O
B
|
=
|
A
B
|
{\displaystyle \;(1-s)|OA|+(1-t)|OB|=|AB|}
Solving these two equations for
s
,
t
{\displaystyle s,t}
(3)
s
=
|
O
A
|
+
|
O
B
|
−
|
A
B
|
2
|
O
A
|
,
t
=
|
O
A
|
+
|
O
B
|
−
|
A
B
|
2
|
O
B
|
.
{\displaystyle \;s={\frac {|OA|+|OB|-|AB|}{2|OA|}},\;t={\frac {|OA|+|OB|-|AB|}{2|OB|}}\;.}
In order to get the coordinates of the center one firstly calculates using (1) und (3)
1
−
1
a
b
=
1
−
(
s
−
1
)
(
t
−
1
)
=
−
s
t
+
s
+
t
=
⋯
=
s
2
(
|
O
B
|
(
|
O
A
|
+
|
O
B
|
+
|
A
B
|
)
.
{\displaystyle 1-{\frac {1}{ab}}=1-(s-1)(t-1)=-st+s+t=\cdots ={\frac {s}{2(|OB|}}(|OA|+|OB|+|AB|)\;.}
Hence
O
M
→
=
|
O
B
|
s
(
|
O
A
|
+
|
O
B
|
+
|
A
B
|
)
(
s
O
A
→
+
t
O
B
→
)
=
⋯
=
|
O
B
|
O
A
→
+
|
O
A
|
O
B
→
|
O
A
|
+
|
O
B
|
+
|
A
B
|
.
{\displaystyle {\vec {OM}}={\frac {|OB|}{s(|OA|+|OB|+|AB|)}}\;(s{\vec {OA}}+t{\vec {OB}})=\cdots ={\frac {|OB|{\vec {OA}}+|OA|{\vec {OB}}}{|OA|+|OB|+|AB|}}\;.}
Mandart inellipse The parameters
s
,
t
{\displaystyle s,t}
de: Ankreis ).
Brocard inellipse The Brocard inellipse of a triangle is uniquely determined by its Brianchon point given in trilinear coordinates
K
:
(
|
O
B
|
:
|
O
A
|
:
|
A
B
|
)
{\displaystyle \ K:(|OB|:|OA|:|AB|)\ }
[ 1]
K
:
k
1
O
A
→
+
k
2
O
B
→
{\displaystyle \ K:k_{1}{\vec {OA}}+k_{2}{\vec {OB}}\ }
trilinear coordinates ) yields
k
1
=
|
O
B
|
2
|
O
B
|
2
+
|
O
A
|
2
+
|
A
B
|
2
,
k
2
=
|
O
A
|
2
|
O
B
|
2
+
|
O
A
|
2
+
|
A
B
|
2
{\displaystyle \ k_{1}={\tfrac {|OB|^{2}}{|OB|^{2}+|OA|^{2}+|AB|^{2}}},\;k_{2}={\tfrac {|OA|^{2}}{|OB|^{2}+|OA|^{2}+|AB|^{2}}}\ }
s
,
t
{\displaystyle s,t}
K
{\displaystyle K}
k
1
=
s
(
t
−
1
)
s
t
−
1
,
k
2
=
t
(
s
−
1
)
s
t
−
1
{\displaystyle \ k_{1}={\tfrac {s(t-1)}{st-1}},\;k_{2}={\tfrac {t(s-1)}{st-1}}\ }
k
1
,
k
2
{\displaystyle k_{1},k_{2}}
s
,
t
{\displaystyle s,t}
s
=
|
O
B
|
2
|
O
B
|
2
+
|
A
B
|
2
,
t
=
|
O
A
|
2
|
O
A
|
2
+
|
A
B
|
2
.
{\displaystyle s={\frac {|OB|^{2}}{|OB|^{2}+|AB|^{2}}}\;,\quad t={\frac {|OA|^{2}}{|OA|^{2}+|AB|^{2}}}\;.}
Inellipse with the greatest area [ edit ] The Steiner inellipse has the greatest area of all inellipses of a triangle. Proof
From Apollonios theorem on properties of conjugate semi diameters
f
→
1
,
f
→
2
{\displaystyle {\vec {f}}_{1},{\vec {f}}_{2}}
F
=
π
|
det
(
f
→
1
,
f
→
2
)
|
{\displaystyle F=\pi \left|\det({\vec {f}}_{1},{\vec {f}}_{2})\right|\quad }
Steiner ellipse ).For the inellipse with parameters
s
,
t
{\displaystyle s,t}
det
(
f
→
1
,
f
→
2
)
=
1
4
a
b
(
a
b
−
1
)
3
/
2
det
(
s
a
→
+
t
b
→
,
s
a
→
−
t
b
→
)
{\displaystyle \det({\vec {f}}_{1},{\vec {f}}_{2})={\frac {1}{4}}{\frac {ab}{(ab-1)^{3/2}}}\det(s{\vec {a}}+t{\vec {b}},s{\vec {a}}-t{\vec {b}})}
=
1
2
s
s
−
1
t
t
−
1
(
1
−
(
s
−
1
)
(
t
−
1
)
)
3
/
2
det
(
b
→
,
a
→
)
,
{\displaystyle ={\frac {1}{2}}{\frac {s{\sqrt {s-1}}\;t{\sqrt {t-1}}}{(1-(s-1)(t-1))^{3/2}}}\det({\vec {b}},{\vec {a}})\;,}
where
a
→
=
(
a
1
,
a
2
)
,
b
→
=
(
b
1
,
b
2
)
,
u
→
=
(
u
1
,
u
2
)
,
v
→
=
(
v
1
,
v
2
)
,
u
→
=
s
a
→
,
v
→
=
t
b
→
{\displaystyle {\vec {a}}=(a_{1},a_{2}),\;{\vec {b}}=(b_{1},b_{2}),\;{\vec {u}}=(u_{1},u_{2}),{\vec {v}}=(v_{1},v_{2}),\;{\vec {u}}=s{\vec {a}},\;{\vec {v}}=t{\vec {b}}}
extrema of function
G
(
s
,
t
)
=
s
2
(
s
−
1
)
t
2
(
t
−
1
)
(
1
−
(
s
−
1
)
(
t
−
1
)
)
3
{\displaystyle G(s,t)={\tfrac {s^{2}(s-1)\;t^{2}(t-1)}{(1-(s-1)(t-1))^{3}}}}
G
s
=
0
→
3
s
−
2
+
2
(
s
−
1
)
(
t
−
1
)
=
0
.
{\displaystyle G_{s}=0\ \rightarrow \ 3s-2+2(s-1)(t-1)=0\;.}
Because
G
(
s
,
t
)
=
G
(
t
,
s
)
{\displaystyle G(s,t)=G(t,s)}
s and t :
G
t
=
0
→
3
t
−
2
+
2
(
s
−
1
)
(
t
−
1
)
=
0
.
{\displaystyle G_{t}=0\ \rightarrow \ 3t-2+2(s-1)(t-1)=0\;.}
Solving both equations for s and t yields
s
=
t
=
1
2
,
{\displaystyle s=t={\frac {1}{2}}\;,\quad }
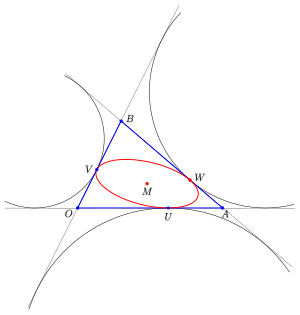
Three mutually touching inellipses of a triangle
^ Imre Juhász: Control point based representation of inellipses of triangles , Annales Mathematicae et Informaticae
40 (2012) pp. 37–46, p.44




































![{\displaystyle A=[a,0],B=[0,b]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e20a3ed09ce0085040d3b2cc37c6303cc137539e)



![{\displaystyle W=[{\tfrac {a}{2}},{\tfrac {b}{2}}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9b2fe07d55a71a4127507e0c14cfa001b1f1824d)

![{\displaystyle [x_{1},x_{2},x_{3}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/51a96ce7336b520a13d666da01d29a958ba2ab0c)

![{\displaystyle [\xi ,\eta ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/03bdcaa9622ae82e479c0b910f97e3d0e992fa5d)
![{\displaystyle [\xi ,\eta ,1]^{T}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8e0c3209483f658ed3d8d2c2af76cceb3850cdf5)
![{\displaystyle [\cdots ,\cdots ,0]^{T}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5a472efb9e56c1741ebfa49b91fa776aa91e4b2a)
![{\displaystyle U:\ [1,0,0]^{T}\ \rightarrow \ (u_{1},u_{2})\ ,\quad V:\ [0,1,0]^{T}\ \rightarrow \ (v_{1},v_{2})\ ,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6c497b1f88e79030ca93f2a180c0684334572003)
![{\displaystyle O:\ [0,0]\ \rightarrow \ (0,0)\ ,\quad A:\ [a,0]\rightarrow \ (a_{1},a_{2})\ ,\quad B:\ [0,b]\rightarrow \ (b_{1},b_{2})\ ,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/25ef40c3ddad1e9bdeb9c7591474b55851361497)


![{\displaystyle [1,-1,0]^{T}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d1bcd1eb14d3670f46f18d86d73a10b44175c1e7)
![{\displaystyle [1,-1,{\color {red}0}]^{T}\ \rightarrow \ (u_{1}-v_{1},u_{2}-v_{2},{\color {red}0})^{T}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5de3b0fd1b4aad1b8dbdb47b38fae93679184665)
![{\displaystyle D_{i}:\left[{\frac {\pm {\sqrt {ab}}}{2}},{\frac {\pm {\sqrt {ab}}}{2}}\right]\ \rightarrow \ {\frac {1}{2}}{\frac {\pm {\sqrt {ab}}}{1\pm {\sqrt {ab}}}}\;(u_{1}+v_{1},u_{2}+v_{2}),\;}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7052800026aaac4c4ae076e61cd2a1cc4afa2adc)



![{\displaystyle \ M:\;\left[{\frac {-ab}{2}},{\frac {-ab}{2}}\right]\;.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4ae4ee71147e7a2b13b7d335cf7bcb81c3cef83a)


![{\displaystyle E_{i}:\left[{\tfrac {-ab\pm {\sqrt {ab(ab-1)}}}{2}},{\tfrac {-ab\mp {\sqrt {ab(ab-1)}}}{2}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a770d90aaa18c9e0928f1342be65ab53c1df35ec)


![{\displaystyle {\begin{aligned}{\vec {f}}_{1}&={\vec {MD_{1}}}={\frac {1}{2}}{\frac {\sqrt {ab}}{ab-1}}\;(u_{1}+v_{1},u_{2}+v_{2})\\[6pt]{\vec {f}}_{2}&={\vec {ME_{1}}}={\frac {1}{2}}{\sqrt {\frac {ab}{ab-1}}}\;(u_{1}-v_{1},u_{2}-v_{2})\;\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/051c4b96e2a66a70990e8af3c4faad92bcb65026)


![{\displaystyle W:\left[{\frac {a}{2}},{\frac {b}{2}}\right]\ \rightarrow \ \left({\frac {u_{1}a+v_{1}b}{a+b+2}}\;,\;{\frac {u_{2}a+v_{2}b}{a+b+2}}\right)\;.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4c12e425cfa9038ab72d6f9ccd25447915a5aa0f)

![{\displaystyle K:\ [a,b]\ \rightarrow \ \left({\frac {u_{1}a+v_{1}b}{a+b+1}}\;,\;{\frac {u_{2}a+v_{2}b}{a+b+1}}\right)\ .}](https://wikimedia.org/api/rest_v1/media/math/render/svg/25a6a51aecf46a39ee8a67c88ebd39922423804e)

![{\displaystyle \left[\xi ,{\frac {ab}{4\xi }}\right]\ \rightarrow \ \left({\frac {4u_{1}\xi ^{2}+v_{1}ab}{4\xi ^{2}+4\xi +ab}},{\frac {4u_{2}\xi ^{2}+v_{2}ab}{4\xi ^{2}+4\xi +ab}}\right)\ ,\ -\infty <\xi <\infty \ .}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3c3a222e4fc60737d44af7ad7fd5c611518d1cec)




















