Orbit of Venus

Venus has an orbit with a semi-major axis of 0.723 au (108,200,000 km; 67,200,000 mi), and an eccentricity of 0.007.[1][2] The low eccentricity and comparatively small size of its orbit give Venus the least range in distance between perihelion and aphelion of the planets: 1.46 million km. The planet orbits the Sun once every 225 days[3] and travels 4.54 au (679,000,000 km; 422,000,000 mi) in doing so,[4] giving an average orbital speed of 35 km/s (78,000 mph).
Conjunctions and transits
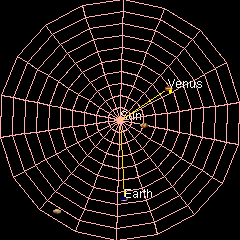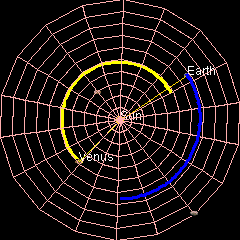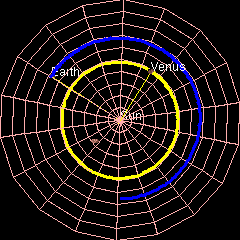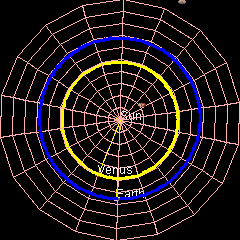
[edit]When the geocentric ecliptic longitude of Venus coincides with that of the Sun, it is in conjunction with the Sun – inferior if Venus is nearer and superior if farther. The distance between Venus and Earth varies from about 42 million km (at inferior conjunction) to about 258 million km (at superior conjunction). The average period between successive conjunctions of one type is 584 days – one synodic period of Venus. Five synodic periods of Venus is almost exactly 13 sidereal Venus years and 8 Earth years, and consequently the longitudes and distances almost repeat.[5]
The 3.4° inclination of Venus's orbit is great enough to usually prevent the inferior planet from passing directly between the Sun and Earth at inferior conjunction. Such solar transits of Venus rarely occur, but with great predictability and interest.[6][7]
Close approaches to Earth and Mercury
[edit]In this current era, the nearest that Venus comes to Earth is just under 40 million km. Because the range of heliocentric distances is greater for the Earth than for Venus, the closest approaches come near Earth's perihelion. The Earth's declining eccentricity is increasing the minimum distances. The last time Venus drew nearer than 39.5 million km was in 1623, but that will not happen again for many millennia, and in fact after 5683 Venus will not even come closer than 40 million km for about 60,000 years. [8] The orientation of the orbits of the two planets is not favorable for minimizing the close approach distance. The longitudes of perihelion were only 29 degrees apart at J2000, so the smallest distances, which come when inferior conjunction happens near Earth's perihelion, occur when Venus is near perihelion. An example was the transit of December 6, 1882: Venus reached perihelion Jan 9, 1883, and Earth did the same on December 31. Venus was 0.7205 au from the Sun on the day of transit, decidedly less than average.[9]
Moving far backwards in time, more than 200,000 years ago Venus sometimes passed by at a distance from Earth of barely less than 38 million km, and will next do that after more than 400,000 years.
Venus and Earth come the closest, but they come less often closer than Venus and Mercury.[10] While Venus approaches Earth the closest, Mercury approaches Earth more often the closest of all planets.[11] That said, Venus and Earth still have the lowest gravitational potential difference between them than to any other planet, needing the lowest delta-v to transfer between them, than to any other planet from them.[12][13]
The distance between Venus and Mercury will become smaller over time primarily because of Mercury's increasing eccentricity.
Historical importance
[edit]The discovery of phases of Venus by Galileo in 1610 was important. It contradicted the model of Ptolemy which considered all celestial objects to revolve around the Earth and was consistent with others, such as those of Tycho and Copernicus.
In Galileo’s day the prevailing model of the universe was based on the assertion by the Greek astronomer Ptolemy almost 15 centuries earlier that all celestial objects revolve around Earth (see Ptolemaic system). Observation of the phases of Venus was inconsistent with this view but was consistent with the Polish astronomer Nicolaus Copernicus’s idea that the solar system is centered on the Sun. Galileo’s observation of the phases of Venus provided the first direct observational evidence for Copernican theory.[14]
Observations of transits of Venus across the Sun have played a major role in the history of astronomy in the determination of a more accurate value of the astronomical unit.[15]
Accuracy and predictability
[edit]Venus has a very well observed and predictable orbit. From the perspective of all but the most demanding, its orbit is simple. An equation in Astronomical Algorithms that assumes an unperturbed elliptical orbit predicts the perihelion and aphelion times with an error of a few hours.[16] Using orbital elements to calculate those distances agrees to actual averages to at least five significant figures. Formulas for computing position straight from orbital elements typically do not provide or need corrections for the effects of other planets.[17]
However, observations are much better now, and space age technology has replaced the older techniques.[18] E. Myles Standish wrote Classical ephemerides over the past centuries have been based entirely upon optical observations: almost exclusively, meridian circle transit timings. With the advent of planetary radar, spacecraft missions, VLBI, etc., the situation for the four inner planets has changed dramatically. For DE405, created in 1998, optical observations were dropped and as he wrote initial conditions for the inner four planets were adjusted to ranging data primarily... Now the orbit estimates are dominated by observations of the Venus Express spacecraft. The orbit is now known to sub-kilometer accuracy.[19]
Table of orbital parameters
[edit]No more than five significant figures are presented here, and to this level of precision the numbers match very well the VSOP87[1] elements and calculations derived from them, Standish's (of JPL) 250-year best fit,[20] Newcomb's,[2] and calculations using the actual positions of Venus over time.
| distances | au | Million km |
|---|---|---|
| semimajor axis | 0.72333 | 108.21 |
| perihelion | 0.71843 | 107.48 |
| aphelion | 0.7282 | 108.94 |
| average[21] | 0.72335 | 108.21 |
| circumference | 4.545 | 679.9 |
| closest approach to Earth | 0.2643 | 39.54 |
| eccentricity |
|---|
| 0.0068 (almost perfect circle) |
| angles | degrees |
|---|---|
| inclination to ecliptic | 3.39 |
| times | days |
|---|---|
| orbital period | 224.70 |
| synodic period | 583.92 |
| speed | km/s |
|---|---|
| average speed | 35.02 |
| maximum speed | 35.26 |
| minimum speed | 34.78 |
Dust ring
[edit]
Venus' orbital space has been shown to have its own dust ring-cloud,[22] with a suspected origin either from Venus trailing asteroids,[23] interplanetary dust migrating in waves, or the remains of the Solar System's circumstellar disc out of which its proto-planetary disc and then itself, the Solar planetary system, formed.[24]
References
[edit]- ^ a b Simon, J.L.; Bretagnon, P.; Chapront, J.; Chapront-Touzé, M.; Francou, G.; Laskar, J. (February 1994). "Numerical expressions for precession formulae and mean elements for the Moon and planets". Astronomy and Astrophysics. 282 (2): 663–683. Bibcode:1994A&A...282..663S.
- ^ a b Jean Meeus, Astronomical Formulæ for Calculators, by Jean Meeus. (Richmond, VA: Willmann-Bell, 1988) 99. Elements by Simon Newcomb
- ^ The sidereal and anomalistic years are both 224.7008 days long. The sidereal year is the time taken to revolve around the Sun relative to a fixed reference frame. More precisely, the sidereal year is one way to express the rate of change of the mean longitude at one instant, with respect to a fixed equinox. The calculation shows how long it would take for the longitude to make one revolution at the given rate. The anomalistic year is the time span between successive closest approaches to the Sun. This may be calculated in the same manner as the sidereal year, but the mean anomaly is used.
- ^ Jean Meeus, Astronomical Algorithms (Richmond, VA: Willmann-Bell, 1998) 238. The formula by Ramanujan is accurate enough.
- ^ Five synodic years is 2919.6 days. Thirteen sidereal years for Venus is 2921.1 days, and eight for Earth is 2922.05 days. The heliocentric longitude of Earth advances by 0.9856° per day, and after 2919.6 days, it has advanced by 2878°, only 2° short of eight revolutions (2880°).
- ^ Venus transit page. Archived 2015-07-01 at the Wayback Machine by Aldo Vitagliano, creator of Solex
- ^ William Sheehan, John Westfall The Transits of Venus (Prometheus Books, 2004)
- ^ close approach distances generated by Solex
- ^ screenshots from the Institut de Mécanique Céleste et de Calcul des Éphémérides (IMCCE) ephemeris generator
- ^ Cain, Fraser (2009-08-06). "Closest Planet to Venus". Universe Today. Archived from the original on 2016-06-13. Retrieved 2022-10-21.
- ^ "Venus is not Earth's closest neighbor". Physics Today. AIP Publishing. 2019-03-12. doi:10.1063/pt.6.3.20190312a. ISSN 1945-0699. S2CID 241077611.
- ^ Petropoulos, Anastassios E.; Longuski, James M.; Bonfiglio, Eugene P. (2000). "Trajectories to Jupiter via Gravity Assists from Venus, Earth, and Mars". Journal of Spacecraft and Rockets. 37 (6). American Institute of Aeronautics and Astronautics (AIAA): 776–783. doi:10.2514/2.3650. ISSN 0022-4650.
- ^ Taylor, Chris (2020-07-09). "Welcome to Cloud City: The case for going to Venus, not Mars". Mashable. Retrieved 2022-10-21.
- ^ "Venus." Encyclopædia Britannica. Encyclopædia Britannica Online. Encyclopædia Britannica Inc., 2014. Web. 05 Aug. 2014. http://www.britannica.com/EBchecked/topic/625665/Venus
- ^ see, for example William Sheehan, John Westfall The Transits of Venus (Prometheus Books, 2004) or Eli Maor, Venus in Transit (Princeton University Press, 2004)
- ^ Meeus (1998) pp 269-270
- ^ see, for example, Simon et al. (1994) p 681
- ^ "The newer and more accurate data types determine these orbits far more accurately (by orders of magnitude) than do the optical data." Standish & Williams (2012). "CHAPTER 8: Orbital Ephemerides of the Sun, Moon, and Planets" (PDF). 2012 version of the Explanatory Supplement p 10
- ^ Folkner; et al. (2008). "The Planetary and Lunar Ephemeris DE421" (PDF). JPL Interoffice Memorandum IOM 343.R-08-003. p. 1.
- ^ Standish and Williams(2012) p 27
- ^ Average distance over times. Constant term in VSOP87. It corresponds to the average taken of many short, equal time intervals.
- ^ Frazier, Sarah (2021-04-16). "NASA's Parker Solar Probe Sees Venus Orbital Dust Ring". NASA. Retrieved 2023-01-21.
- ^ Garner, Rob (2019-03-12). "What Scientists Found After Sifting Through Dust in the Solar System". NASA. Retrieved 2023-01-21.
- ^ Rehm, Jeremy (2021-04-15). "Parker Solar Probe Captures First Complete View of Venus Orbital Dust Ring". JHUAPL. Retrieved 2023-01-21.
